What is the definition of a RHOMBUS?
First, let's go over the definition of a quadrilateral- a closed polygon in the plane consisting of four line segments where the segments do not cross each other.
Rhombus- a quadrilateral whose sides all have the same length.
Recall that the definition of a square- is a quadrilateral with four right angles whose sides all have the same length.
Therefore a square is a special type of rhombus. We can use our knowledge about squares to make conjectures about rhombuses and then prove some facts about rhombuses.
Examples:

Part 1: Construction of a square and a rhombus using GSP and the definition of a square and a rhombus.
1. Click here to open a GSP sketch. You will find a segment that is 9.00cm in length. Use what you know about squares to construct a square with an area of 81cm^2.
Click here to see the final square.
2. Click here to open a GSP sketch. You will find another segment that is 9.00cm in length. Construct a "Kite" shaped rhombus, with segment AB as one side.
Review: The mathematical definition of a circle is all the points that are equal distance from the center point. Use circles with equal radii to construct a rhombus.
Click here to see the final rhombus.
Question: Explain why the quadrilateral ABCD is a rhombus.
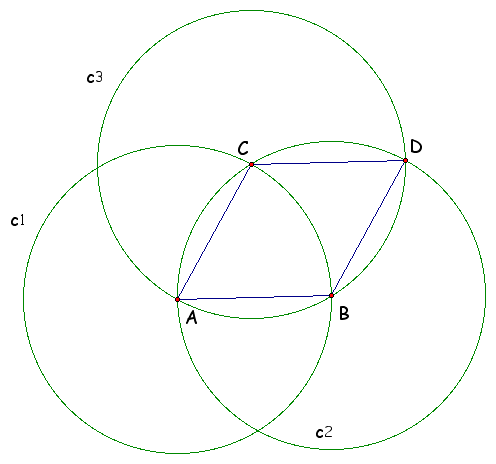
The way we constructed the rhombus implies that all the lengths are equal. Each of the sides of the rhombus are all radii of circles that were created with equal radii.
Part 2: Exloring the Diagonals of a Rhombus.
Exploration #1: Lengths of the diagonals of a rhombus vs. a square.
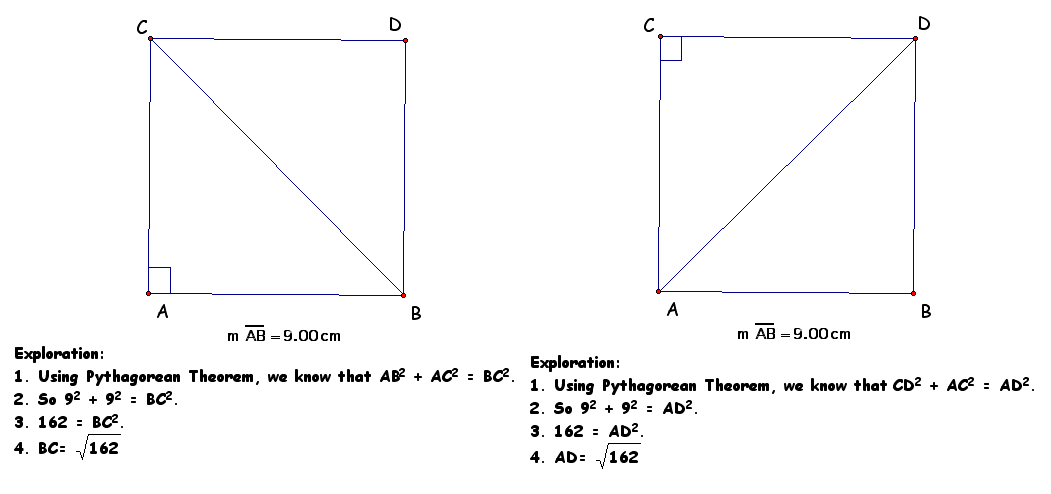
1. Click here to open a square in GSP and measure the diagonals.
Questions: What relationship did you find between the two diagonals of a square?
Answer: They have equal lengths.
Questions: Use what you know about squares to explain why the diagonals must be equal for a square?
Answer:
2. Since a square is a special type of a rhombus, should the diagonals of rhombuses be equal?
Click here to open a rhombus in GSP and measure the diagonals.
Question: Are the lengths of the two diagonals the same length for a rhombus?
After you measured the lengths of the diagonals, you would discover that they are not equal.

Exploration #2: Midpoint of the diagonals of a rhombus.
1. Now, using the same rhombus, mark the center point E, where the diagonals intersect. Now explore the diagonals.
Question: Is there a relationship between the diagonals? Explore point, E with respect to each of the diagonals.
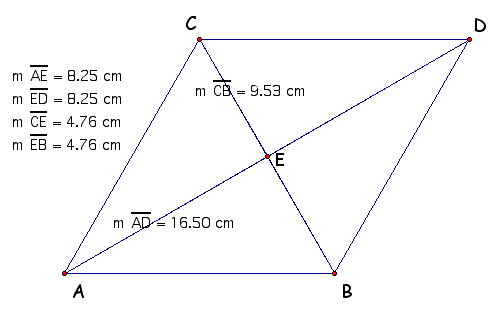
Conjecture: The intersection of the diagonals in a rhombus, is the midpoint of each of the diagonals.
2. Construct another rhombus in GSP and test our conjecture.
3. Prove our conjecture:
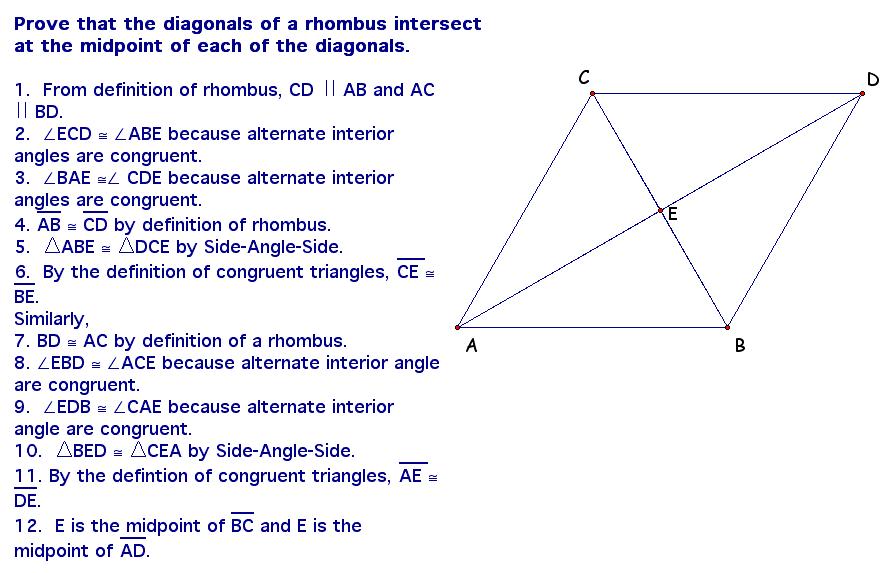
4. Should this theorem hold for squares, why or why not?
Exploration #3: Explore the intersection of the diagonals of a rhombus.
1. Recall that we have just learned that the intersection of the diagonals is the midpoint of each of the diagonals. Now let's look at the four angles that are formed at the intersection of the diagonals.
Click here to open a rhombus in GSP and measure the angles formed at the intersection.
2. Conjecture: The diagonals of a rhombus are perpendicular at the intersection.
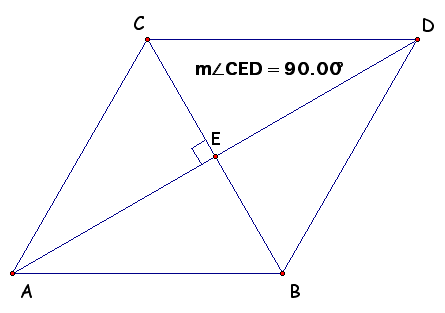
3. Prove our conjecture: Hint use congruent triangles.

Exploration #4: Explore the angles in a rhombus.
1. Open the following rhombus in GSP and measure the different angles. Do you see any relationships?

2. After measuring the different angles in the rhombus, you should have discovered that:

3. Conjecture: The diagonals bisect each of the angles of a rhombus.
4. Prove our conjecture. (Hint: use the previous proof we did in exploration #3)
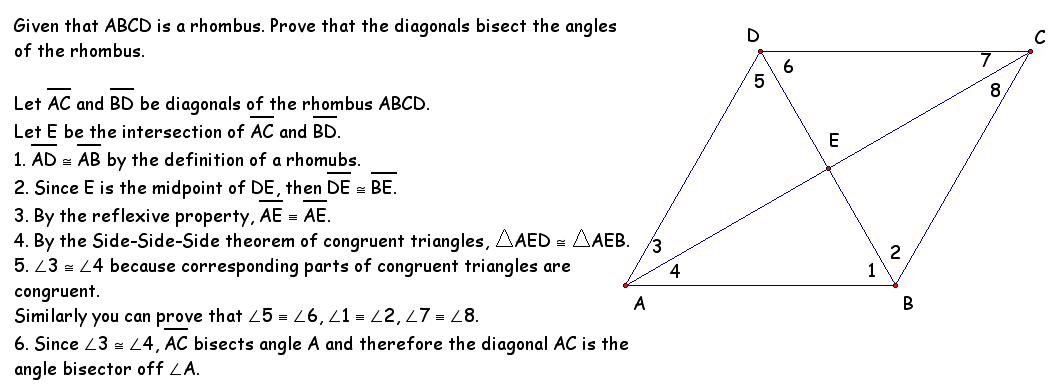
Part 3: Exloring the Area of a Rhombus.