By using Geometer's Sketchpad we can explore
the various constructions and relationships of tangent circles.
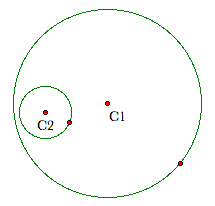
Given two circles, construct a circle that is tangent to both. Find two tangent circles that will satisfy this condition.
Step 1:
Let the smaller given circle, with center C2, rest inside of the larger circle, with center C1.


Step 2:
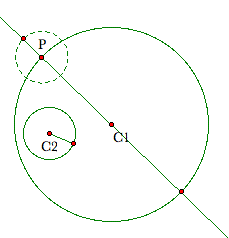
Find a point P on the larger circle and draw the diameter of the larger circle passing through this point P .


Step 3:
Using P as the center, construct a circle identical to the smaller given circle. This new circle is shown dashed. Find the two points on this new circle that pass through the line of the largest circle diameter.
Step 4:
For the first tangent circle, refer to the left image below with the orange construction lines. Connect C2 with the point on our dashed circle to form the orange line segment. Construct the midpoint of this line segment and its orange perpendicular bisector. This perpendicular bisector intersects the diameter of our original circle at point C3. Notice the isoceles triangle that has been formed. This point C3 will be the center of our tangent circle.
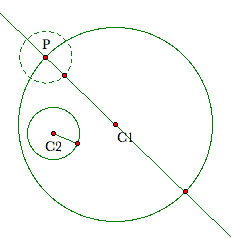
For the second tangent circle, refer to the right image below with the blue construction lines. Connect C2 with the point on our dashed circle to form the blue line segment. Construct the midpoint of this line segment and its blue perpendicular bisector. This perpendicular bisector intersects the diameter of our original circle at point C4. Notice this isoceles triangle that has been formed. This point C4 will be the center of our other tangent circle.
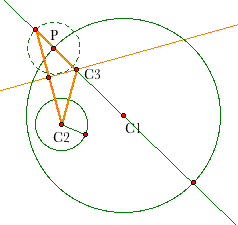
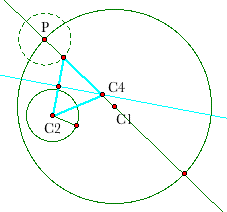
Step 5:
Using our new points, C3 and C4, construct the tangent circles. Each tangent circle will have a center of C3 or C4, and will intersect the larger given triangle at point P.
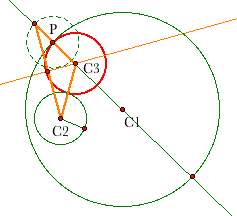
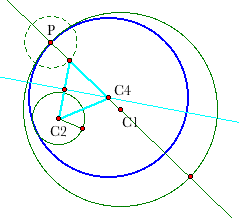
Step 6:
By removing our construction lines, we can clearly see two specific tangent circles, shown red and blue, to our two given circles.

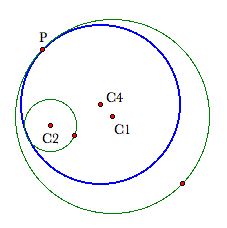
Additional Information:
View the animation of the red tangent circle as point P moves around the circumference of the larger given circle. Try experimenting with different sizes of the given green circles, and see what happens to the tangent circle.
View the animation of the blue tangent circle as point P moved around the circumference of the larger given circle. Again, try to experiment with different sizes of the given circles, and notice what happens to the tangent circle.
Geometer's Sketchpad Script Tools for your
use: Tangent 1, red tangent circle
and Tangent 2, blue tangent circle
Consider the ellipse or hyperbola generated from the locus of various centers C3 and C4, as the tangent circle moves.
We have seen in the above animations that, as our red and blue tangent circles move around the circumference of the larger circle at point P, their sizes change. We can also see that as these tangent circles move, their centers move as well. What shapes do the locus of these centers take?
Let's first trace these centers as our tangent circles move. Once point P has traveled completely around the circumference of the larger given circle, we can see the centers of our tangent circles have traced a very particular path. The resulting path in dark red looks like...
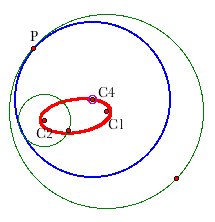
...an ELLIPSE! Each ellipse has C1 and
C2, the centers of our given circles, as foci. By definition,
an ellipse is a curve that is the locus of all points in the plane,
the sum of whose distances of k1 and k2 from the two fixed points
C1 and C2 is given by a positive constant. Let k1 be the line
segment connecting C1 and C3, and k2 be the line segment connecting
C2 and C3. View the animations below in order to see this relationship.
What happens if our given circles overlap?
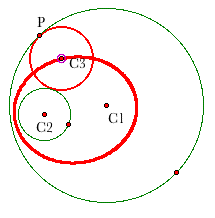
If our given circles overlap, we can follow the construction steps described above to find our two tangent circles. The resulting tangent circles will look like:


What would you expect to happen when we traced these centers C3 and C4, as point P moved? Will the centers continue to trace an ellipse? As shown in the images below, the resulting dark red paths will result in...
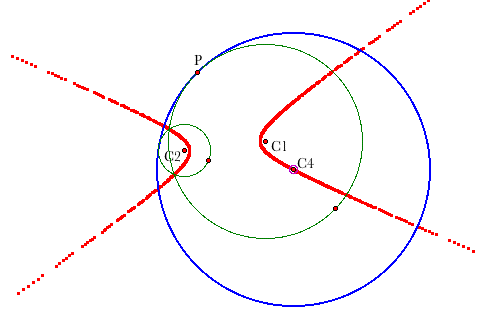
...an ELLIPSE and a HYPERBOLA!
View the animation of the red tangent circle. Notice how point C3, the center of our tangent circle, still traces the ellipse as point P moves.View the animation of the blue tangent circle. Notice how point C4, the center of our tangent circle, traces the hyperbola as point P moves.
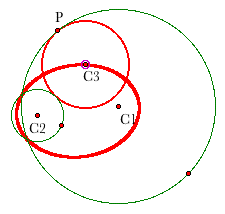
What happens if our given circles do not touch?
If our given circles do not touch, we can again follow the construction steps described above to find our two tangent circles. The resulting tangent circles will look like:
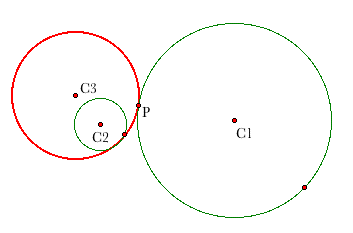

What would you expect to happen when we traced these centers C3 and C4, as point P moved? Will the centers continue to trace the locus of an ellipse, or will both result in hyperbolas? As shown in the images below, the resulting dark red paths will result in...
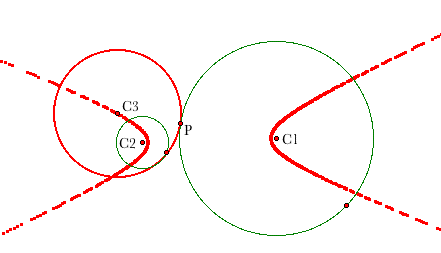
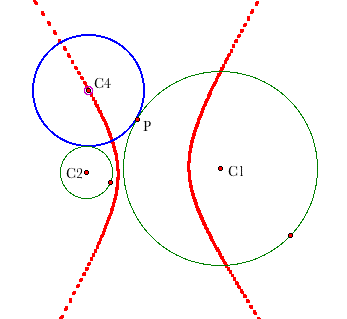
...two HYPERBOLAS!
View the animation of the red tangent circle. Notice how point C3, the center of our tangent circle, now traces the hyperbola as point P moves.