Characterization of Spherical Trigonometry

by Shawn D. Broderick

This page is about the characterization of Spherical Trigonometry that will be used for the unit. This can be thought of as the content that the teacher of the unit on spherical trigonometry should know before and they teach this unit.

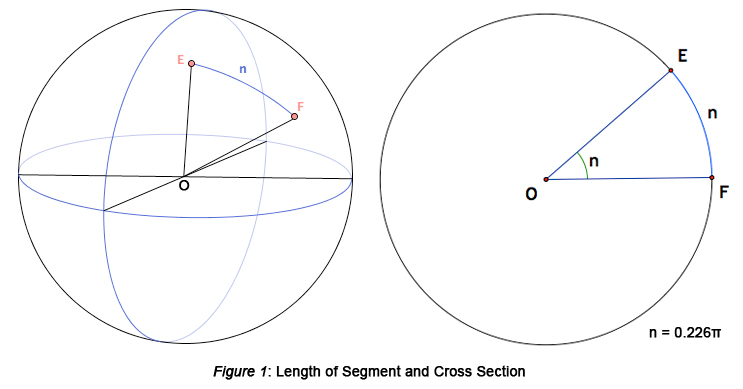
Spherical Geometry and Trigonometry is the study of geometrical and trigonometric structure and relationships on the surface of a sphere instead of the plane. The focus of this unit is on spherical trigonometry. However, as a prerequisite to the trigonometry, students spend the first day becoming familiar with spherical geometry. They explore spherical objects with the technology tool Spherical Easel. They also learn about measuring angles and segments in spherical geometry. Measuring angles is the same as in plane trigonometry. Measuring segments is usually done using radians, because the segment is thought of as an arc of the circle resulting from the cross-section of the sphere (see Figure 1).

Spherical triangles are the essence of spherical trigonometry. The characteristics of spherical triangles are a little different than planar triangles. For example, the sum of their interior angles can be greater than 180 degrees. A conventional way to label spherical triangles is shown in Figure 2:

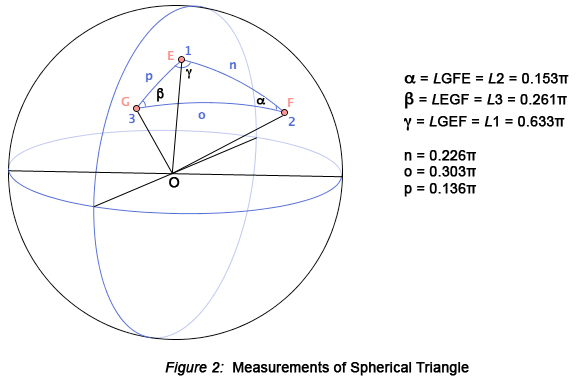
Notice that the sum of the interior angle measures in this example are: 0.153p + 0.261p + 0.633p = 1.047p. This is more than p or 180°. Spherical trigonometry also has its own laws of sines and cosines. Using the same labeling as in the above spherical triangle:

Law of Sines

Law of Cosines

One of the main reasons why we study spherical geometry and trigonometry is to navigate our way around the earth. Note the following figure:

Figure 4: from http://www.answers.com/topic/spherical-geometry

Here we see the earth and an inset showing part of the country of Japan. When we navigate our city, for example, our paths are very nearly straight, thus the rules of trigonometry follow plane trigonometry (e.g., the sum of the interior angles of a triangle is 180°). However, if we navigate around our entire hemisphere, our paths are not straight, but curved. The rules we learned in plane trigonometry do not necessarily apply (e.g., the sum of the interior angles of a spherical triangle do not have to equal 180°.) To assist in locating on and navigating the globe, a coordinate system was set up. Note the following figure:

Figure 5: from http://www.bramboroson.com/astro/jan30.html

Just like the Cartesian coordinate system, there are lines that signify zero. Because a sphere is circular, we use degrees as a unit. The earth is divided up into North, South, East, and West analogous to the divisions of Quadrants I, II, III, and IV on the Cartesian coordinate plane. Lines that go north-south are called lines of longitude or meridians. Lines that go east-west are called lines of latitude. The Prime Meridian is the line that is 0° east or west. The other significant line of longitude is the International Date Line or 180° east or west. The equator is the line that is 0° north or south. The very top point on the earth, or the North Pole, is 90° north, and the South Pole is 90° south. Notice that the figure above has given the coordinates for Miami and Rome. Naturally, you might ask, how far apart are those two cities? When flying, we want to use the shortest route to get there. We can use spherical trigonometry to determine it.