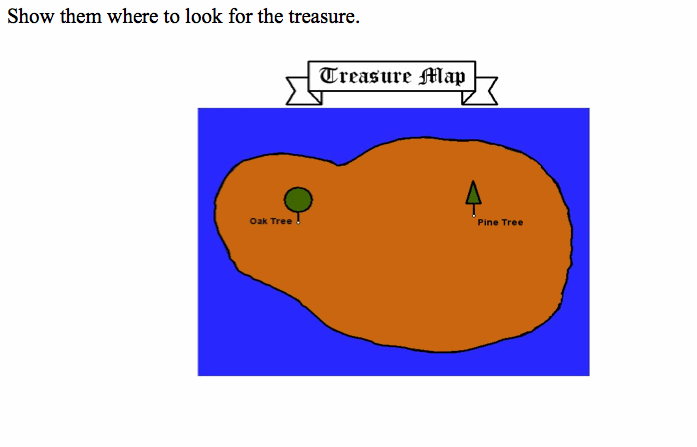
Let’s find the treasure!!

Try assuming an arbitrary location for the gallows and use GSP to construct the location of the two spikes.

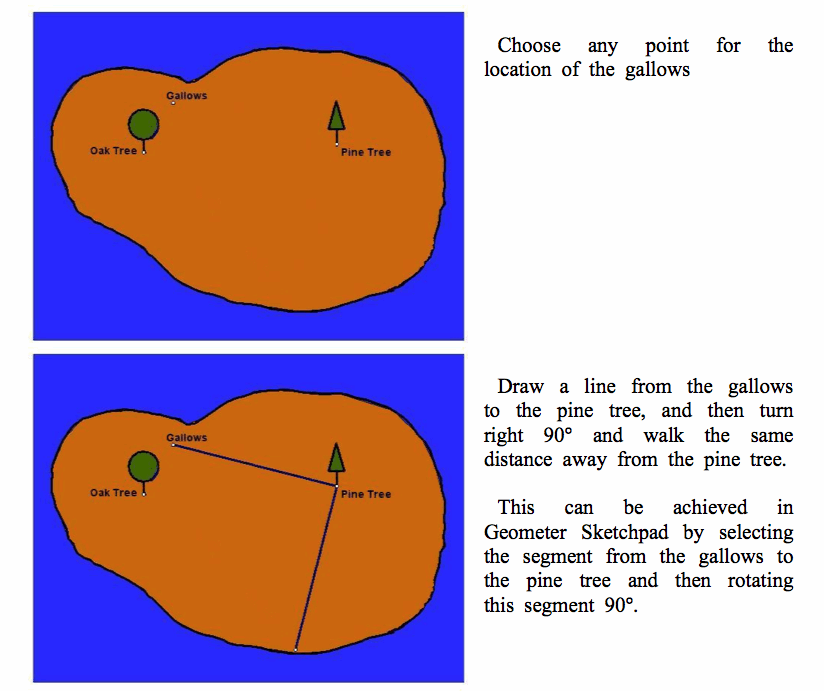
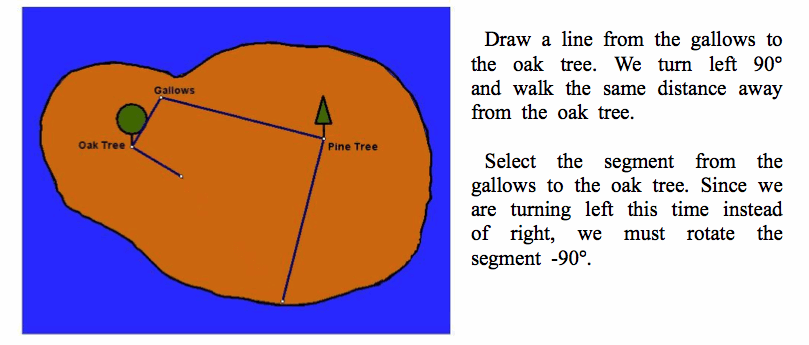
Now, move the location of the gallows.

If you want to check the location of the treasure, click here for GSP file.

What happens?

We can find that the location of the treasure is always the same regardless of the location of the gallows.

Why? Now, prove this result!!

We will use the axis of coordinates to figure out the location of the treasure. Before reflecting this situation onto the axis of coordinates, we can think of this:

For an arbitrary point for the Gallows, construct right triangles to represent the directions. Construct the line determined by the two trees and drop perpendiculars to that line from the gallows and from each of the stakes at the other acute vertices of the right triangles.

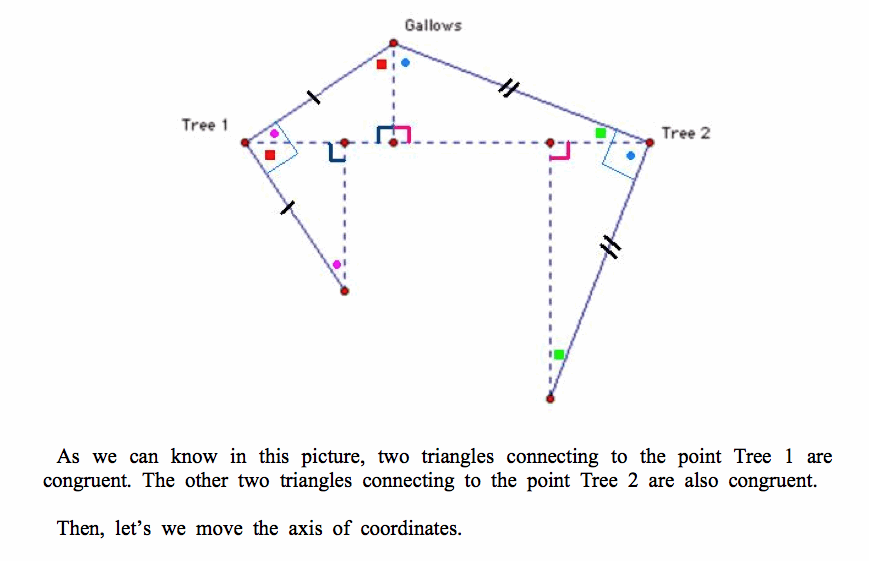
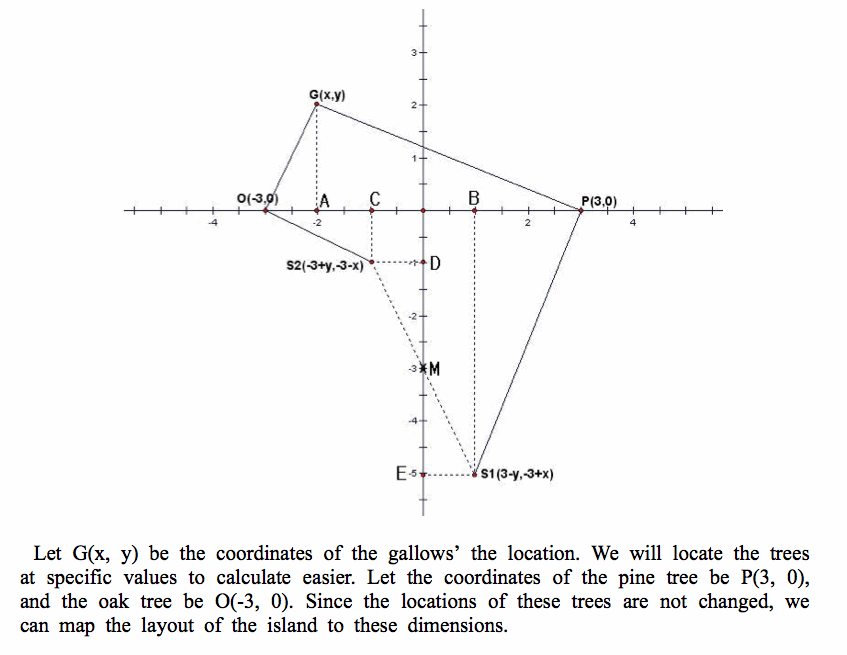
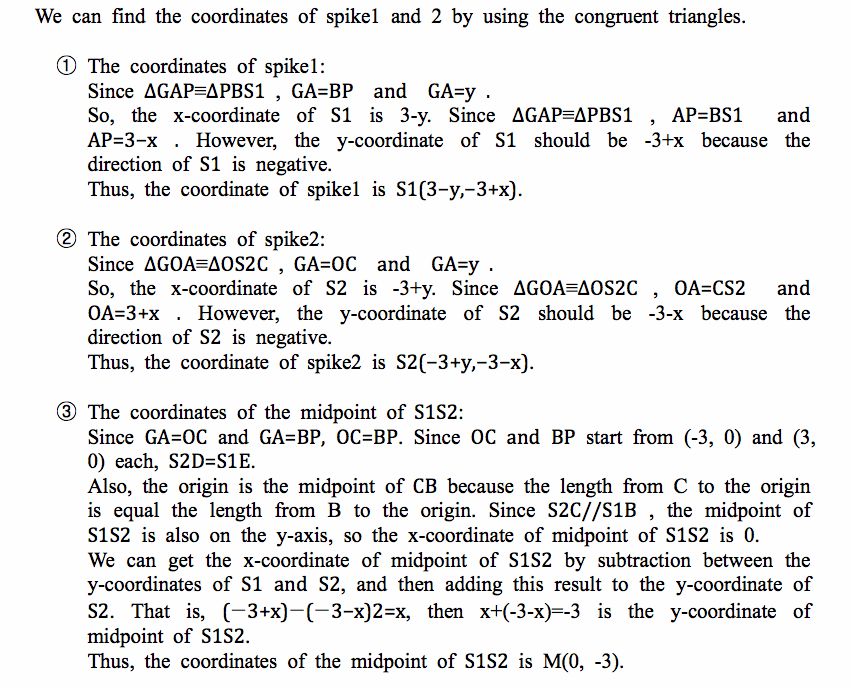
Therefore, no matter where the gallows are located, the treasure is always buried in the same location.