
Assignment
12 :: Spreadsheets
Fibonacci
Sequence
By Jamie K.
York
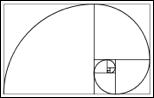
Have you ever seen a pattern
in nature? Perhaps you have seen one in a seashell or a cactus. Many of these
patterns can be described mathematically using sequences.
The Fibonacci sequence is a series of
numbers such that f(0) = 1, f(1) = 1, and f(n) = f(n-1) + f(n-2). See the full list of numbers in the
series below, up to f(30):
|
n |
f(n) |
|
0 |
1 |
|
1 |
1 |
|
2 |
2 |
|
3 |
3 |
|
4 |
5 |
|
5 |
8 |
|
6 |
13 |
|
7 |
21 |
|
8 |
34 |
|
9 |
55 |
|
10 |
89 |
|
11 |
144 |
|
12 |
233 |
|
13 |
377 |
|
14 |
610 |
|
15 |
987 |
|
16 |
1597 |
|
17 |
2584 |
|
18 |
4181 |
|
19 |
6765 |
|
20 |
10946 |
|
21 |
17711 |
|
22 |
28657 |
|
23 |
46368 |
|
24 |
75025 |
|
25 |
121393 |
|
26 |
196418 |
|
27 |
317811 |
|
28 |
514229 |
|
29 |
832040 |
|
30 |
1346269 |
In the sequence, there are
multiple patterns that exist. LetŐs explore these together.
Adjacent Terms
In a comparison of adjacent
terms in the sequence, we can see that the ratio approaches a specific value as
n increases.
|
n |
f(n) |
Ratio of Adjacent Terms |
|
0 |
1 |
1 |
|
1 |
1 |
0.50000000 |
|
2 |
2 |
0.66666667 |
|
3 |
3 |
0.60000000 |
|
4 |
5 |
0.62500000 |
|
5 |
8 |
0.61538462 |
|
6 |
13 |
0.61904762 |
|
7 |
21 |
0.61764706 |
|
8 |
34 |
0.61818182 |
|
9 |
55 |
0.61797753 |
|
10 |
89 |
0.61805556 |
|
11 |
144 |
0.61802575 |
|
12 |
233 |
0.61803714 |
|
13 |
377 |
0.61803279 |
|
14 |
610 |
0.61803445 |
|
15 |
987 |
0.61803381 |
|
16 |
1597 |
0.61803406 |
|
17 |
2584 |
0.61803396 |
|
18 |
4181 |
0.61803400 |
|
19 |
6765 |
0.61803399 |
|
20 |
10946 |
0.61803399 |
|
21 |
17711 |
0.61803399 |
|
22 |
28657 |
0.61803399 |
|
23 |
46368 |
0.61803399 |
|
24 |
75025 |
0.61803399 |
|
25 |
121393 |
0.61803399 |
|
26 |
196418 |
0.61803399 |
|
27 |
317811 |
0.61803399 |
|
28 |
514229 |
0.61803399 |
|
29 |
832040 |
0.61803399 |
|
30 |
1346269 |
|
Every Second Term
Next we can look at the ratio
of every second term. Similarly to the ratio of adjacent terms, we see that
this ratio approaches a new value as n increases.
|
n |
f(n) |
Ratio of Every Second Term |
|
0 |
1 |
0.5 |
|
1 |
1 |
0.333333333 |
|
2 |
2 |
0.4 |
|
3 |
3 |
0.375 |
|
4 |
5 |
0.384615385 |
|
5 |
8 |
0.380952381 |
|
6 |
13 |
0.382352941 |
|
7 |
21 |
0.381818182 |
|
8 |
34 |
0.382022472 |
|
9 |
55 |
0.381944444 |
|
10 |
89 |
0.381974249 |
|
11 |
144 |
0.381962865 |
|
12 |
233 |
0.381967213 |
|
13 |
377 |
0.381965552 |
|
14 |
610 |
0.381966187 |
|
15 |
987 |
0.381965944 |
|
16 |
1597 |
0.381966037 |
|
17 |
2584 |
0.381966001 |
|
18 |
4181 |
0.381966015 |
|
19 |
6765 |
0.38196601 |
|
20 |
10946 |
0.381966012 |
|
21 |
17711 |
0.381966011 |
|
22 |
28657 |
0.381966011 |
|
23 |
46368 |
0.381966011 |
|
24 |
75025 |
0.381966011 |
|
25 |
121393 |
0.381966011 |
|
26 |
196418 |
0.381966011 |
|
27 |
317811 |
0.381966011 |
|
28 |
514229 |
0.381966011 |
|
29 |
832040 |
|
|
30 |
1346269 |
|
Sequence Modifications
The Fibonacci sequence above
is based on the fact that f(0) = 1 and f(1) = 1. What would happen to the
pattern if these constants were altered? LetŐs consider other integers greater
than 1.
If f(0) = 1 and f(1) = 3,
this reflects a Lucas
Sequence. However, with further analysis of the same two ratios, you will
find that they approach the same values. The ratio of adjacent terms approaches
0.61803399 as n increases, and the ratio of every second term approaches
0.381966011.
|
n |
f(n) |
Ratio of Adjacent Terms |
Ratio of Every Second Term |
|
0 |
1 |
0.333333333 |
0.25 |
|
1 |
3 |
0.75000000 |
0.428571429 |
|
2 |
4 |
0.57142857 |
0.363636364 |
|
3 |
7 |
0.63636364 |
0.388888889 |
|
4 |
11 |
0.61111111 |
0.379310345 |
|
5 |
18 |
0.62068966 |
0.382978723 |
|
6 |
29 |
0.61702128 |
0.381578947 |
|
7 |
47 |
0.61842105 |
0.382113821 |
|
8 |
76 |
0.61788618 |
0.381909548 |
|
9 |
123 |
0.61809045 |
0.381987578 |
|
10 |
199 |
0.61801242 |
0.381957774 |
|
11 |
322 |
0.61804223 |
0.381969158 |
|
12 |
521 |
0.61803084 |
0.381964809 |
|
13 |
843 |
0.61803519 |
0.38196647 |
|
14 |
1364 |
0.61803353 |
0.381965836 |
|
15 |
2207 |
0.61803416 |
0.381966078 |
|
16 |
3571 |
0.61803392 |
0.381965986 |
|
17 |
5778 |
0.61803401 |
0.381966021 |
|
18 |
9349 |
0.61803398 |
0.381966008 |
|
19 |
15127 |
0.61803399 |
0.381966013 |
|
20 |
24476 |
0.61803399 |
0.381966011 |
|
21 |
39603 |
0.61803399 |
0.381966011 |
|
22 |
64079 |
0.61803399 |
0.381966011 |
|
23 |
103682 |
0.61803399 |
0.381966011 |
|
24 |
167761 |
0.61803399 |
0.381966011 |
|
25 |
271443 |
0.61803399 |
0.381966011 |
|
26 |
439204 |
0.61803399 |
0.381966011 |
|
27 |
710647 |
0.61803399 |
0.381966011 |
|
28 |
1149851 |
0.61803399 |
0.381966011 |
|
29 |
1860498 |
0.61803399 |
|
|
30 |
3010349 |
|
|
In further investigation, we
can explore other modifications to the value of f(1) to find that these two
ratios still remain unchanged.
f(1) = 4
|
n |
f(n) |
Ratio of Adjacent Terms |
Ratio of Every Second Term |
|
0 |
1 |
0.25 |
0.2 |
|
1 |
4 |
0.80000000 |
0.444444444 |
|
2 |
5 |
0.55555556 |
0.357142857 |
|
3 |
9 |
0.64285714 |
0.391304348 |
|
4 |
14 |
0.60869565 |
0.378378378 |
|
5 |
23 |
0.62162162 |
0.383333333 |
|
6 |
37 |
0.61666667 |
0.381443299 |
|
7 |
60 |
0.61855670 |
0.382165605 |
|
8 |
97 |
0.61783439 |
0.381889764 |
|
9 |
157 |
0.61811024 |
0.381995134 |
|
10 |
254 |
0.61800487 |
0.381954887 |
|
11 |
411 |
0.61804511 |
0.38197026 |
|
12 |
665 |
0.61802974 |
0.381964388 |
|
13 |
1076 |
0.61803561 |
0.381966631 |
|
14 |
1741 |
0.61803337 |
0.381965774 |
|
15 |
2817 |
0.61803423 |
0.381966102 |
|
16 |
4558 |
0.61803390 |
0.381965977 |
|
17 |
7375 |
0.61803402 |
0.381966024 |
|
18 |
11933 |
0.61803398 |
0.381966006 |
|
19 |
19308 |
0.61803399 |
0.381966013 |
|
20 |
31241 |
0.61803399 |
0.381966011 |
|
21 |
50549 |
0.61803399 |
0.381966012 |
|
22 |
81790 |
0.61803399 |
0.381966011 |
|
23 |
132339 |
0.61803399 |
0.381966011 |
|
24 |
214129 |
0.61803399 |
0.381966011 |
|
25 |
346468 |
0.61803399 |
0.381966011 |
|
26 |
560597 |
0.61803399 |
0.381966011 |
|
27 |
907065 |
0.61803399 |
0.381966011 |
|
28 |
1467662 |
0.61803399 |
0.381966011 |
|
29 |
2374727 |
0.61803399 |
|
|
30 |
3842389 |
|
|
f(1) = 10
|
n |
f(n) |
Ratio of Adjacent Terms |
Ratio of Every Second Term |
|
0 |
1 |
0.1 |
0.090909091 |
|
1 |
10 |
0.90909091 |
0.476190476 |
|
2 |
11 |
0.52380952 |
0.34375 |
|
3 |
21 |
0.65625000 |
0.396226415 |
|
4 |
32 |
0.60377358 |
0.376470588 |
|
5 |
53 |
0.62352941 |
0.384057971 |
|
6 |
85 |
0.61594203 |
0.381165919 |
|
7 |
138 |
0.61883408 |
0.382271468 |
|
8 |
223 |
0.61772853 |
0.381849315 |
|
9 |
361 |
0.61815068 |
0.382010582 |
|
10 |
584 |
0.61798942 |
0.381948986 |
|
11 |
945 |
0.61805101 |
0.381972514 |
|
12 |
1529 |
0.61802749 |
0.381963527 |
|
13 |
2474 |
0.61803647 |
0.38196696 |
|
14 |
4003 |
0.61803304 |
0.381965649 |
|
15 |
6477 |
0.61803435 |
0.38196615 |
|
16 |
10480 |
0.61803385 |
0.381965958 |
|
17 |
16957 |
0.61803404 |
0.381966031 |
|
18 |
27437 |
0.61803397 |
0.381966004 |
|
19 |
44394 |
0.61803400 |
0.381966014 |
|
20 |
71831 |
0.61803399 |
0.38196601 |
|
21 |
116225 |
0.61803399 |
0.381966012 |
|
22 |
188056 |
0.61803399 |
0.381966011 |
|
23 |
304281 |
0.61803399 |
0.381966011 |
|
24 |
492337 |
0.61803399 |
0.381966011 |
|
25 |
796618 |
0.61803399 |
0.381966011 |
|
26 |
1288955 |
0.61803399 |
0.381966011 |
|
27 |
2085573 |
0.61803399 |
0.381966011 |
|
28 |
3374528 |
0.61803399 |
0.381966011 |
|
29 |
5460101 |
0.61803399 |
|
|
30 |
8834629 |
|
|
We can also explore
modifications to the value of f(0) to find that these two ratios still remain
unchanged.
f(0) = 5
|
n |
f(n) |
Ratio of Adjacent Terms |
Ratio of Every Second Term |
|
0 |
5 |
5 |
0.833333333 |
|
1 |
1 |
0.16666667 |
0.142857143 |
|
2 |
6 |
0.85714286 |
0.461538462 |
|
3 |
7 |
0.53846154 |
0.35 |
|
4 |
13 |
0.65000000 |
0.393939394 |
|
5 |
20 |
0.60606061 |
0.377358491 |
|
6 |
33 |
0.62264151 |
0.38372093 |
|
7 |
53 |
0.61627907 |
0.381294964 |
|
8 |
86 |
0.61870504 |
0.382222222 |
|
9 |
139 |
0.61777778 |
0.381868132 |
|
10 |
225 |
0.61813187 |
0.382003396 |
|
11 |
364 |
0.61799660 |
0.381951731 |
|
12 |
589 |
0.61804827 |
0.381971466 |
|
13 |
953 |
0.61802853 |
0.381963928 |
|
14 |
1542 |
0.61803607 |
0.381966807 |
|
15 |
2495 |
0.61803319 |
0.381965707 |
|
16 |
4037 |
0.61803429 |
0.381966127 |
|
17 |
6532 |
0.61803387 |
0.381965967 |
|
18 |
10569 |
0.61803403 |
0.381966028 |
|
19 |
17101 |
0.61803397 |
0.381966005 |
|
20 |
27670 |
0.61803400 |
0.381966014 |
|
21 |
44771 |
0.61803399 |
0.38196601 |
|
22 |
72441 |
0.61803399 |
0.381966012 |
|
23 |
117212 |
0.61803399 |
0.381966011 |
|
24 |
189653 |
0.61803399 |
0.381966011 |
|
25 |
306865 |
0.61803399 |
0.381966011 |
|
26 |
496518 |
0.61803399 |
0.381966011 |
|
27 |
803383 |
0.61803399 |
0.381966011 |
|
28 |
1299901 |
0.61803399 |
0.381966011 |
|
29 |
2103284 |
0.61803399 |
|
|
30 |
3403185 |
|
|
f(0) = 10
|
n |
f(n) |
Ratio of Adjacent Terms |
Ratio of Every Second Term |
|
0 |
10 |
10 |
0.909090909 |
|
1 |
1 |
0.09090909 |
0.083333333 |
|
2 |
11 |
0.91666667 |
0.47826087 |
|
3 |
12 |
0.52173913 |
0.342857143 |
|
4 |
23 |
0.65714286 |
0.396551724 |
|
5 |
35 |
0.60344828 |
0.376344086 |
|
6 |
58 |
0.62365591 |
0.38410596 |
|
7 |
93 |
0.61589404 |
0.381147541 |
|
8 |
151 |
0.61885246 |
0.382278481 |
|
9 |
244 |
0.61772152 |
0.381846635 |
|
10 |
395 |
0.61815336 |
0.382011605 |
|
11 |
639 |
0.61798839 |
0.381948595 |
|
12 |
1034 |
0.61805140 |
0.381972663 |
|
13 |
1673 |
0.61802734 |
0.38196347 |
|
14 |
2707 |
0.61803653 |
0.381966982 |
|
15 |
4380 |
0.61803302 |
0.381965641 |
|
16 |
7087 |
0.61803436 |
0.381966153 |
|
17 |
11467 |
0.61803385 |
0.381965957 |
|
18 |
18554 |
0.61803404 |
0.381966032 |
|
19 |
30021 |
0.61803397 |
0.381966003 |
|
20 |
48575 |
0.61803400 |
0.381966014 |
|
21 |
78596 |
0.61803399 |
0.38196601 |
|
22 |
127171 |
0.61803399 |
0.381966012 |
|
23 |
205767 |
0.61803399 |
0.381966011 |
|
24 |
332938 |
0.61803399 |
0.381966011 |
|
25 |
538705 |
0.61803399 |
0.381966011 |
|
26 |
871643 |
0.61803399 |
0.381966011 |
|
27 |
1410348 |
0.61803399 |
0.381966011 |
|
28 |
2281991 |
0.61803399 |
0.381966011 |
|
29 |
3692339 |
0.61803399 |
|
|
30 |
5974330 |
|
|
Now, we can explore the
modification of both variables to see that the ratios still remain constant.
f(0) = 5, f(1) = 5
|
n |
f(n) |
Ratio of Adjacent Terms |
Ratio of Every Second Term |
|
0 |
5 |
1 |
0.5 |
|
1 |
5 |
0.50000000 |
0.333333333 |
|
2 |
10 |
0.66666667 |
0.4 |
|
3 |
15 |
0.60000000 |
0.375 |
|
4 |
25 |
0.62500000 |
0.384615385 |
|
5 |
40 |
0.61538462 |
0.380952381 |
|
6 |
65 |
0.61904762 |
0.382352941 |
|
7 |
105 |
0.61764706 |
0.381818182 |
|
8 |
170 |
0.61818182 |
0.382022472 |
|
9 |
275 |
0.61797753 |
0.381944444 |
|
10 |
445 |
0.61805556 |
0.381974249 |
|
11 |
720 |
0.61802575 |
0.381962865 |
|
12 |
1165 |
0.61803714 |
0.381967213 |
|
13 |
1885 |
0.61803279 |
0.381965552 |
|
14 |
3050 |
0.61803445 |
0.381966187 |
|
15 |
4935 |
0.61803381 |
0.381965944 |
|
16 |
7985 |
0.61803406 |
0.381966037 |
|
17 |
12920 |
0.61803396 |
0.381966001 |
|
18 |
20905 |
0.61803400 |
0.381966015 |
|
19 |
33825 |
0.61803399 |
0.38196601 |
|
20 |
54730 |
0.61803399 |
0.381966012 |
|
21 |
88555 |
0.61803399 |
0.381966011 |
|
22 |
143285 |
0.61803399 |
0.381966011 |
|
23 |
231840 |
0.61803399 |
0.381966011 |
|
24 |
375125 |
0.61803399 |
0.381966011 |
|
25 |
606965 |
0.61803399 |
0.381966011 |
|
26 |
982090 |
0.61803399 |
0.381966011 |
|
27 |
1589055 |
0.61803399 |
0.381966011 |
|
28 |
2571145 |
0.61803399 |
0.381966011 |
|
29 |
4160200 |
0.61803399 |
|
|
30 |
6731345 |
|
|
Further Exploration
How would the pattern change
if f(0) and f(1) were changed to non-integer values?
How would the pattern change
if f(0) and f(1) were changed to irrational values?
Home | EMAT 6680 | Dr. Jim Wilson