In previous investigations we looked at how to find the orthocenter by constructing the lines that goes through each vertex on a triangle and are perpendicular to the opposite sides. However, we didn't look at any of the characteristics of the orthocenter or what can be said about the triangle when the orthocenter is constructed. That is the point of this assignment. We are going to explore a very interesting relationship between some of the segments that lie on the lines that define the orthocenter. This understanding will open doors to countless other problems that can be explored, all dealing with the orthocenter of a triangle.

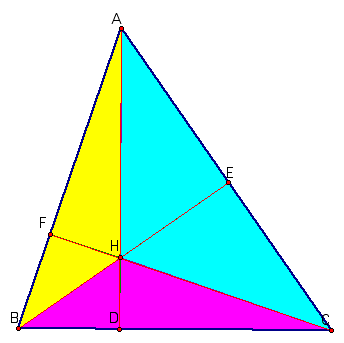
To begin this project refresh your memory on how to construct the orthocenter of a triangle by going here and following the directions that are in the introduction. Play with different types of triangles to see where the orthocenter lies on the plane (acute, obtuse, isosceles). When you construct the orthocenter your picture should look something like this

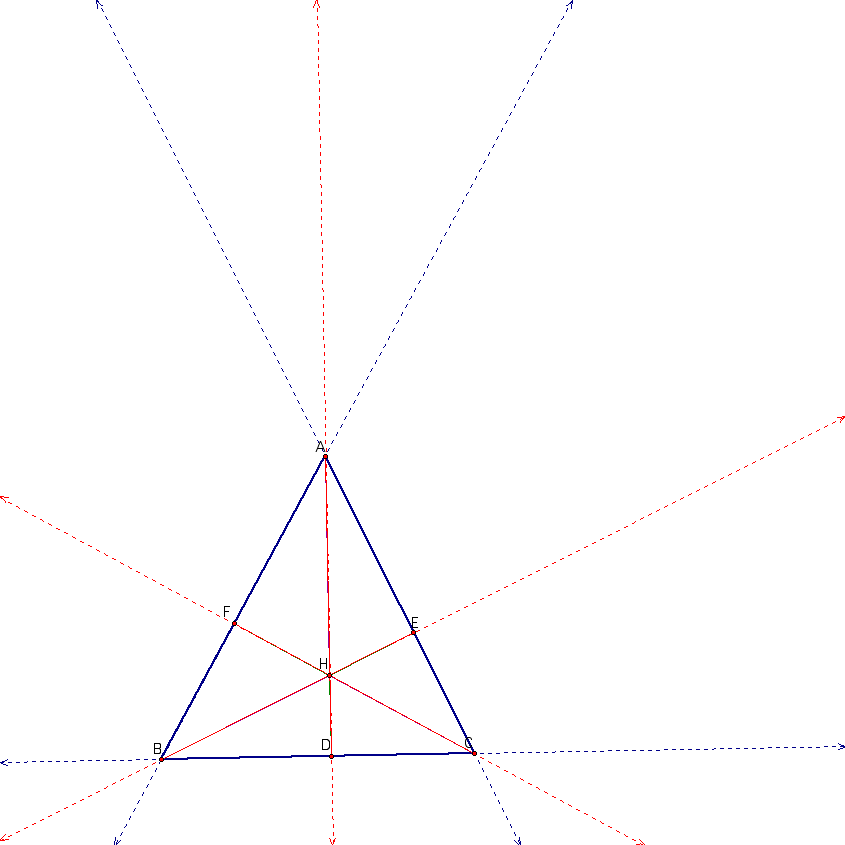
Notice how the triangle is constructed with lines instead of with segments. This is important because the perpendicular line does not always intersect a segment that defines the triangle, but we still want to able to define the orthocenter in such cases.

If you indeed went to the Geometry Sketchpad link, as was suggested, you may have noticed a series of ratios being added at the top of the page. If you didn't go to the link before please go here now. Move the vertices around. When is the sum constant? When does it start to change? When the sums are staying constant we get that the relationships

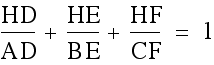
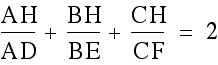
appear to be true. However, there is no proof by GSP so let's try find out exactly why these relationships are showing up.

After playing with the vertices you might have noticed that the relationships in which we are interested do not hold when we are dealing with obtuse triangles. Why will be discussed later. That means that we should look at an acute triangle when triangle to prove our conjecture.

When trying to prove something you should always start with something that you know and then try to work with that to get to your goal. The first thing we might notice that we know is that for each altitude the vertex, orthocenter and foot (where it intersects the segment) are colinear. That means the distance from the vertex to the orthocenter plus the distance from the orthocenter to the foot equals the length of the altitude. That gives us a series of relationships.

Now let's divide each side by the length of that altitude. This seems like a good idea because in our desired sums the denominators are all altitude lengths. When we do that we get

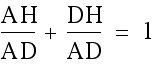
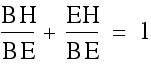
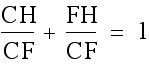
Now add all three of the equations up to get

which is the same as

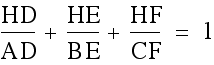
the second one will fall naturally out of the equation we developed.

Notice that the segments that we care about are altitudes. What else are altitudes good for? Computing area! So let's do so. We can compute the area of our triangle in a variety of different ways. First we will do it from the three altitudes that are drawn in.

Notice that in our desired equation we have altitudes in the denominators. If we change the denominators so that they all represent area of the large triangle we will have in a sense a common denominator since they will all have equal value even though their letters may look different.

Let's look at those pieces on the top. They each represent areas of smaller triangle embedded into our large triangle (why?).

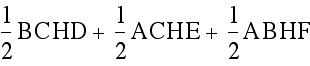=AREA

then their ratio is one. That gives us

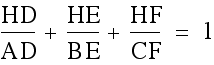
as desired. Now by simple subtraction from

We get that

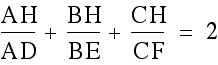
and we are done proving all that we wanted to prove.

An interesting thing happened when our triangle became obtuse, the sums ceased to be constant. Why is that? First of all the statements with which we started are no longer true when the triangle becomes obtuse

Go back to the GSP link to convince yourself of this. The other thing that goes wrong is that we can longer represent the area of the large triangle by adding those smaller triangles up. This is because the orthocenter now lives outside of the triangle and so triangles that have the orthocenter as a vertex do not live inside the triangle. Again, look at the picture and move vertices around to convince yourself. Do interesting ratios and sums like this exist for other centers of the triangle? Can you find other relationships using the orthocenter? What happens when you draw a triangle based on the feet of the altitudes? Explore and learn. By now you should be believing that triangles are rich with mathematical possibilities that are nearly infinite.

Return