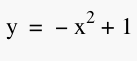---

#1) In this exercise we are going to look at the following quadratic equation

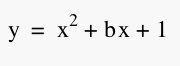
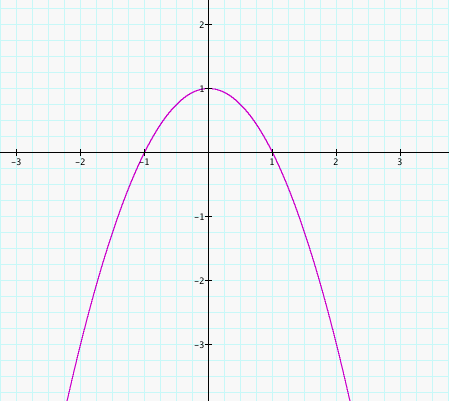
We are going to start the investigation by setting b = -3,-2,-1,0,1,2,3, and overlaying the graphs so the following picture is obtained.

As you can see the parabola always passes through the same point on the y-axis which in this case is (0,1). As you can see four different things happen with the roots:

* For b < -2 the parabola will intersect the x-axis in two points with positive x values (i.e. the original equation will have two real roots, both positive).

* For b = -2, the parabola is tangent to the x-axis and so the original equation has one real and positive root at the point of tangency.

* For -2 < b < 2, the parabola does not intersect the x-axis -- the original equation has no real roots.

* For b = 2 the parabola is tangent to the x-axis (one real negative root) and for b > 2, the parabola intersets the x-axis twice to show two negative real roots for each b.

If you were to change the equation so that two was added as the constant at the end of the equation then all the parabolas would pass through the y-axis at two. Let's take a look at what happens when the constant changes.

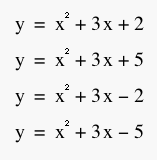
By varying the constant term the parabola moves up and down the y-axis. This also plays a role in the determination of the number of real roots.

Now take a look at the locus of points that form a parabolic shape.

 
The locus of the vertices for the parabolas create a parabola with the vertex at (0,1) and the direction facing downward, thus the following equation is formed: