The M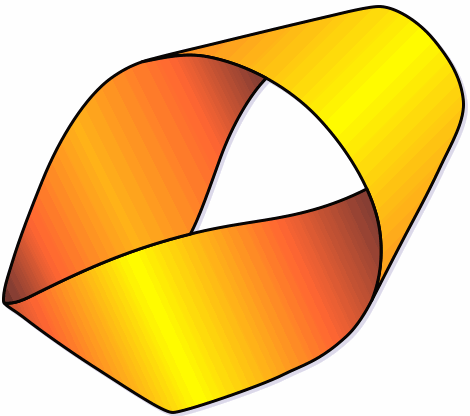
bius Strip
picture taken from http://www.puzzles.com/puzzlesineducation/HandsOnPuzzles/MobiusStrip.html
by
Amanda Newton
The Mbius strip is a puzzling representation of a mathematical object. It is formed by rotating a rectangular strip 180 degrees and joining it with the other end. The strip appears to be two-sided because of its "bend," but at a closer look, one can see it is actually one-sided. The strip is named after August Mobius. Mobius was a mathematician and astronomer of the 19th century. The Mbius strip is often used as an optical illusion and appears in the work of the famous artist M.C. Escher. Escher's work uses optical illusions quite frequently and he is most known for "Waterfall" and "Ascending and Descending." He drew what many called "impossible" constructions. Among these illusions was the mbius strip, and more specifically "Parade of Ants" as seen below where ants are drawn walking across a mbious strip. The mbius strip shows up in various other artworks such as the sculpture "Endless Ribbon," by Max Bill as well as short stories like "A Subway Named Möbius", by A.J. Deutsch.
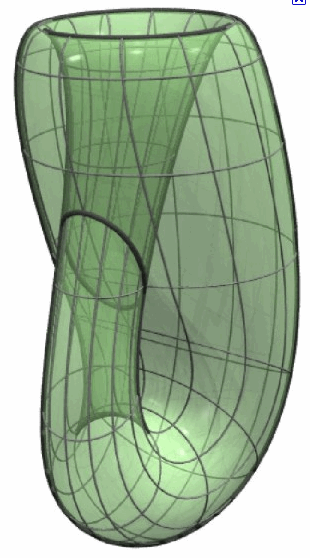
A popular geometric object using the topology of the mbious strip is called the Klein bottle (shown below). These are sold in many different sizes and make for popular gift ideas, especially for those that enjoy the confusion brought about by a good illusion. Mbious scarfs are sold as well which are one-sided scarfs with a twist in them.
Taken From: google.com/imgres...iowahawk....
Some say that the mbius strip was once used as a conveyor belt so that both sides wore out evenly. Though this idea seems to make perfect sense, I could find no proof of this. But this mathematical puzzlement is most interesting when you are able to see and touch it for yourself, rather than just hypothesis about its greatness. So let's make a mbius strip!
Begin with a rectangular strip. Mine has one yellow side and one red side. (This is only for effect. Use a strip where the front and back colors are the same.)
Fold one end 180 degrees and join the opposite side. So for me, I will turn the paper, yellow side up, 180 degrees and align the yellow ends facing together. Now join the two by tape or staple. What you are left with should have 1 "twist" in it. You have made a mbius strip!
Do you see how the two colors are almost combined together? Are there still two sides on the figure? NO! If you are not convinced by the two colors in the picture and want to see it for yourself using a uni-colored strip, draw a line across the surface (using a pen or pencil) until you end up where you started.
A strip where both ends were the same color would look like the one below:
Now take a look at the strip - what do you notice? Both "sides" have the line - this is because there are not two sides; there is only one side. Unfortunately, many leave the discussion at this point. However, there are endless extensions to the puzzle of the mbius strip. Let's explore a few.
First, cut along this line drawn on the strip. What do you predict will happen? My original prediction: the strip will be cut into two congruent smaller mbius strips.
Surprisingly, we now have one strip with 2 twists. Is this still a mbius strip? In other words, is it still 1 sided? Let's do the pen test again. The result is shown below.
The pen is not along the entire surface. Ergo, the figure is 2-sided and not a mbius strip. So that we are not wasting, let's use the same strip and cut along the line made by the pen again. The outcome is shown below.
At first glance, the figure looks like a mess of knots, but upon further inspection, it is 2 intertwined strips with 2 twists each. Our conclusion is that when we cut through the middle of a one-sided strip (with 2 twists), we are left with 2 equivalent pieces that are looped together. What would happen if we cut each one in half again? My hypothesis is that since we already know cutting a 2-sided strip in half yields 2 congruent strips, we should get 4 small strips with 2 twists each. But will they all be looped together? Or will it be a string of loops? See for yourself!
Now let's look at something a little different. What if we cut the strip by a third? You're going to need a new mbius strip. Once you've constructed a new mbius strip, make a hash mark for your starting point at about a third of the strip (this does not have to be exact, but make sure to stay straight along this path while cutting). What predictions do you make? Will it have 3 twists now? The interesting result is shown below.
What do you predict to happen if we start at one of these hash marks and cut all the way around? Will it have 3 twists now? The interesting result is shown below.
The strip was cut into 2 strips looped together. However, this time, the two strips made are not similar in length or twists. One of the strips is a small mbius strip (one sided), the other is a long two-sided strip. Why is one longer than the other? One question that comes to mind, is what is the difference in length between the two? Maybe there is no correlation between the two strips' lengths, but it is always interesting to check a relationship. What prediction do you make about their lengths? To compare the two, I will cut the strips and lay them out flat, so they are simply 2 rectangular strips as shown below.
It looks as though the longer one may be twice the length of the shorter strip. Let's measure to be sure.
The longer strip is about 12 inches and the shorter is about 6 inches! The shorter strip is half the length of the longer strip!
What would happen if we cut a mbius strip into fourths? Fifths? Will we see a pattern? What if we took a loop with 2 twists and cut it into thirds? What if we made a strip with 3 twists? Would it be 1 sided? What would happen if we cut the strip with 3 twists in half. There are numerous explorations with mbius strips - the possibilities are endless. The best part is that there are explorations and activities that can be done with mbius strips aside from taking one strip and cutting it a certain amount of times.
I want to do an activity that combines love and mathematics (not that they are not already perfectly intertwined together). I have explored the heart shape and how it relates to mathematics on my page - The Heart of Mathematics. At the end of the page, you will see a Valentine's day card with the heart made purely through math! Let's do something creative and mathematical that you can do without technology.
First, begin with 2 same sized rectangular strips of paper (this time, they should preferably be the same color on each side). I recommend using pink or red.
Take one piece of paper and fold it downwards and over. Then tape the ends together (forming a mobius strip).
Take the other and fold it upwards and over. Then tape the ends together. (This part is critical!) In doing this, you have created a left-handed and right-handed mbius strip.
Tape the two flat sections of the strips together at a 90 degree angle.
Here is an amazing Valentine's Day gift. I'm sure you're thinking, "What is this?! This is not a very interesting gift at all!" What you do is give this to your Valentine and tell them to make an incision on the middle of each loop, and cut along it. The process of this is shown below.
Let's see what our Valentine's will see.
We cut a mbius strip in half, similarly to how we did before. It is surprising to see that we don't have 2 strips with 2 twists as we saw in the first section of this write-up. This is without a doubt, a unique and mathematically friendly Valentine's Day gift!
To see a video demonstration similar to what was shown above see: Valentine's Day
There are plenty of interesting sites dedicated just to mbius strips. Some of my favorites that I have found are listed below.
http://www.georgehart.com/bagel/bagel.html
http://www.maa.org/features/lego.html
http://people.math.gatech.edu/~berglund/Mobius.html