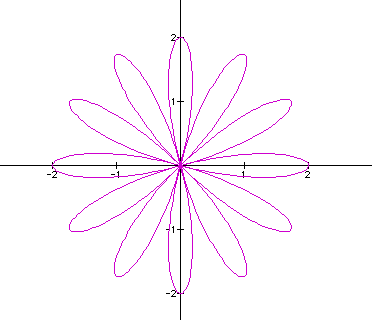
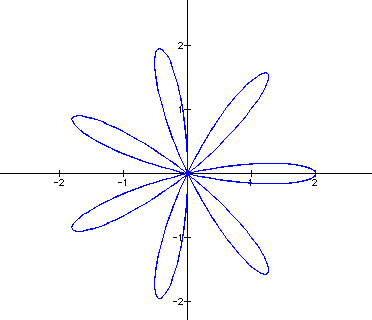
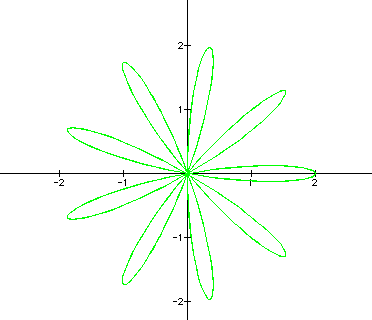
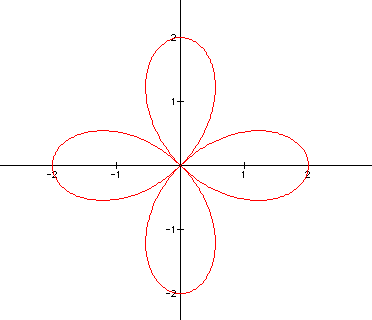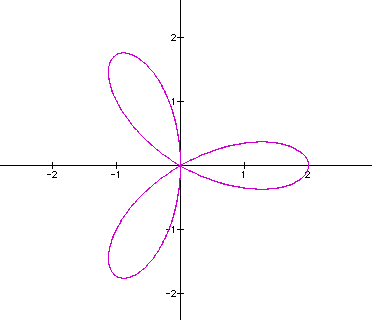The graphs of the equation are known as roses with various numbers of leaves. Below are graphs of the above equation with a = 1 and varied values for k. It appears that k affects the number of "petals" of the graph. If k is odd, then there are k petals. If k is even, then there are 2k petals. A negative value for k produces the same graph since cos Q = cos (- Q).
Notice that when k is odd, there is always one petal that "lies" on the x-axis in such a way that the x-axis goes through the center of the leaf (as if it were a vein through the center of the leaf). We will call this petal the "axis petal."
When k is even, there are 4 axis petals, each lying on the positive or negative side of the x- or y- axis.
Glance at the the graphs to verify these conclusions. Then go on to "What does a do?"