This write up is for problems #2 in Assignment
11.
Investigating Polar
Equations
by
Kimberly Burrell
and Brad Simmons
Investigation of
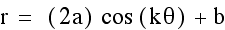After we investigated the polar equation
by varying "a" and "k", we were given a similar
polar equation except for the addition of "b". At this
point in our investigation, we will vary the "b" using
the before mentioned values for "a" and "k".
Again the cosine equation will remain symmetric about the x-axis.
We will now work with the cosine problem
(red) which will be graphed over the sine problem (blue).
When all values are 1, we have the
following graph.
The graph now has a distinct shape.
There appears to be a smaller curve inside the larger curve and
passing through the origin. The cosine graph still appears to
be rotated to the right. Will the curve behave like the previous
curves if we change the value of "b" to 2? Let's see.
The smaller curve appears to disappear.
However, there is still a noticeable bend at the origin of the
graph. The cosine graph (red) appears to have rotated to the right
as before.
Now, let us vary both "k"
and "b".
When a = 1, k = 2, and b = 2, we get
the following graph.
Here, we can observe two pedal-like
curves that are connected at the origin with the cosine being
rotated to the right.
Now let us change "a" to
2, also.
When a = 2, we get 4 pedal-like curves
that are connected at the origin. Two of these pedals are smaller
than the original two pedals. Again, the cosine graph is rotated
to the right. Now let us see what occurs when we change a = 5.
The pedals have expanded as with the
previous changes of "a", and there was a rotation to
the right. Therefore, if we change "k" the number of
pedals should change if the same principles for "k"
apply in this polar equation.
Let us try k = 5.
By observation one can see that there
are 5 large pedals and 5 small pedals which are inside the larger
ones.
Finally, we will let all values of
a, k, and b vary.
Here a = 2, b = 1, and k = 5. What
conclusions can you draw from this?
Return