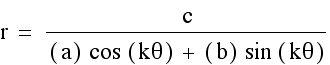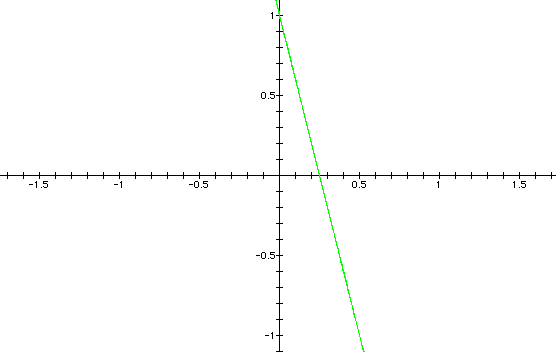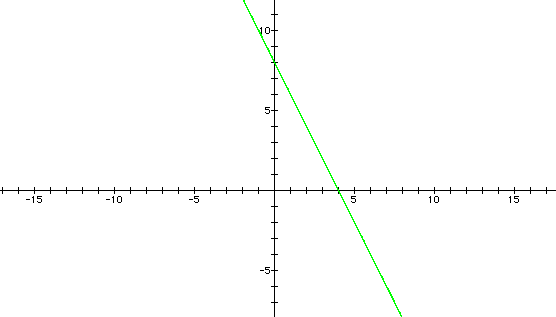This write up is for problems #2 in Assignment 11.

Investigating Polar Equations

by

Kimberly Burrell and Brad Simmons

Investigation of

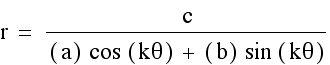We will begin this part of our investigation by holding "k", "b" and "c" constant at 1. We we vary the "a" and look for the change it makes in the graph of the polar equation.

Let us begin with a = 1

 

We get a linear graph with the slope = -1, the y-intercept = 1, and the x-intercept = 1.

Now we will let a =4.

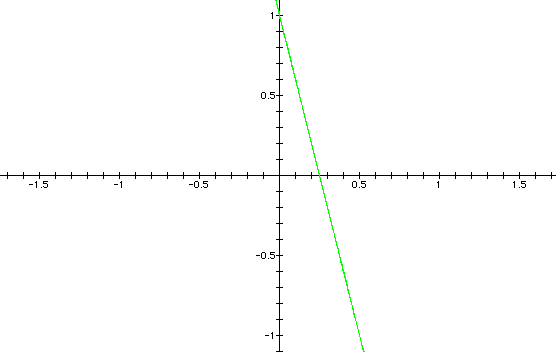As we see the graph has a y-intercept still at 1 and the x-intercept is now at 1/4. Could the x-intercept be determined by the ratio of c/a? If so, is the y-intercept determined by the ratio of c/b? If we continue to hold "k" constant at 1, we can vary "a", "b", and "c" to test our conjecture with regard to the intercepts.

Let c = 80, a = 20, and b = 10.

 

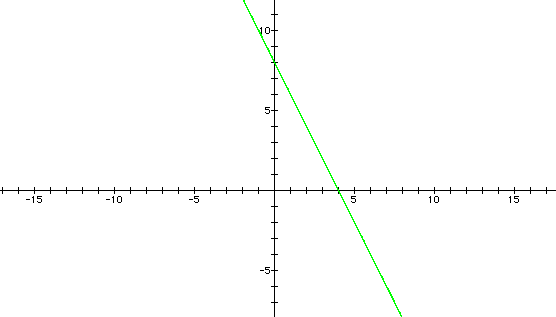As we see our conjecture holds true so far. The ratio of c/a = 4 which is the x-intercept. The ratio of c/b is 8 which is the y-intercept. Although two examples do not constitute a proof, one more case could be helpful to examine.

Let c = -50, a = 25, and b = -100

 

Once again our conjecture holds true. The ratio of c/a is equal to the x-intercept -2. The ratio c/b is equal to the y-intercept -1/2.

Now that we have seen how "a", "b", and "c" can change the graph of this polar equation, let us investigate what happen when we let "k" vary.

Let us hold "a", "b", and "c" constant at 1, and let k = 2.

 

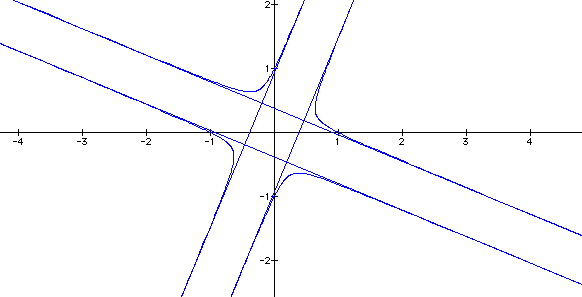The graph is a rectangle (maybe a square) that appears to have center at the origin. The extended side of the rectangle form asymptotes of what appears to be four parabolas.

What happens if we let k = 3?

 

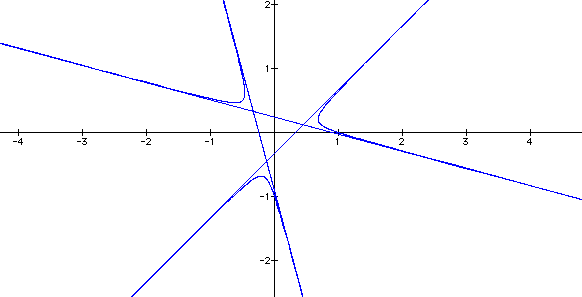This time the polygon is a triangle and its extended sides form the asymptotes of what appears to be three parabolas.

Can we make a similar conjecture to that of the pedals in the polar equation at the beginning of this write up? If k is an odd number, then the graph will be that of a polygon with k sides whose extended sides form the asymptotes for number of parabolas that is equal to k. Furthermore, if k is an even number, then the graph will be that of a polygon with 2k sides whose extended sides form the asymptotes for number of parabolas that is equal to 2k.

To test this conjecture and possibly explore this topic further please click here for a QuickTime for in which we will let k vary.

 

Finally, we will let c = 8, a = 4, b = 2 and k = 5.

 

 

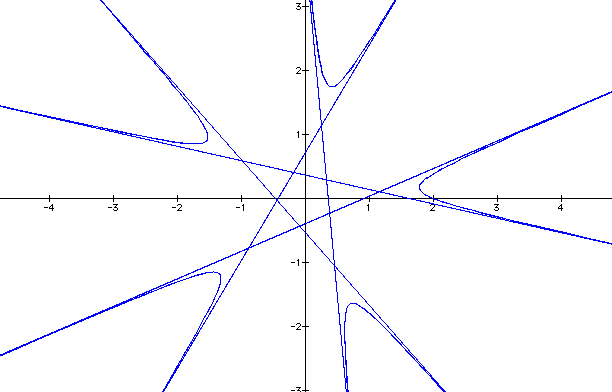The polygon has 5 sides as we might suspect since k = 5. How do the values of "c", "a", and "b" effect the intercepts of the extended sides of the polygon? Is there a relationship involving "c", "a", "b", and the vertex of each parabola? If there is a relationship, can a definite ratio be established similar to the linear graphs presented at the beginning of this part of our investigation?

Return