Assign9.html

This write up is for Assignment 9.

Pedal Triangles

Brad Simmons

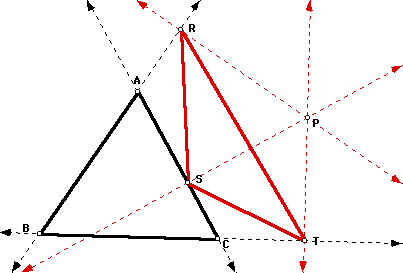
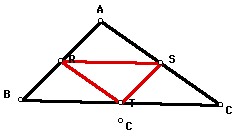
To begin our exporation and discussion of pedal triangles, we will examine the construction of a pedal triangle. The point P is a point in the same plane as triangle ABC. The (red) pedal triangle RST for triangle ABC and pedal point P is shown in the image below. By constructing perpendiculars to each side of triangle ABC that pass through the pedal point P, we can locate the vertices of the pedal triangle RST. The vertices of the pedal triangle are located at the intersection points of the (red) perpendicular lines and the sides (or the extention of the sides) of triangle ABC.

For a GSP sketch that can be manipulated please click here.

For a GSP script to construct a pedal triangle to triangle ABC please click here.

Geometer's Sketchpad 4.0 users please click here.

It may be interesting to look at the pedal triangle of triangle ABC if the pedal point P is located at a particular specified point such as the centroid. In other words, the pedal point P is the centroid of triangle ABC. The image below shows the centroid (G) of triangle ABC and the pedal triangle RST with the pedal point P outside of triangle ABC.

Now if the pedal point is the centroid (G), then the vertices of the pedal triangle RST appear to be located on the sides of triangle ABC. The point T also appears to be the midpoint of segment BC.

To explore the conjectures made above or any other conjectures that could be made, please click here for a GSP sketch that can be manipulated.

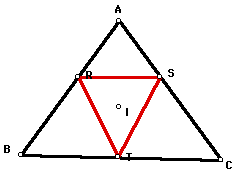
If the pedal point P is the Incenter (I) of triangle ABC, then the pedal triangle RST appears to be the same a the image above.

For a GSP sketch that can be manipulated please click here.

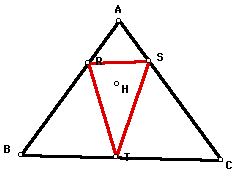
If the pedal point P is the orthocenter (H), then the (red) pedal triangle looks similar.

For a GSP sketch that can be manipulated please click here.

If triangle ABC is manipulated so the orthocenter (H) is outside triangle ABC, the pedal triangle RST for the pedal point H appears to have one vertex R on one side AB (see the image below). Will one vertex of the pedal triangle always be located on at least one side of triangle ABC?

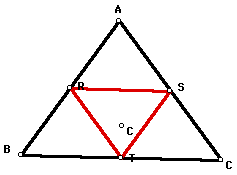
Now if we move the pedal point of triangle ABC to the circumcenter of ABC, then the (red) pedal triangle once again appears to have its vertices R, S, T located on the sides of triangle ABC.

For a GSP sketch that can be manipulated please click here.

If triangle ABC is manipulated so its circumcenter (C) is outside of triangle ABC, then the pedal triangle RST for the pedal point C still appears to have its vertices R, S, T located on the sides of triangle ABC (see the image below).

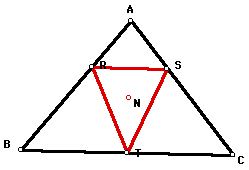
The image below shows triangle ABC and the pedal triangle RST for the pedal point N. The point N is the center of the nine point circle for triangle ABC.

For a GSP sketch that can be manipulated please click here.

Now that we have explored moving the pedal point to the different centers of triangle ABC, it may be interesting to move the pedal point to one of the sides of triangle ABC. The image below shows the pedal triangle RST for triangle ABC when the pedal point P is on side AB.

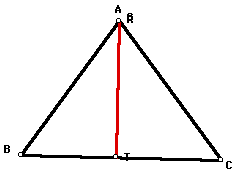
When the pedal point is moved to vertex A, then the (red) pedal triangle degenerates to a line segment AT. This line segment is called the Simson Line.

For a GSP sketch that can be manipulated please click here.

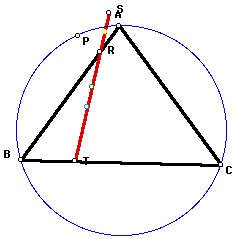
If the circumcircle (blue) is constructed for triangle ABC, then we can see that the Simson Line is formed when the pedal point P lies on the the circumcircle.

If we locate the midpoints of the three sides of the pedal triangle, then we can examine the locus of each midpoint as the pedal point P moves around the circumcircle.

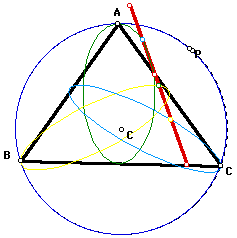Does each median of triangle ABC lie on the major axis of an ellipse formed by the locus of a midpoint of a side of the pedal triangle for pedal point P when P moves around the circumcircle of triangle ABC?

For a GSP sketch that can be manipulated please click here.

If we move the pedal point P around a circle (black dashed circle) with its center at the circumcenter of triangle ABC and its radius larger than that of the circumcircle of triangle ABC, the locus of the three midpoints of the sides of the (red) pedal triangle can be examined. How do they compare to the locus of midpoints observed above?

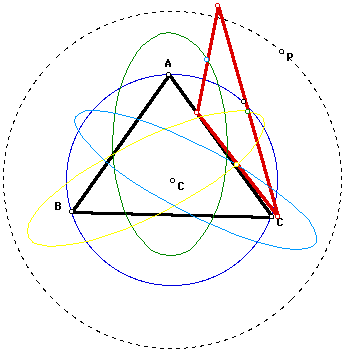Now what if we move the pedal point P around a circle (black dashed circle) with its center at the circumcenter of triangle ABC and its radius smaller than that of the circumcircle of triangle ABC. The locus of the midpoints of the sides of the (red) pedal triangle are elliptical. Will this always be the case? (see the image below)

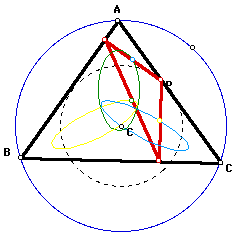
The image below can be constructed if we trace the extention of the simson line as the pedal point P moves around the circumcirle of triangle ABC.

For a GSP sketch that can be manipulated please click here.

Is there a point on the circumcircle for pedal point P that has side AC as its Simson line? What about AB as its Simson line? What about BC as its Simson line?

For a GSP sketch that may help answer these questions please click here.

Return