

This is the write-up for assignment 11.
In this write-up we will be investigating polar
equations. Click here
for a quick review of the polar coordinate system.
Let's consider the equation 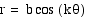 and take a look at several graphs while
we let the b value remain constant and let the k value
vary. We only look at k values that are positive integers.
and take a look at several graphs while
we let the b value remain constant and let the k value
vary. We only look at k values that are positive integers.
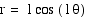
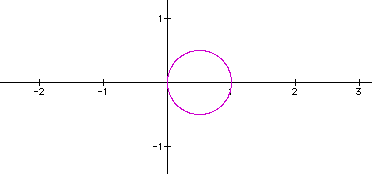
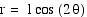
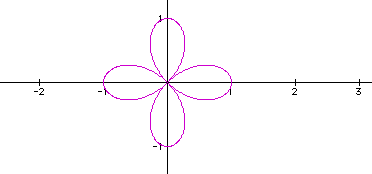
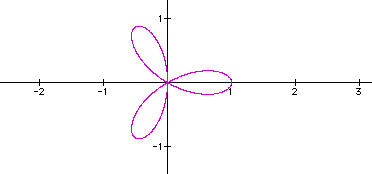

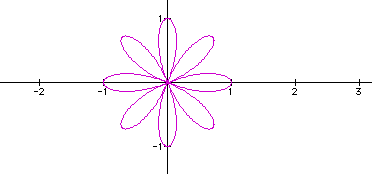
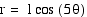
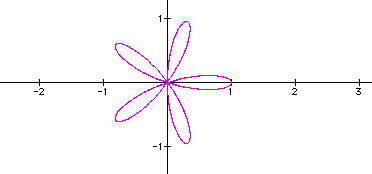
Equations of the form 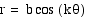 , where b is a constant and k
is a positive integer, produce graphs called roses.
, where b is a constant and k
is a positive integer, produce graphs called roses.
The radius of the rose is determined by the
coefficient b in 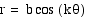 .
.
We can see that the k value effects the number of petals in the rose. If the k value is odd, there will be exactly k petals. If the k value is even, there will be exactly 2k petals. (In the examples, the k value was always an integer. If k is not an integer, then a complete petal will not be formed.)
Click here for a dynamic presentation in which the b value remains constant (b=1) and the k value varies from -10 to 10.
Now, let's take a look at the equation:
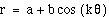
Let k=1 and vary the a and b values ( but we will keep a=b ) in the equation.
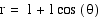

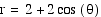

When a=b in the equation 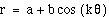 and k=1, we
get graphs that are called cardiods.
and k=1, we
get graphs that are called cardiods.
Now, if we let a and b be different numbers and keep k=1, we get the sample graphs below:
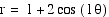
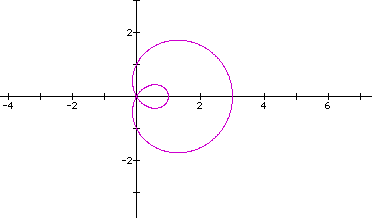
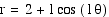
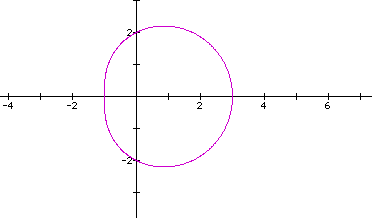
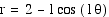
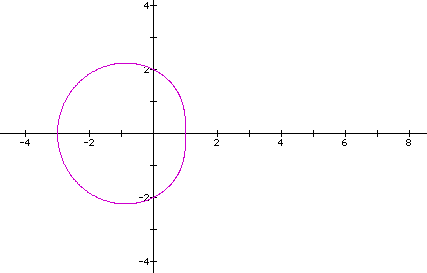
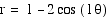
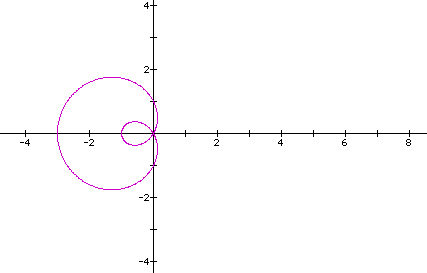
All of the graphs above are called limacons.
Some of the graphs above had inner loops. These are called limacons with an inner loop.
The limacon 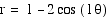 has an inner loop. A close look at the graph and the
equation reveals that these loops correspond to negative values
of r. For
has an inner loop. A close look at the graph and the
equation reveals that these loops correspond to negative values
of r. For 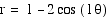 , we
have r=0 when
, we
have r=0 when 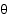 =
= /3 or 5
/3 or 5 /3, and
/3, and
r<0 for  /3 <
/3 < 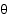 <
5
<
5 /3. For these
/3. For these
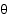 values the curve
is drawn on the opposite side of the origin or pole.
values the curve
is drawn on the opposite side of the origin or pole.
These graphs also have symmetry with respect
to the x-axis. This symmetry occurs because the cosine function
is an even function ( i.e. for any 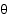 , cos
, cos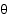 =cos
(-
=cos
(-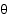 ) ).
) ).
If we were to replace the above equations with the sine function, we would obtain basically the same type graph, except the graph would be symmetric with respect to the y-axis, because the sine function is odd.
Below are some graphs of the above equation letting a=1 and b=1 and varying the k value.
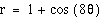
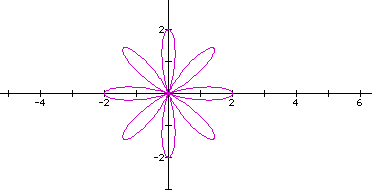
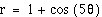
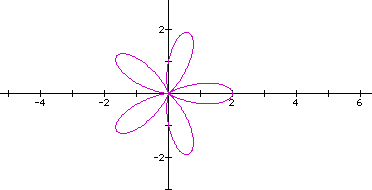
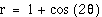
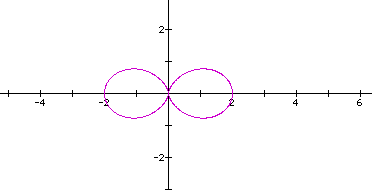
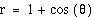

Click here
for a dynamic presentation of the graph of 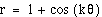 where the value of k varies from -10
to 10.
where the value of k varies from -10
to 10.
So, when a and b are both the same value in
the equation 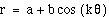 and
we vary the k value (positive integer values only), we still obtain
a curve that that resembles a rose. The number of petals on the
rose is equal to k. This is different when k was
an even integer in the equation
and
we vary the k value (positive integer values only), we still obtain
a curve that that resembles a rose. The number of petals on the
rose is equal to k. This is different when k was
an even integer in the equation 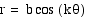 .
.
Now, let's take a look at how the a and
b values effect the equation of 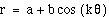 . In the previous examples above, we
let a=1 and b=1. (Notice that in all of the examples,
the petal has a maximum length of 2). Now, if we let the a
and the b value vary (keeping both the same value)
and let the k value a remain a constant we can see from the examples
below that the length of the petal will depend on the value of
a and b.
. In the previous examples above, we
let a=1 and b=1. (Notice that in all of the examples,
the petal has a maximum length of 2). Now, if we let the a
and the b value vary (keeping both the same value)
and let the k value a remain a constant we can see from the examples
below that the length of the petal will depend on the value of
a and b.
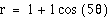
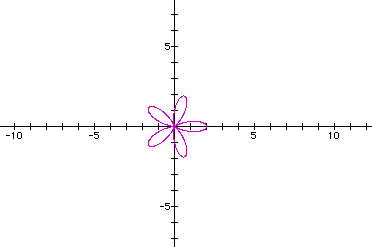
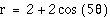

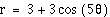
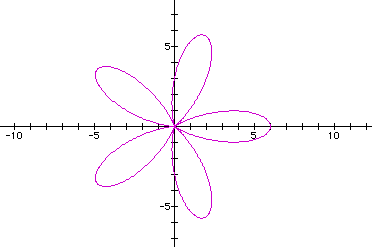
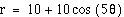
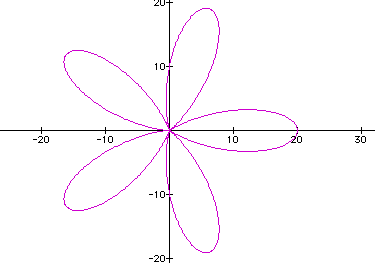
We can see from the examples above that the
lengths of the petals got longer as we increased the values of
a and b (keeping a and b the same
value).