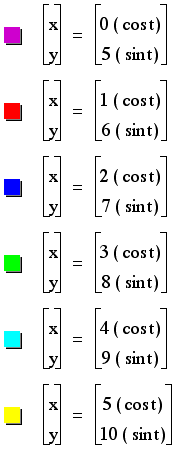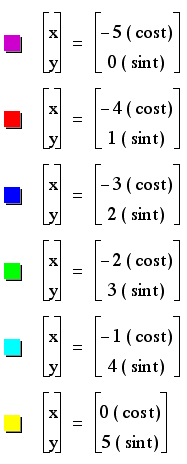Now we will look at
the cases when
a < b.
In this part of our
investigation of parametric equations, we will be using the parametic
equations
 where
0 < t < 2Pi.
where
0 < t < 2Pi.
a = b - 1

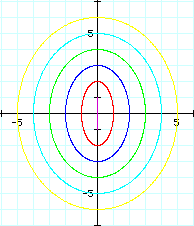 It is easy to conclude
from the above sketch that each graph forms an ellipse that
crosses the x-axis
at a positive/negative a value and a positive/negative b value
and has its center at the origin.
We get the major axis
lying on the y-axis, and the minor axis lying on the x-axis.
(Notice in purple that
when a = 0, you will get a vertical line on the y-axis with a
length of positive/negative b.)
It is easy to conclude
from the above sketch that each graph forms an ellipse that
crosses the x-axis
at a positive/negative a value and a positive/negative b value
and has its center at the origin.
We get the major axis
lying on the y-axis, and the minor axis lying on the x-axis.
(Notice in purple that
when a = 0, you will get a vertical line on the y-axis with a
length of positive/negative b.)
a = b - 5
Resulting with positive
a values
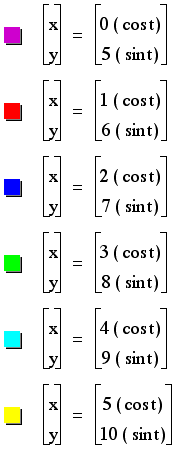
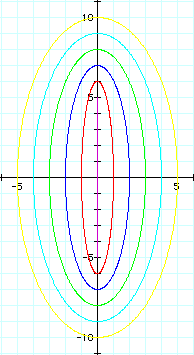 We get the same sort
of picture with these values, just a bit more exagerated vertically,
because obviously the b value controls the heigth of the graph.
(Once again notice
the a = 0 line)
We get the same sort
of picture with these values, just a bit more exagerated vertically,
because obviously the b value controls the heigth of the graph.
(Once again notice
the a = 0 line)
a = b - 5
Resulting with negative
a values
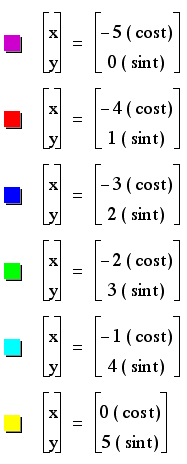
 But it is easy to see
that this graph is very different from the first two.
The first thing that
we notice is that we sometimes get the opposite results for the
major and minor axes.
This forces us to take
absolute value into consideration.
(Notice b = 0
and a = 0)
But it is easy to see
that this graph is very different from the first two.
The first thing that
we notice is that we sometimes get the opposite results for the
major and minor axes.
This forces us to take
absolute value into consideration.
(Notice b = 0
and a = 0)
Conclusions:
When IaI < IbI
, and a or b is not equal
to zero, the graph of the parametric equation
 results in ellipses
centered at the origin, with a major axis on the y-axis and the
minor axis on the x-axis.
Return to
Investigation HomePage
results in ellipses
centered at the origin, with a major axis on the y-axis and the
minor axis on the x-axis.
Return to
Investigation HomePage
 where
0 < t < 2Pi.
where
0 < t < 2Pi. where
0 < t < 2Pi.
where
0 < t < 2Pi.