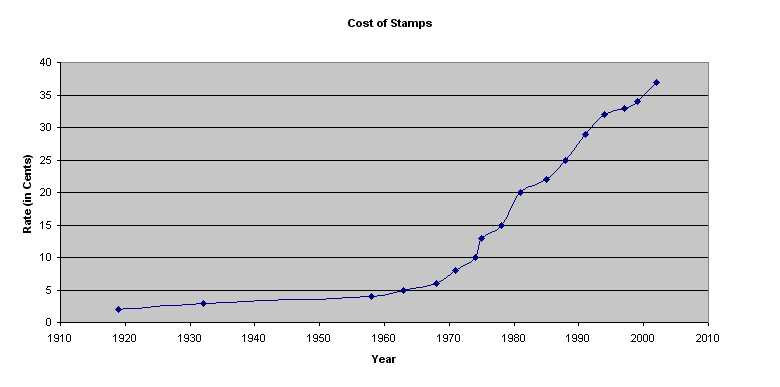
It seems that the cost of stamps has been rising at an unprecedented rate. Will it ever end? Can we determine when the next increase will be? When will the cost of stamps be $1.00? In order to predict these question, let's first take a look at the past data. The following table displays the rising cost of stamps from 1919 to 2002.
| Year | Rate (in Cents) |
| 1919 | 2 |
| 1932 | 3 |
| 1958 | 4 |
| 1963 | 5 |
| 1968 | 6 |
| 1971 | 8 |
| 1974 | 10 |
| 1975 | 13 |
| 1978 | 15 |
| 1981 | 20 |
| 1985 | 22 |
| 1988 | 25 |
| 1991 | 29 |
| 1994 | 32 |
| 1997 | 33 |
| 1999 | 34 |
| 2002 | 37 |
Furthermore, we can use the graphing functionality of MS Excel to display this data to visually see what phenomena is going on.

What type of graph does this look like? It appears to be a power function. Within Excel's Chart Wizard option, we can compare this graph to that of a power function. In the following graph, the line in red is the power function and the line in blue is the original plotted function.
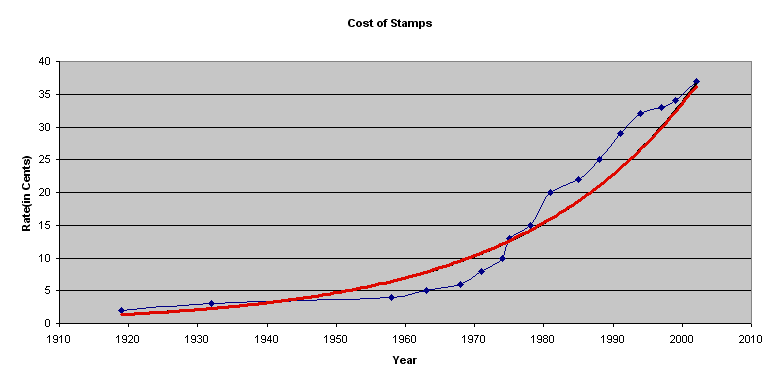
Is the power function a good match in predicting the next rise
in the cost of stamps? To determine that, the correlation
coefficient needs to be determined between the two functions.
The closer it is to one the better the "fit".
A power function is in the form of y = axb. In
our situation, the equation is rate = a(year)b.
We now need to input the stamp data, into this function and then
determine the correlation coefficient. By taking the
log of both sides of the power function:
log(rate) = log (a(year)b) = log(rate)
= log a + b*log(year).
We can compute the value of the logarithms for our values of rate and year by using Excel's function wizard. The table below shows these values. Then we can compute the correlation coefficient via Excel as well, by using the CORREL function. As it turns out, the coefficient is 0.970999716. Therefore, we can assume that the rising costs of stamps can be represented as a power function.
| Log(year) | Log(rate) |
| 3.283074975 | 0.301029996 |
| 3.286007122 | 0.477121255 |
| 3.291812687 | 0.602059991 |
| 3.2929203 | 0.698970004 |
| 3.294025094 | 0.77815125 |
| 3.294686624 | 0.903089987 |
| 3.295347148 | 1 |
| 3.2955671 | 1.113943352 |
| 3.296226287 | 1.176091259 |
| 3.296884476 | 1.301029996 |
| 3.297760511 | 1.342422681 |
| 3.29841638 | 1.397940009 |
| 3.29907126 | 1.462397998 |
| 3.299725154 | 1.505149978 |
| 3.300378065 | 1.51851394 |
| 3.300812794 | 1.531478917 |
| 3.301464073 | 1.568201724 |