It has now become a rather standard exercise, with available technology, to construct graphs to consider the equation
and to overlay several graphs of
for different values of a, c, or c as the other two are held constant. From these graphs discussion of the patterns for the roots of
can be followed. For example, if we set
for b = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture is obtained.

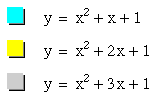
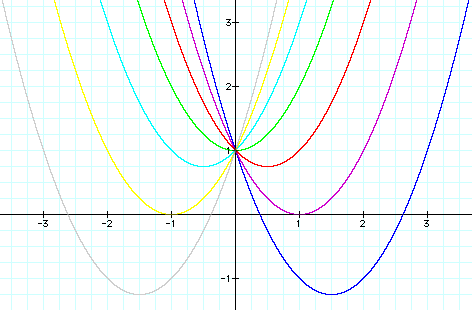
We can discuss the "movement' of a parabola as b is changed. The parabola always passes through the same point on the y-axis (the point (0,1) with this equation). For b < -2, the parabola will intersect the x-axis in two points with positive x values (i.e. the original equation will have two real roots, both positive). For b = -2, the parabola is tangent to the x-axis and so the original equation has one real and positive root at the point of tangency. This point of tangency is (1,0). For -2 < b < 2, the parabola does not intersect the x-axis -- the original equation has no real roots. Similarly for b=2 the parabola is tangent to the x-axis (one real negative root) and for b > 2, the parabola intersects the x-axis twice to show two negative real roots for each b.
Now we can consider the locus of the vertices of the set of parabolas graphed from
We can see, by looking at the above graphs, that this locus is a downward-opening parabola with a vertex at (0,1). To find an equation for this new parabola, we can use the vertex.
is the equation most commonly used when we know the vertex (b, c) and the amount of stretch (a). In this case, b=0 and c=1. There is no stretch (because the numbers change in the same increments as they do in the graph of y = x^2, but a is negative because the graph opens downward. Therefore, the equation for this locus is:
We can look at this graph overlaid on the graphs of different values of b to see if this is in fact the correct equation:
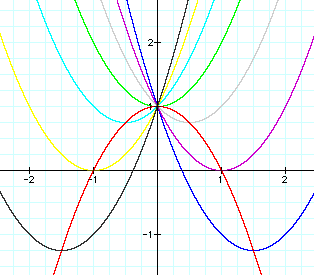
This does work, so our equation was correct. We can generalize to conclude that the locus of the vertices of the set of parabolas graphed from
where either a and c are constant and b is varied, is the parabola with the vertex at the point of intersection of each of these parabolas. The locus parabola opens in the opposite direction of the other parabolas, so if a is negative the locus opens up and if a is positive, the locus opens down.
Graphs in the xb plane
Consider again the equation
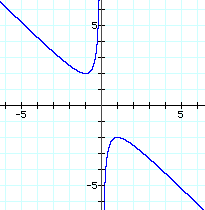
Now graph this relation in the xb plane. We get the following graph.
If we take any particular value of b, say b=3, and overlay this equation on the graph we add a line parallel to the x-axis. If it intersects the curve in the xb plane the intersection points correspond to the roots of the original equation for that value of b. We have the following graph.

The graph has one set of assymptotes, with the vertical assymptote being x=0 and the diagonal assymptote being x+y=0. It is a hyperbolic graph that exists only in the second and fourth quadrants.
For each value of b we get a horizontal line. We can therefore use this information to determine the roots for any equation of this form in different values of b. For example, when b>2, we get two negative roots. When b = 2, there exists one real root at x = -1. When -2 < b < 2, the equation has no real roots, and when b=-2, the equation has one real root at x = 1. When b < -2, the equation has two positive real roots.
Another situation to consider is the situation in which c = -1 instead of c = +1.
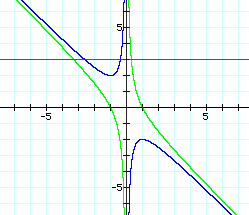
In this situation, every horizontal
line intersects the graph in two points, one positive and one
negative. This means that for every value of b, two real roots
exist, one positive and one negative. The assymptotes are the
same as those when c = +1. When looking at both graphs on the
same set of axes, we can see that the line 2x+y=0 divides the
entire graph into two symmetrical halves.
Graphs in the xc plane
In the following example the equation
is considered. If the equation is graphed on the xc plane, it is easy to see that the curve will be a parabola. For each value of c considered, its graph will be a line crossing the parabola in 0, 1, or 2 points--the intersections being at the roots of the original equation at that value of c. In the graph, the graph of c = 1 is shown. The equation
will have two negative roots--approximately -0.2 and -4.8.
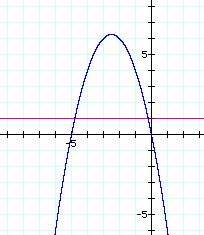
When c is greater than 6.25, the equation will have no real roots, because the horizontal line will not intersect the parabola in any points. When c is equal to 6.25, the equation will have one real root because c=6.25 is tangent to the parabola. This point is the vertex of the parabola, which means that it is the maximum point. When c is less than 6.25, the equation will have two real roots because the horizontal line intersects the parabola in two points.
The roots of this equation are positive when c is positive, and negative when c is negative. All negative values of c yield two negative roots. This parabola does not have assymptotes, but it can be divided into two symmetrical halves by the line x = -2.5. Each value of c which contains two real roots will have one root on the left side and one root on the right side. Sine c=6.25 lies on the line x=-2.5 and on the parabola, this value of c only contains one root.
This format can be used to
find the roots of the equation for any value of c. All that needs
to be done is for us to draw the line for the value of c and observe
the points at which it intersects the parabola.
Graphs in the xa plane
This example will use the equation
to demonstrate a way to find the roots of a quadratic equation for different values of a. When the equation is graphed in the xa plane, it creates a strange figure with the c- and x- axes as asymptotes in certain parts of the graph.
Again, we can determine the real roots of this equation by drawing a horizontal line at the value of a. In the figure below, we have used a = -2.
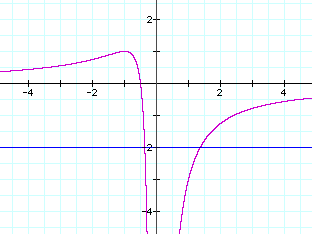
We can see that a = -2 yields two roots, one positive and one negative. Any value of a less than zero will yield one positive and one negative root. When a is equal to zero, one real root exists, which is x = -.5. When a is between 0 and 1, it can be seen that two real roots are present, both of which are negative. When c=2, again there is one real root, this time at -1. If c is greater than 2, no real roots exist.