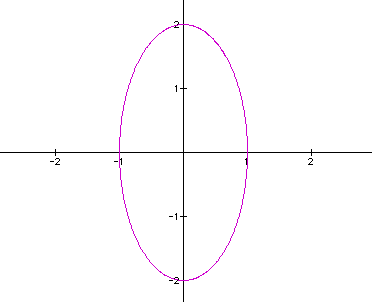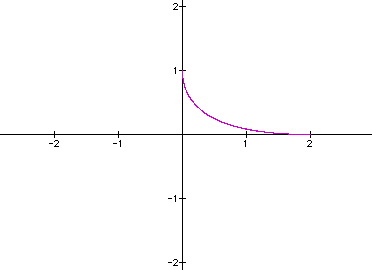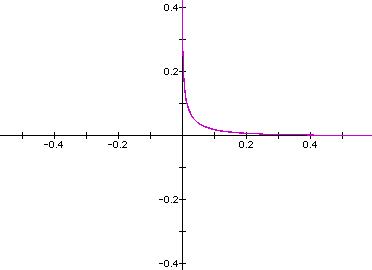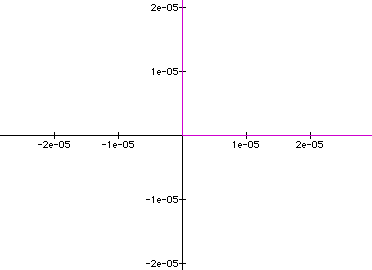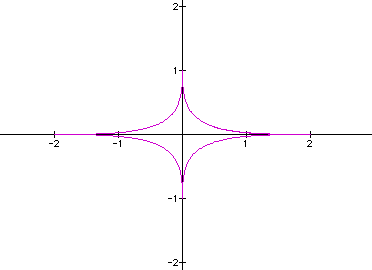Parametric Curves involving sin and cos

Para-Fun

Fun with Parametric Curves

In this exploration, I am investigating parametric curves involving sin and cos. I am first looking at

x = a cos(t)

y = b sin(t)

where a = b, a < b, and a > b.

It did not take many examples discover that when a = b, the graph is a circle with radius =1 centered about the origin. Here, I have shown where a = b = 1.

When a < b (a = 1 and b = 2), the graph is an ellipse centered about the origin where our vertical distance from the center is 2 and the horizontal distance from the center is 1. This gives insight in that the a = horizontal distance from the center of the ellipse and b = vertical distance from the center of the ellipse.

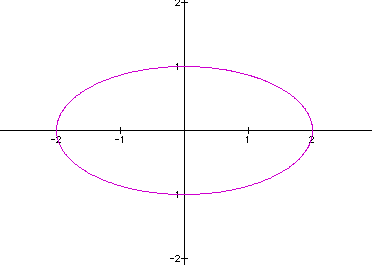
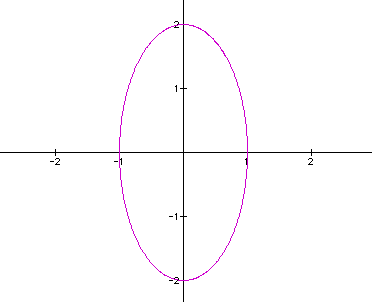This leads me to believe that when a = 2 and b = 1 (a > b), I will get a graph of an ellipse with a horizontal distance of 2 (a) from the center and a vertical distance of 1 (b).

Next, I want to see what happens when the sin and cos are squared in:

x = a (cos(t))^2

y = b (sin(t))^2

where a = b, a < b, and a > b.

If a = b (=2), we have a graph that looks like this:

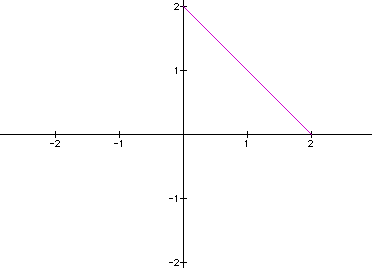This is not at all what I was expecting. I was expecting to see some sort of graph with a loop -- not a straight line. Since a = b = 2 and the x-intercept = y-intercept = 2, we may be able to deduce that the a = x-intercept (because a represented the horizontal distance on the previous example) and b = y-intercept (because b represented the vertical distance on the previous example). It does not surprise me that the entire graph sits in quadrant I because you are squaring a value and then multiplying by a positive number. Therefore, if a = b = -#, the graph will lie entirely in quadrant III where all values are negative because after squaring, you will have a positive value multiplied by a negative number. Let's check to make sure. The following graph is a = b= -2.

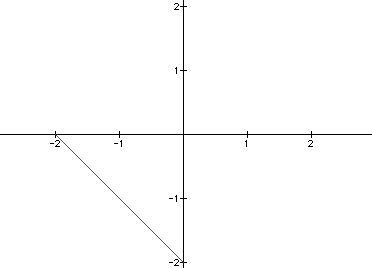Nothing surprising there. Now, let's check my conjecture about a = x-intercept and b = y-intercept. In the following graph, I let a = 1 and b = 2. This graph should rest in quadrant I due to all values being positive and the x-intercept should equal 1 and the y-intercept should equal 2.

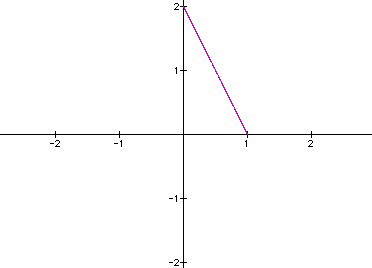Again, nothing new and nothing surprising. What if a is positive and b is negative? Where will the graph lie? Our x-value will be positive and the y-value will be negative, leading us to believe that this will lie in quadrant IV. Let's see where a = 1 and b = -2.

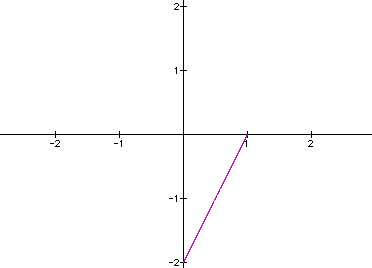WOW! I am really on today! Let's move on to cubing the sin and cos. Will it make another circle/ellipse? Or will it have other fun loops? Let's look at:

x = a (cos(t))^3

y = b (sin(t))^3

where a = b = 1.

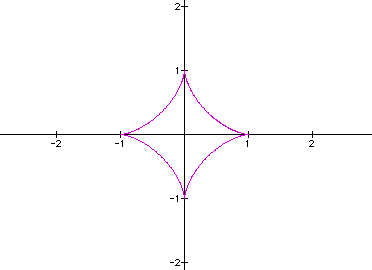OK! This is finally fun! I promised you fun in the title and we are finally there! So if a = b = 2, will we have the same shape stretched out to 2 units on each side of the center?

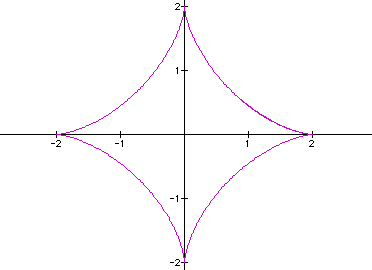This is getting cool! What if a < b or a > b? Let a = 1 and b = 2 and then switch the values. I think that our diamond will extend from -1 to 1 on the x-axis and from -2 to 2 on the y-axis when a = 1 and b = 2. I think that our diamond will extend from -2 to 2 on the x-axis and from -1 to 1 on the y-axis when a = 2 and b = 1.Let's see!

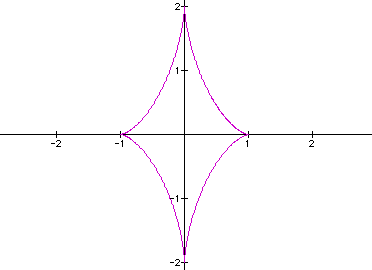
Due to the fact that cubes can be positive or negative, it won't matter if a and/or b is negative because the negative values will still come up and the positive values will still come up -- just in a different "order" in a t-table. However, order does not matter when you are plotting points. This has me interested in raising sin and cos to the forth power and the fifth power! Will all even powers lead to that boring straight line? Will odd powers lead to other interesting diamond like figures?

For a = 2 and b = 1 in

x = a (cos(t))^4

y = b (sin(t))^4

we get the graph:

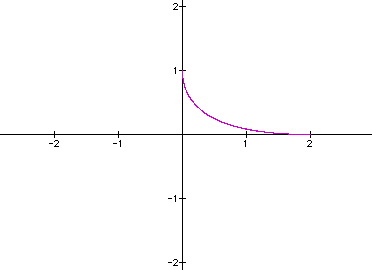Not a boring straight line, but close! It appears as if the line is being pulled in by the center (the origin) when the power is even. What if the power was 10?

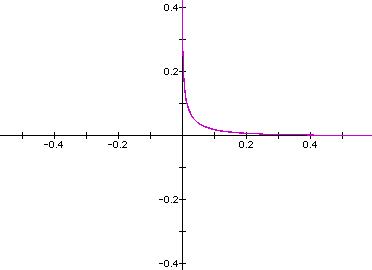Note the change in the scale on the axes. If we pick an even power big enough, our graph will soon resemble a right angle. What if the power was 50?

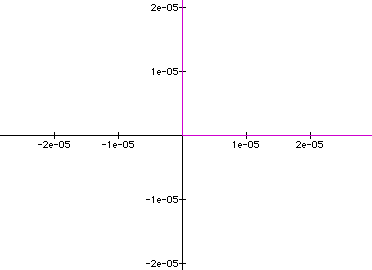Look how small the values on the axes are and the graph still looks like a right angle!

For odd powers, we will have diamond shapes.

For a = 2 and b = 1 in

x = a (cos(t))^5

y = b (sin(t))^5

we get the graph:

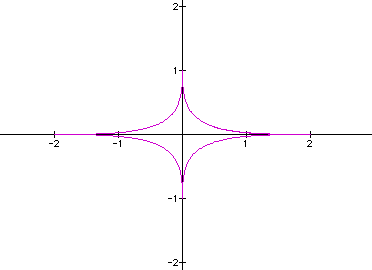Our diamond is sinking in closer and closer to the center (the origin) for odd powers. What if the power was 11?

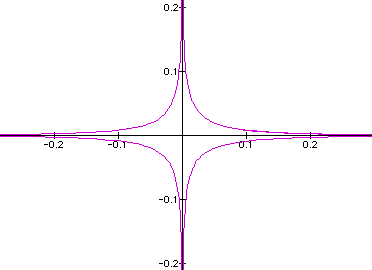What if the power was 49? Will it be sucked into the axes and look like nothing is there beside the color on the axes?

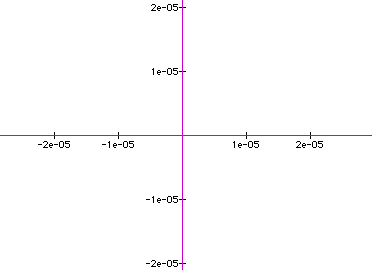Truly remarkable! This has been a fun exercise. Again, not to worry, there is plenty more where this came from!

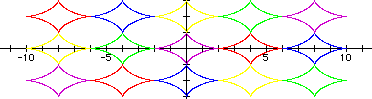Kind of reminds me of Argyle socks!

Return