Intermath: Investigations: Geometry: Circle
What happens to the circumference of a circle if you double the diameter? If you triple the diameter? If you halve the diameter? As the diameter increases (or decreases) in measure, how does the circumference change? Why does this change occur?
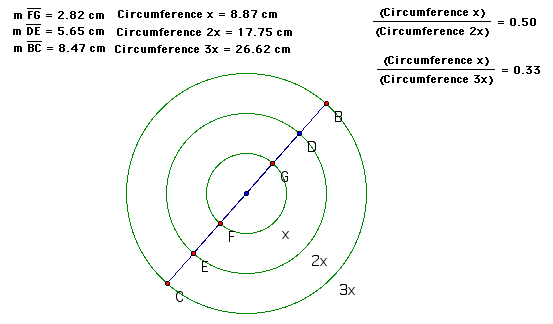
(This change will be shown with Geometer's Sketchpad. Please note that the circles constructed are double and triple the diameter of the innermost circle. The points that can be used to adjust the size of the circles are only the blue points. Note how the measurements change as the size is adjusted.)
Looking at the sketch below the diameters' measures are given to show that they are in accordance with the problem above. The corresponding circumferences to these diameters are given out to the side of the corresponding diameter. From looking simply at the measurements' ratios, it is clear that there is a distinct relationship between the increase in diameter length and the increase in circumference. It is in accordance with the proportion of the original cirlce x.

Now to make sense of what can be seen. First, think of the equation for the circumference of a circle. Circumferece = 2*pi*radius. Continuing this, we can simplify the equation with simple substitution. Knowing that diameter = 2*radius, Circumference also equals pi*diameter. Given that the only variable in the equation is the diameter of the circle, the circumference would vary along with only that variable.