Lesson 4:

Parabolas

By Carly Coffman

 

 

 

Or

 

 

 

Definitions: 

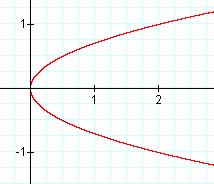
Ø    A parabola is the set of all points (x,y) in a plane that are equidistant from a fixed line, the directrix, and a fixed point, the focus, not on the line.

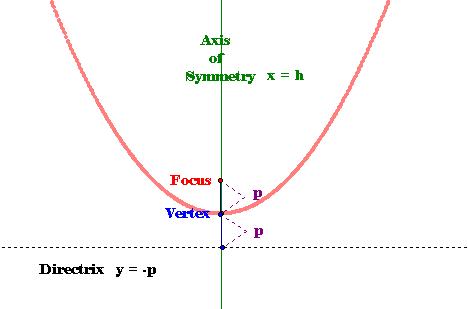
Ø    The vertex is the midpoint between the focus and the directrix.

Ø    The axis of the parabola (or axis of symmetry) is the line passing through the focus and the vertex.

 

Look at the creation of a parabola (you must have GSP to see this link):  Parabola

 

Let’s explore the parabola a bit.  In the equation, , let’s look at the affect h and k have on the graph.

 

1)    Do you have any predictions as to what increasing h and k will do to the graph?

     *Remember to respond in complete sentences.

 

Let’s see if you were on target or not.  Click on the play button at the bottom of the window.  Exploration 1

 

2)              What is the relationship between h and the parabola graph?

 

Now, let’s test what k does to the graph.  Click on the play button at the bottom of the window.    Exploration 2

 

3)              What is the relationship between k and the parabola graph?

 

Now you should be able to tell where the vertex of each of the following parabolas lies.  State the vertex of each parabola in a complete sentence. Copy and paste the equation into your Word document.

    

4)             

 

5)             

 

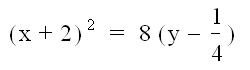
Notice also that all of the equations we have worked with so far have had the x term squared.   Each one of these graphs has been concave up, which means the vertex is the minimum point on the y-axis and the graph looks like a smiley face.

 

6)              What do you think would happen if there was a negative sign in front of the parenthesis of the x-term? 

 

Let’s see if your prediction was correct.  Exploration 3 

 

7)              Was your prediction correct?  How did the negative sign affect the graph?

 

When the vertex is the maximum point on the graph, the graph is concave down.  These parabolas look like a frown.

 

Let’s see what happens when the y-term is squared. 

 

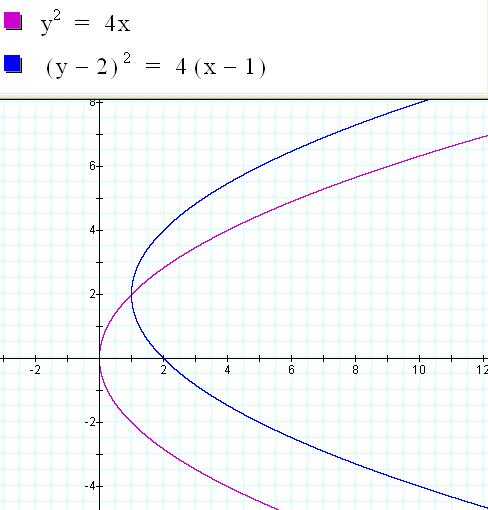
State the vertex and axis of symmetry of the following parabolas.   Remember the axis of symmetry is the equation of the line of symmetry for each parabola.

     8)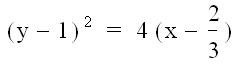  

    

     9)  

 

10)       What do you predict will happen to the parabolas above when a negative sign is placed in front of the parenthesis for the y-term?  Test your prediction and tell whether or not your prediction was correct.

Now, we have one last aspect of parabolas to explore.  The last aspect is the value of p in each parabola equation. In most of the equations above, p has been one. 

 

11)       What do you think p affects on the graph of a parabola? (take a guess if you do not know)

 

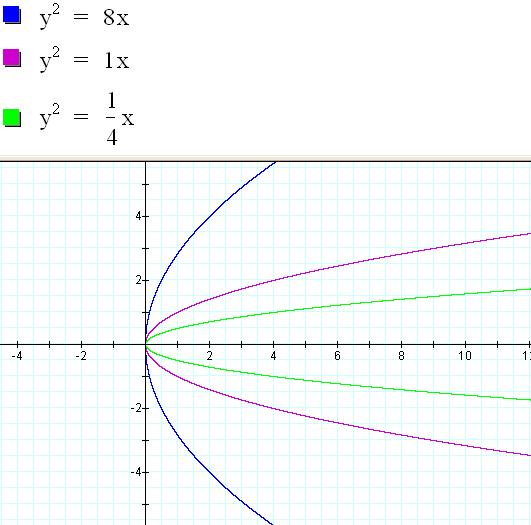
Remember, the coefficient of x is 4p.  So, in order to get the value of p we must divide the coefficient of x by 4.  The first p is 2, the second p is ¼ and the third p is 1/16. 

    

12)       As p decreases, what happens to the graph of the parabola?

 

 

We have finished exploring the variables of parabolic equations.  Print your Word document and file it with your other conic explorations.

 

Congratulations you have finished your parabola exploration!

Return to Home Page                                                                 Next Lesson