Some Different Ways to Examine

ax² + bx + c = 0

 

By James W. Wilson and Donna Greenwood

University of Georgia

It has now become a rather standard exercise, with available technology, to construct graphs to consider the equation

ax² + bx + c = 0

and to overlay several graphs of 

y = ax² + bx + c

for different values of a, b, or c as the other two are held constant.From these graphs discussion of the patterns for the roots of

ax² +bx + c = 0

can be followed.For example, if we set 

y = x² + bx + 1

for b = -3 (magenta), -2 (red), -1 (blue), 0 (green), 1 (aqua), 2 (yellow), 3 (gray) and overlay the graphs, the following picture is obtained.

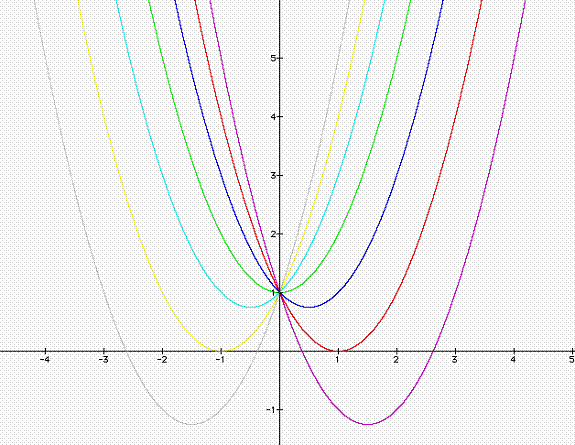
We can discuss the 'movement' of a parabola as b is changed. The parabola always passes through the same point on the y-axis (the point (0, 1) with this equation). For b < -2 the parabola will intersect the x-axis in two points with positive x values (i.e. the original equation will have two real roots, both positive). For b = -2, the parabola is tangent to the x-axis and so the original equation has one real and one positive root at the point of tangency.For -2 < b < 2, the parabola does not intersect the x-axis - the original equation has no real roots.  Similarly for b=2 the parabola is tangent to the x-axis twice to show two negative real roots for each b.

The locus of a parabola

y = x² + bx + 1

Here's a picture of a GSP sketch that animates the movement of the vertex of this family of parabolas.

If you'd like an explanation of the construction, click here. The locus is clearly another parabola. To get the equation of that parabola, start with the equations for the x and y coordinates of the vertex. For the general case, that'sx = -b/2a and y = f(-b/2a). In this equation, a = b = c = 1, so the equation x-coordinate is x = -b/2. The y-coordinate is given by y = (-b/2)² + b(-b/2a) + 1, 

or y = (b²/4) - b²/2 + 1, which simplifies to -b²/4 + 1 or ? (-b/2) ² + 1. Therefore, the equation of the vertex is y = -x² + 1.

Graphs in the xa plane

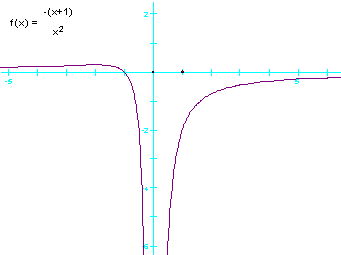
If we take particular values of a and overlay the equation on the graph we add lines parallel to the x-axis.If the lines intersect the graph in the xa plane the intersection points correspond to the roots of the equation for that value of a.Here are a few values of a.

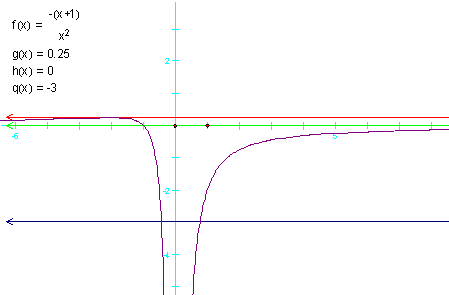
The red line is a = .25, the green is a = 0 and the dark blue is a = -3.

 

Value of a	Number/type of roots
a > .25	no real roots
a = .25	one negative real root
0 < a < .25	two negative real roots
a < 0	one positive, one negative real root

So, is a = .25 a lucky guess?Not quite.It's not as easy to see here as it is on a more 'normal-looking' graph, but take the discriminant, set it equal to zero and solve for a:

b² - 4ac = 0, or 1 - 4a = 0 simplifies to a = 1/4.

Graphs in the xb plane

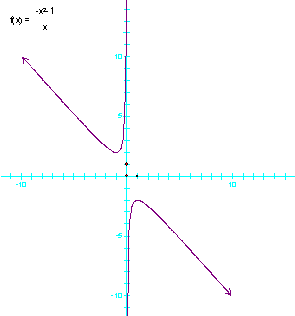
If we take any particular value of b, say b = 3, and overlay this equation on the graph we add a line parallel to the x-axis.  If it intersects the curve in the xb plane the intersection points correspond to the roots of the original equation for that value of b. We have the following graph.

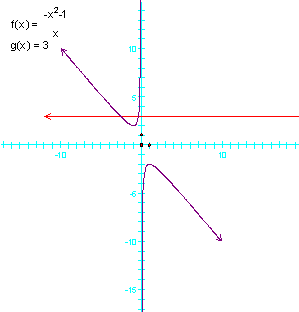
For each selected value of b, we get a horizontal line.It is clear on a single graph that we get:
 
 
 

 

Value of b	Number/type of roots
b < -2	two positive real roots
b = -2	one positive real root
-2 < b < 2	no real roots
b = 2	one real negative root
b > 2	two negative real roots

Consider the case when c = -1 rather than +1, which is the magenta graph.It is clear that there are always two distinct real roots, one positive and one negative.

 
 

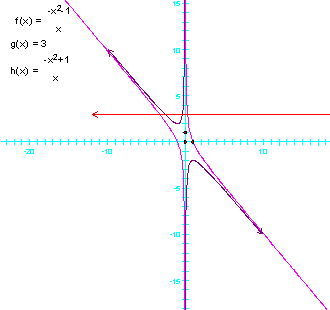
Graphs in the xc plane

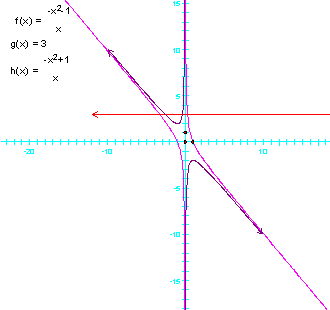
The vertex of this parabola is at -5/2, 25/4, or -2.5 and 6.25. Since this is a downward opening parabola, the roots are as follows.

 

Value of c	Number/type of roots
c > 6.25	no real roots
c = 6.25	one positive real root
0 <= c < 6.25	two real negative roots
c < 0	one real positive, one real negative root

Graphing the Locus of a Function