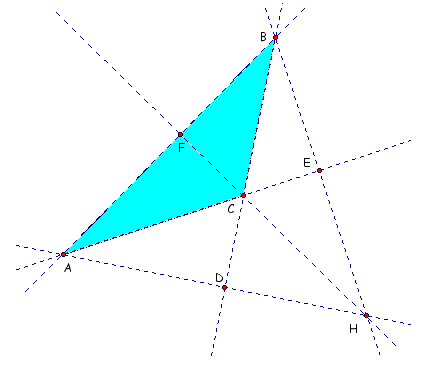

Notice that H, the Orthocenter, is no longer inside the triangle. So, insteead of being able to focus on the three triangles inside of ABC, we now need to focus on ABC, AHC, and HBC, which are inside of triangle AHB.

Considering the areas of the triangles, we can say
If we divide everything on both sides by the area of triangle ABC, we obtain 1 on the left side.
Using our sketch we can obtain the following formulas the areas of our regions.
![]()
We can use these formulas to substitute, obtaining
We can simplify this equation to obtain
In a way, this makes since, because the orthocenter was taken outside of the triangle ABC, so the altitudes are longer. It is amazing that so much of the relationship was retained.
Since the second conjecture builds on the first, it will fail in the case of an obtuse triangle, as well.
Do the Two Conjectures hold for Obtuse Triangles?
No.
Click here to return to the Altitudes and Orthocenters main page
Click here to return to Julie's main page