
Altitudes and Orthocenters
By
Audrey V. Simmons
We will be exploring the orthocenters and altitudes of any triangle ABC. By definition,.The altitude is a line drawn from a vertex and perpendicular to the opposite side. The orthocenter is the point of concurrency of all three altitudes of a triangle..
Let’s start with triangle ABC and orthocenter H. If we construct the orthocenter for triangle ABH we find it is located at vertex C. The orthocenter for triangle AHC is located at vertex B. The orthocenter for triangle BHC is located at vertex A.
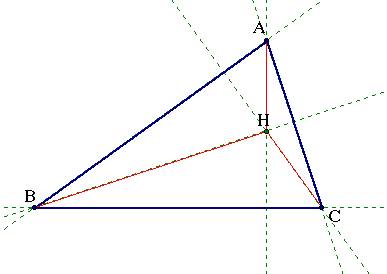
Does this relationship holds true for triangle ABC whether it is acute, obtuse, or right? Click HERE to test.
Now let’s construct the circumcenter for each of the triangles ABH, BHC, and CHA. Click HERE to see if any conjectures can be made.
What can be said about the radii of the circumcircles? Click HERE
The radii are congruent.
What would happen if one of the vertices of triangle ABC was moved to where the orthocenter (H) is located?
If point A was moved to orthocenter H, then H moves to the same location as the starting point of A. The area of the two triangles is exactly the same. This is also true for vertices B, and D.
In conclusion some conjectures are:
o The radii of each circumcircle is exactly the same.
o All the circumcircles pass through the orthocenter(H) of the original triangle ABD.
o A cube is formed by connecting the centers of the circumcircles with the orthocenter H. Click here
o The area of the overlap of the circumcenters at each vertex is bisected by the altitudes of the original triangle.
o A second area of overlap of the circumcircles is bisected by the sides of the original triangles.
o We know that the perpendicular bisector that intersects each side of the original triangle and travels through the orthocenter also intersects one of the centers of the outside circumcircles.