Assignment 2

Examining the Parabola

By Cara Haskins, Robin Kirkham, and Matt Tumlin

___________________________________________________________________

We will examine the parabola as the coefficient values change to see the effects these changes have on the various parabolas.

Examine the parabola of y = ax²+bx+c for different values of a, b, and c.

Our first step will be to:

Look at the basic parabola when a=1, b=0 and c=0

Notice that the Domain is the set of all real numbers, and the Range is all non-negative numbers. The range for this basic parabola is all non-negative numbers.

The lowest point on a parabola is called the Minimum. The minimum point for our basic parabola is (0,0).

Step 1: y=ax²+bx+c

Let b=0,c=0, and vary the values of ‘a’. Our new equation becomes y=a x².

Let us use the graphing calculator to examine the effects of using different values for a, remembering to use positive and negative values.

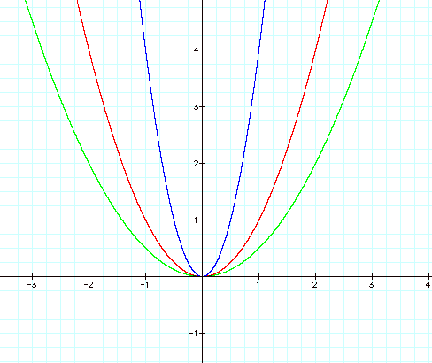
Notice that the minimum of the graphs does not change even though the value for ‘a’ was varied. When ‘a’ >1, the graph has been narrowed horizontally, resulting in a horizontal shrinking of the graph. When 0 < a < 1, the graph has now been stretched horizontally.  

This leaves us with the question, what happens when negative values are substituted for ‘a’?

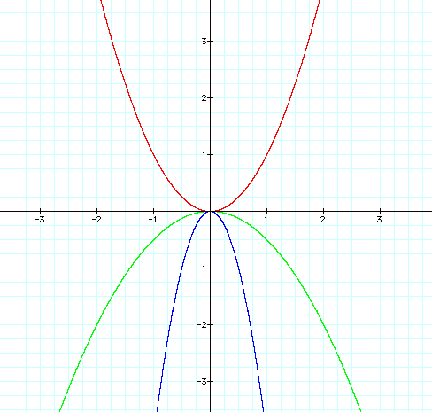
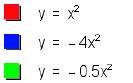
By substituting negative values for ‘a’, notice there is a reflection across the x-axis for our two graphs as well as a horizontal change of the basic parabola. The highest point on a parabola is called a Maximum. The maximum on our parabola is (0,0).

Step 2: Now we are examining the effects of variable ‘b’. Let a=1, and c=0 and change the values for b.  Our new equation is now   y = x²+bx.

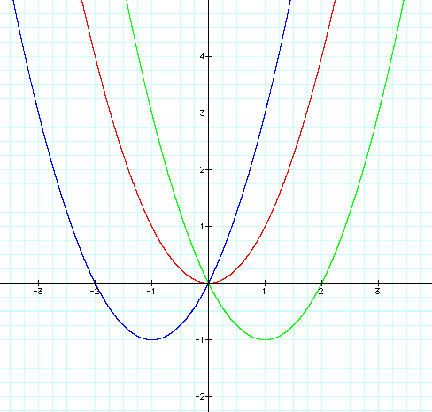
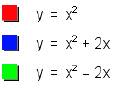
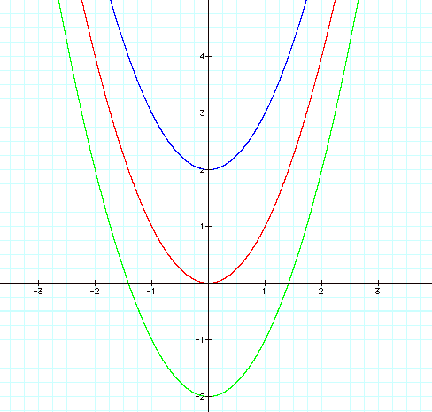
Notice that the widths of the parabolas remained the same, while the location of the minimum changed. This movement appears to be equal to the value of –c/2 both vertically and horizontally. This is investigated in our step 3 below.

Step 3: Let us again start with equation y=ax²+bx+c. Let a=1, b=0, and vary c, resulting in y = ax²+c. Note this investigation is not complete until we review the effects that variable ‘b’ might have with ‘c’.

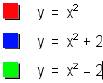
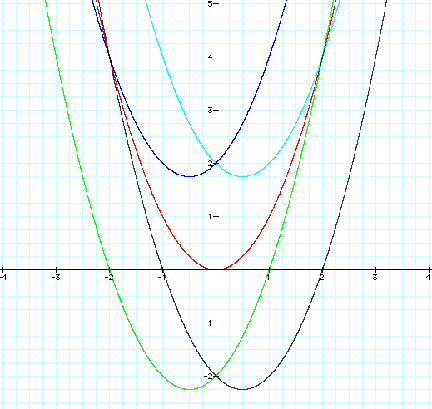
The value of variable “c” moves the parabola up or down.. When c > 0, the graph moves to the left. When c < 0,the graph moves to the right.

This vertical movement changes with respect to our minimum point. This vertical shift appears to be equal to the value of ‘c’. To be sure, let us check that with a change to variable “b” simultaneously.

This shows that the horizontal and vertical shifts are a result of both the variables ‘b’ and ‘c’. The horizontal shift still appears to be –c/2 while the vertical shift appears to be smaller than ‘c’. To be sure, let us investigate further all three variables with respect to each other.

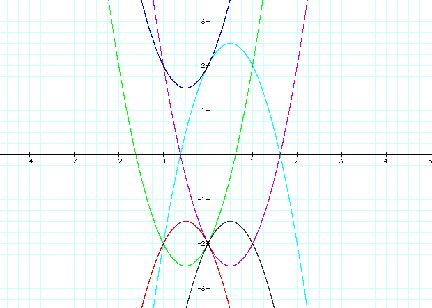
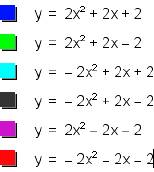
This shows us that the horizontal and vertical shifts are a result of all a, b, and c respectively. The horizontal shift turns out to be ‘-b’/2a while a vertical shift turns out to be a

This set of parabolas introduces many interesting effects. Firstly, one can see that the y=ax2+bx+c, where a, b, and c, are all positive and the similar parabola where ‘a’ is the additive inverse, one observes that these two parabolas are inverses and both shifted to opposite quadrants around the

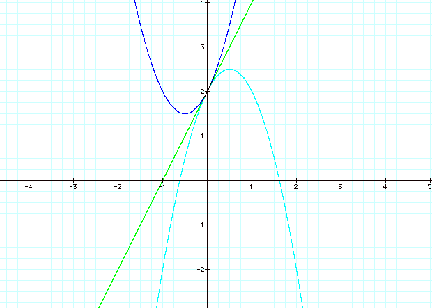
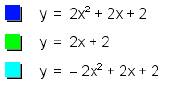
In summary, given the equation y = ax² +bx+c) the following are true:

Changes in the value of ‘a’ effects the altitude of the graph.

Changes in the value of ‘b’ effects the of the graph.

Changes in the value of ‘c’ effects in conjunction with ‘b’ together effect the phase shift of the graph.

Return to Cara’s Homepage