
____________________________________________________________
Polar
Equations
(Assignment 11)
by
Robin Kirkham, Cara Haskins , and Matt Tumlin
____________________________________________________________
Through the assistance of
Graphing Calculator 3.2, we investigate the different variances when graphing
Polar equations.
Explore the equation r = a + b
cos (k q)
such that 0 £ q £ 2 p
Since there are three
variables a, b, and k to explore, there are many cases to explore.
1. When a and b are equal, and k is an integer, this is
referred to
as the
“n-leaf rose.”
Let
us graph such that :
a
= b, k=1 (red)
a = b = 4, k=1 (green)
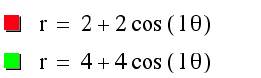
Notice that when a=b
, a and b are scalar factors for the “n-leaf rose”. Also, when k=1, the roots of
0 and a+b.
Next,
we observe the effect of k on the equation.
Below
are the graphs of a=b=2, k=3 (red)
a=b=4, k=5 (green)
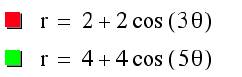
With these graphs and further exploration, we observe that k determines the number of leaves in the “n-leaf rose” figure.
2.
Let’s now look at the graph of a=2, b=8, and k=1.
![]()
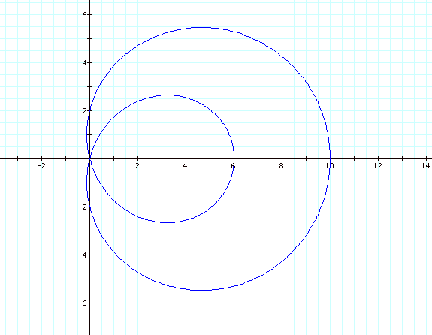
When a < b and k is
an integer, r = a + b cos (k q) have roots at 0, a+b, and a-b.
When a< b, the function traces a similar path to
the “n-leaf rose” graph, but not on the same scale.
It appears that the results provide a “k-leaf rose”.
One inner “leaf” always is at b-a on the x-axis.
3. a > b and k is an integer
Now let’s investigate the graph of a = 5, b= 2,
and k= 10.
![]()

The “leaves” are merging
towards a circle form.
The leaves come into a point
on the circle centered on the origin with the radius a-b.
The tips of the leaves work
out to a point on the circle centered at the origin with the radius a+b.
The function oscillates
between these two circles k times to produce k “leaves”.
Once k becomes large enough,
other characteristics can be explored.
Look at a=5, b= 4, and k=1000
![]()
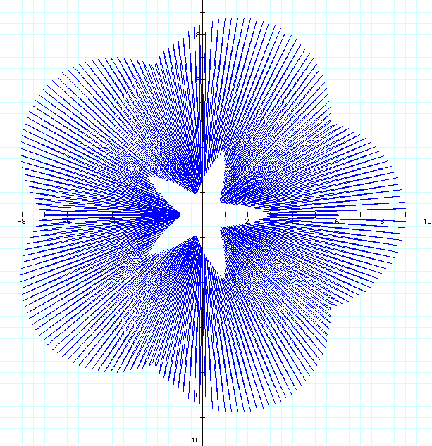
This graph has many different characteristics that could be explored. Observe that the center is not filled.
Notice that there is a five leaf rose in the center as well as the outer leaves (if you will) are also five.
This seems to be related to the “a” value.
![]()
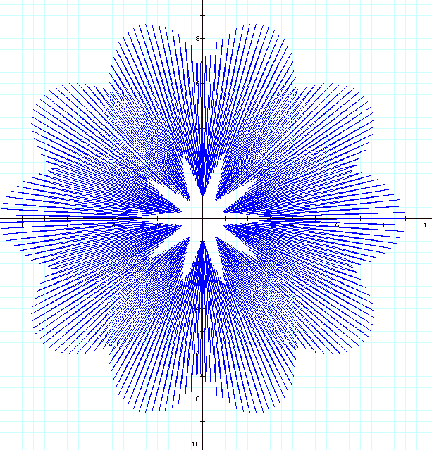
When
k=2000 is tried, notice that the internal number of leaves becomes 10.
With some further investigation it seems that the number of leaves is “1000/200 = 5”.
4) a
= b and k is NOT an integer
Let a=b=5, and k=3 in the green
and a=b=5, and k=3.4 in red

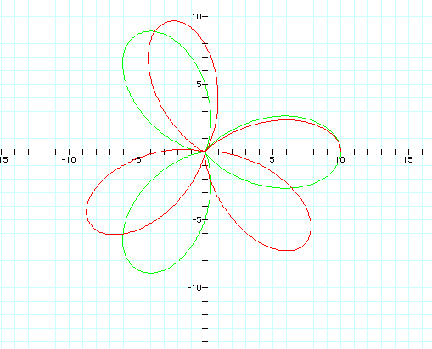
Observe that the graph is no longer continuous.
The two graphs are merging towards each other.
Thus, only when k is an integer is the graph continuous.
When k is between 3 and 4 then the number of leaves is also between three and four.
5) a < b and k is not an integer
When a
< b and k is not an integer, observe a similar transformation taking place
as we witness when a=b and k is not an integer.
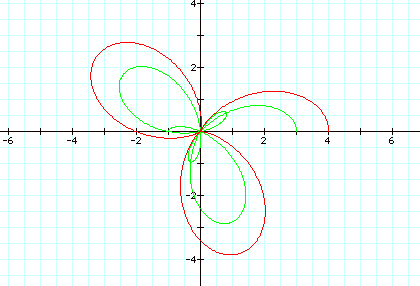
As observed before there are between 2 and 3 leaves due to k being not an integer.
6) a
> b and k is not an integer
When
a > b and k is not an integer, what do you think is observed this time?


Conclusion:
·
As
a, b, and k vary there seems to be many relationship issues that can be
discussed. The number of leaves and the relationship that both a and b have
seem to be related.
·
It
becomes more interesting when the values are no longer integers, that is when
all the changes and predictions change.
This looks like an investigation that can be
shared with high school students allowing them to draw quite a few different
and interesting conclusions as well as what has been observed.