
____________________________________________________________
Centers of a
Triangle
(Assignment 4)
by
Robin Kirkham, Matt Tumlin, Cara Haskins, and Venki Ramachandran
____________________________________________________________
Here
we show that the distance between the CENTROID, CIRCUMCENTER and the
ORTHOCENTER of any triangle always maintain a constant ratio. HG = 2GC
(key;
blue dot = H; purple dot G; green dot C . HG green line; GC orange line)
H
G
C
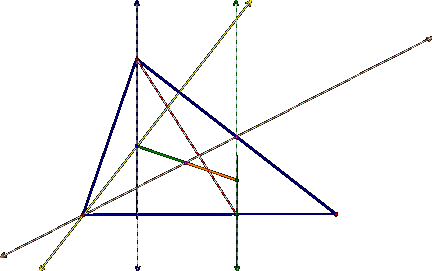
We
construct the three centers of the triangle first. The Orthocenter is the point
of intersection of the perpendiculars BH and CK. The circumcenter is the point of intersection of EF and GL.
These two lines are the perpendicular bisectors of the sides CD and BD. The Centroid is the point of
intersection of BG and CE. These are the lines drawn from the vertex to the
midpoint of the sides opposite to the vertex.
We
now need to prove that the length HG is twice the length of GC.
Consider
the two triangles HCG and CAE.
These two triangles are similar.
Proof of this similarity is easily recognized by observing that lines FE
and CK are parallel. Thus angle
ECG = angle H. Further, angle CGA = angle HGC being vertically opposite angles.
Therefore,
CG : GH as QG : GE.
We
know that G being the CENTROID of the triangle divides QE in the ration of 2:3.
Thus, QG:GE = 1:2.
Therefore,
CG : GH as 1 : 2.
If you think this was funÉ
Continued investigations of triangles:
1.
The
Centroid G is the intersection of the tree medians of a triangle. The median being a line joining the
midpoint of a side with the opposite vertex.
D
G
E
F
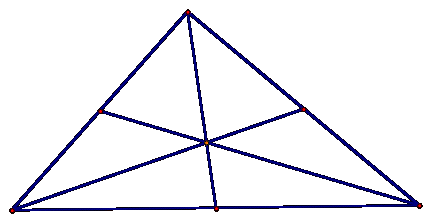
Triangle
DEF has the Centroid at G (orange
dot in center). Whatever shape the
triangle assumes, G lies within the triangle.
2.
The
Orthocenter of a triangle is the point of intersection of the altitudes dropped
from each vertex to the opposite side.
I
J
K
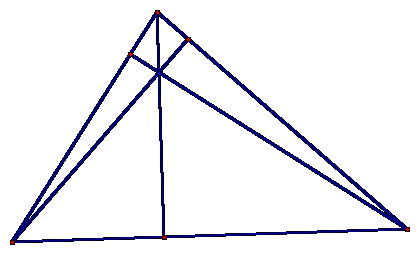
The
orthocenter could be inside or outside the triangle.
3.
The circumcenter of a triangle is the point of intersection of the
perpendicular bisectors of the sides.
A circle drawn with the circumcenter as the center would pass through
the three vertices of the triangle.
H
H
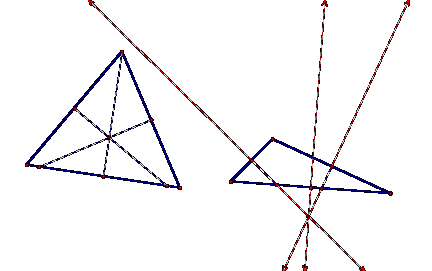
The
circumcenter C of a triangle may lie within or outside of the triangle as
demonstrated above. It can however
be observed that the locus of C will be one of the three perpendicular
bisectors.
4. The INCENTER of a triangle is the point
inside the triangle that is equidistant from the three sides. Such a point would obviously lie on the
angle bisector of the vertices.

Needless
to say, irrespective of the shape of the triangle, the in-center lies inside
the circle. Further, a circle
drawn with the In-center as a center and radius equal to the perpendicular
distance to any one side will touch the other two sides.
5.
To construct G, H, C and I for a given triangle and observe them
H
G
I
C
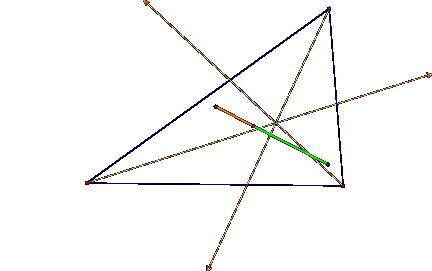
The circumcenter C, the Centroid G and the Orthocenter are always collinear. When the triangle becomes an equilateral triangle, all these four points become concurrent. Further, the ratio of the distances between C, G, and H is constant.
The
circumcenter C, the Centroid G and the Orthocenter are always collinear. When the triangle becomes an
equilateral triangle, all these four points become concurrent. Further, the ratio of the distances
between C, G, and H is constant.
6.
The INCENTER (I) of a triangle is the point on the interior of the triangle
that is equidistant from the three side.
Since a point interior to an anle that is equidistant from the two sides
of the angle lies on the angle bisetor, ten I must be on the anlge bisector of
each angle of the triangle.
I
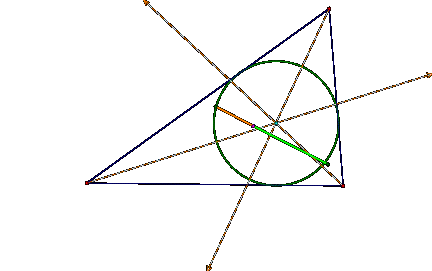
.