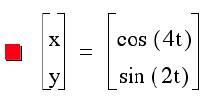Parametric
Curves
(Assignment 10)
by
J. Matt Tumlin, Cara Haskins, and Robin Kirkham
Parametric curves in the
plane x = f(t) and y = g(t)
are pairs of functions such that there are two continuous functions defined by
an ordered pair (x, y).
These equations are usually called the parametric equations of a
curve. The extent of the curve
depends on the range of t and the work with parametric equations while paying
close attention to the range of t.
In many applications, think of x and y as they vary with respect to time
ÒtÓ or the angle of rotation that some line makes from an initial location.
There are various
technology that can be used to demonstrate these curves such as : TI-81, TI-82,
TI-83, TI-85, TI-86, TI-89, Ohio state Grapher, xFunction, theorist, Graphing
Calculator 3.2, and Derive. This
investigation is performed using the Graphing Calculator 3.2.
Graph
y = sin ( t ) for 0 £ t £ 2p
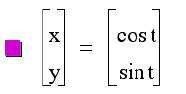
As you observe the solution
appears to be a circle with center at the origin and a radius of 1.
Further, let us observe
the parametric equations
x = cos ( at )
y = sin ( bt ) for 0 £ t £ 2p
for various aÕs and bÕs.
Let us observe some
examples:
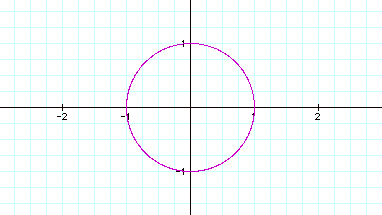
When a = b

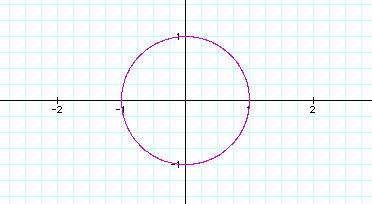
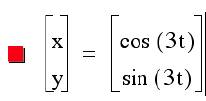
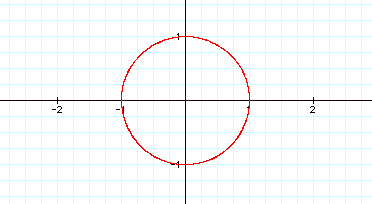
The
observation is that although the values of a and b are changed the circle
remains about the origin with a radius of 1.
LetÕs
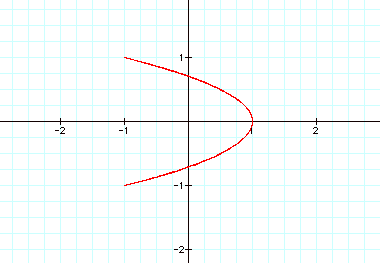
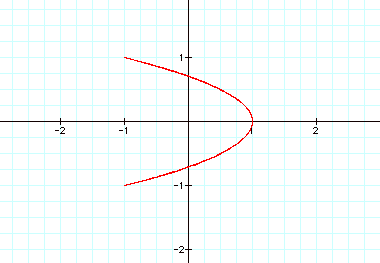
look at what happens when a = 2
and vary b in each graph such that b = 3, then b= 4, then b=5, and finally b=
6.
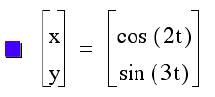
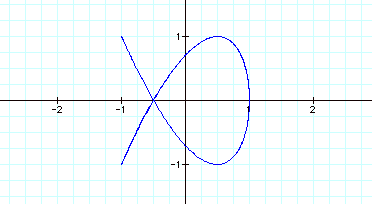
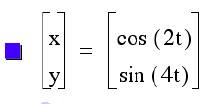

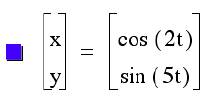

As we observe
when a=2 ten the solution is a series of curves that look like loops.
The number of
loops depends on what the value of b is set at. The number of loops = 1/2(b).
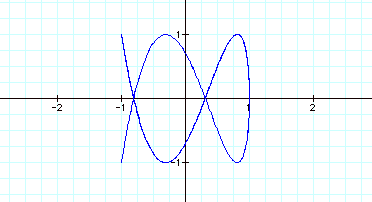
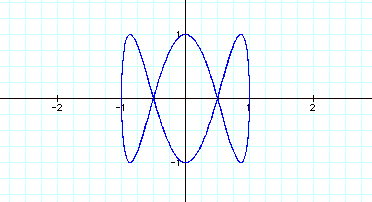
What happens when b= 2 and we vary a as we
have already done for b?

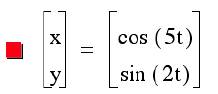
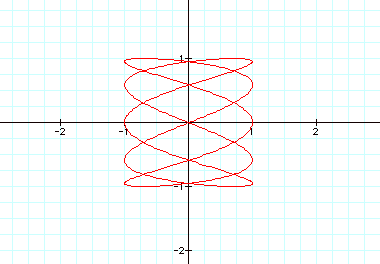
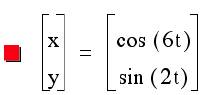
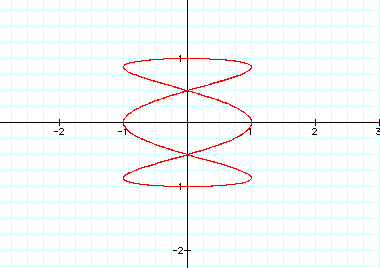
These graphs look very
different; it appears that some of the oddity is when a is an odd number versa
a is an even number.
When a is an odd number, there
is always 2 local maximums and minimums for y and there is 1 maximum and
minimum for x.
When a is an
even number, there appears to be only one maximum and minimum for y and 1/2 of
a maximums and minimums for x. When a=4, however, this is not true. Could there
be a relationship change since at that point a=2b.
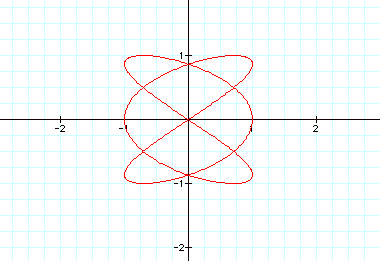
Let us observe
what happens then when a=2b.

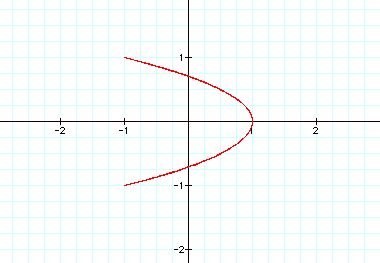

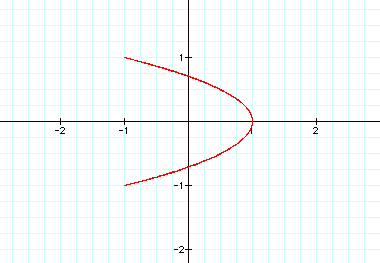
It seems true that when
a=2b the graph is always in the above shape.
Conclusion:
This investigation shows a
small sampling of what can be determined with the use of parametric curves.
There are many interesting investigations that we could continue with yet this
surely provides enough such that every new observation opens the doors to many
other variations.
Simple using the basic
curves and varying the a and b parameters to observe how the curves react
provides us with much more that can be used and expanded on in the classroom.