The diagonals of a trapezoid divide the interior region into four nonoverlapping triangles. What is the relationship between the areas of the green triangles (the triangles that share a side with the legs)? What is the relationship between the areas of the yellow triangles (the triangles that share a side with the bases)?

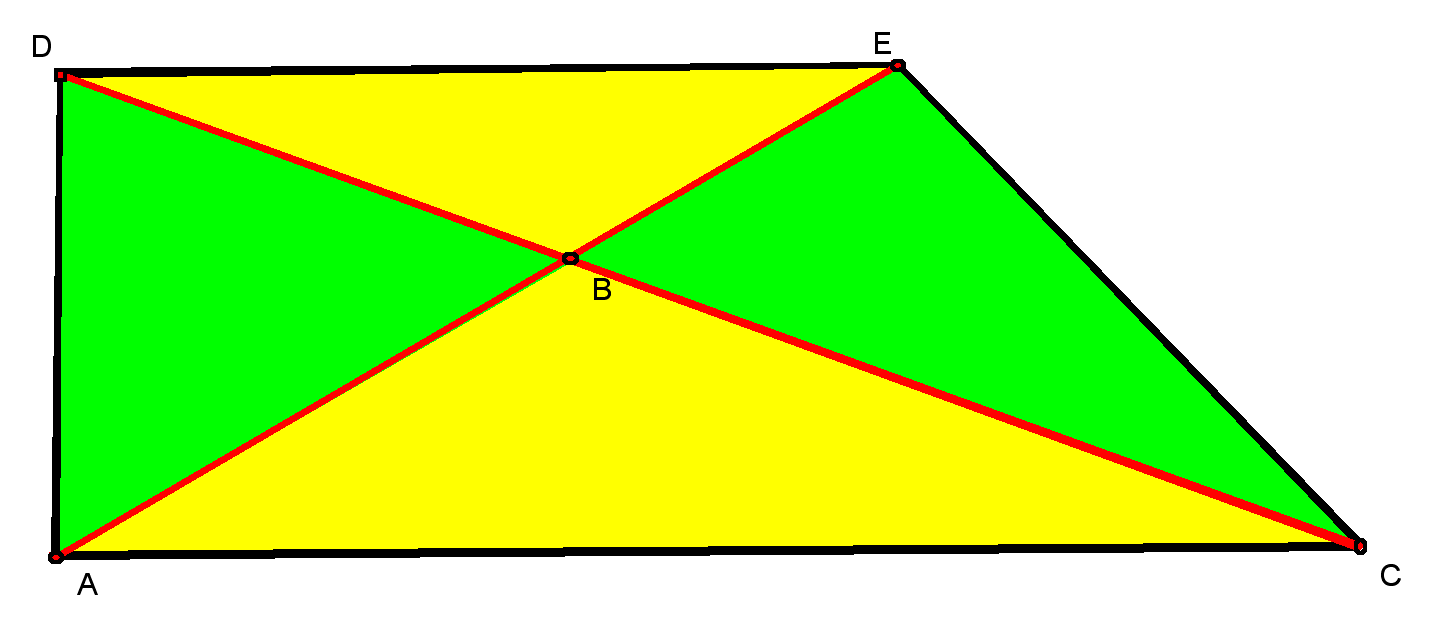
The first thing to notice is that the yellow triangles are similar. This is easy to see with the angle measures.

Regardless of the trapezoid shape the yellow triangles are similar. However, the green triangles are similar only when the trapezoid is regular. In this case, they would also be congruent. However, regardless of the shape of the trapezoid the green triangles have a one- to- one ratio between the areas.

The relationship between the yellow triangles, however, is not quite as obvious. The area of a triangle is found by 1/2 * base * height. So it makes sense that the ratio of the bases divided by the ratio of the height of the triangles is equivalent to the ratio of the top triangle to the bottom triangle.

Click here to use the GSP file.

Return to Page Bird's Emat 6690 page.