A parabola is the set of points equidistant from a line, called the directrix,
and a fixed point, called the focus. Assume the focus is not on the line.
Construct a parabola given a fixed point for the focus and a line (segment)
for the directrix.
A. Use an Action Button to generate the parabola from an animation and
trace of a constructed point.
B. Repeat 9a with a trace of the tangent line at the constructed point.
C. Use the locus command to generated the parabola from a constructed point
or the tangent line at that point.
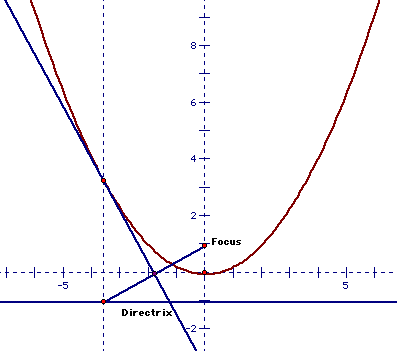
n this assignment, we will use Geometer's Sketchpad to construct a parabola.
First, draw a line on the graph. I drew a horizontal line at y=-2.
This line is called the directrix.
Next, place a fixed point on the graph. I placed mine at the origin.
This point is called the focus.
Now create a segment between the focus and the directrix. Create a midpoint on the segment.
Then, using the point on the directrix used to create the segment with the focus,
construct a perpindicular line from the directrix. The perpindicular line
should pass through the focus at only one particular point.
Construct another perpindicular line, this time from the midpoint of the segment
between the focus and the directrix. The two perpindicular lines should cross
at one point. This is the locus point of the parabola.
Finally, trace the locus point and animate the point on the directrix.
As the point on the directrix moves back and forth, the trace of the locus point will draw a parabola.