As we know the pythagorean theorem, is theorem that we can use to find a leg of a right triangle. We know that theorem as,
where,
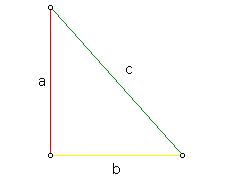
This theorem can be proven by using a right triangle and form squares around each leg. The area of square,a, plus the area of square ,b, should be equal the area of square c.
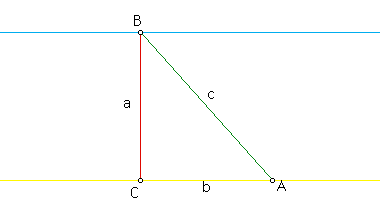
The first step in constructing square a is to extend leg b and construct a parallel line through point C and parallel to line AB.
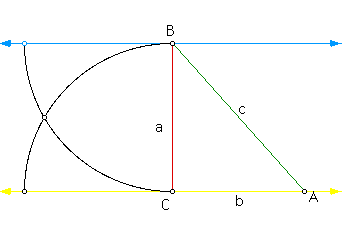
Now using point B as the center and segment a as the radius construct a circle. Where the circle and line AC meet label it D. Next use point C and the same radius a and construct a circle. Label the point E where the circle and the line B meet.
Next connect ACED and this forms your square a.
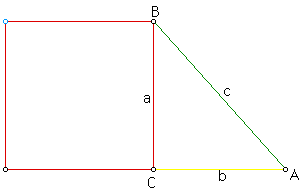
You can do the same consturction for the other two squares. Square b should look like this:
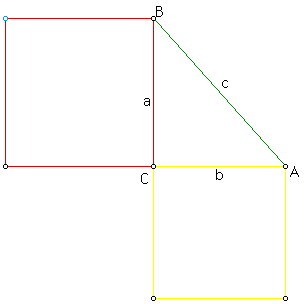
The finished 3 squares should look like this:
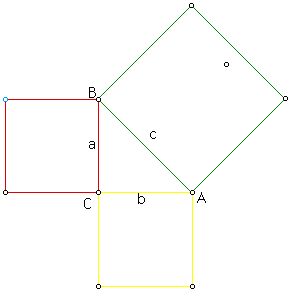
Now let's explore the areas of the squares.
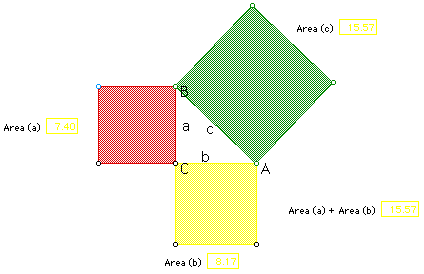
As you can see the red square plus the yellow square equals the green square.