For this investigation
I will explore the following equation:
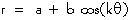 for a=b, a<b,
and a>b
for a=b, a<b,
and a>b
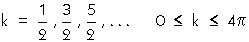
Let us begin by looking
at when a = b.
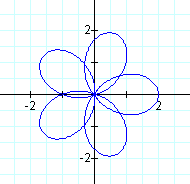
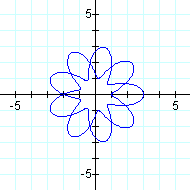
When we observe what happens
when a = b, we see that the number of petals of
our flower equals the number in the numerator of the fraction
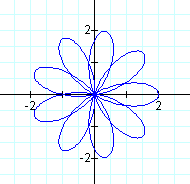
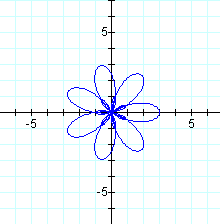
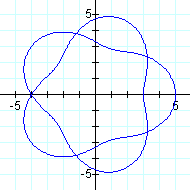
k is equal to. As we can see in the graph below, the new
flower we have created with k = 5/2
has "wider" petals than if k = 5.
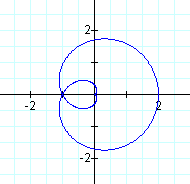
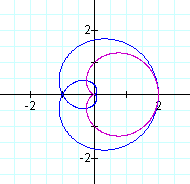
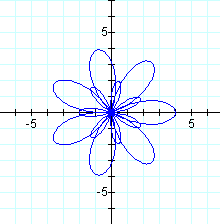
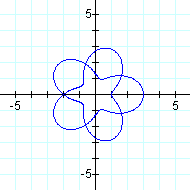
One difference that we
do see is that not only is the new flower petal wider when k = 1/2, but it also adds a loop when
compared to when k = 1.
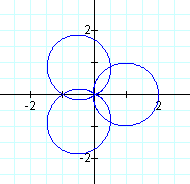
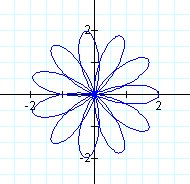
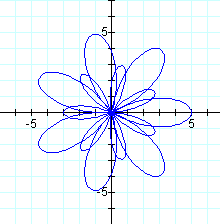
Let a > b.
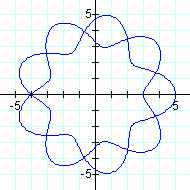
We see that just like when k was an integer, when a
> b, we create a flower with two sets of petals. Also
it once again appears that when a and b increase
so does our flower. Below is an example when k = 7/2.
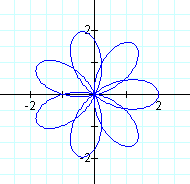
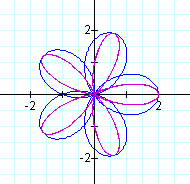
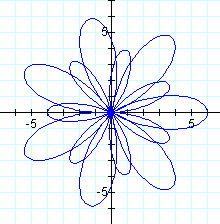
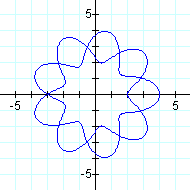
When a > b,
we see that our petals do not appear to intersect in the middle.
Instead it appears that our petal leaves connect farther away
from the center. The following graphs will illustrate this characteristics.
Look closely to see which petals are "connected" to
each other.
return to: