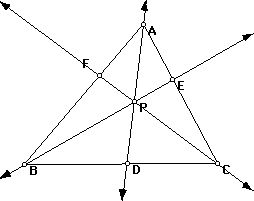
Take any triangle ABC. In order to make this proof generalizable to all of the investigations, we let point P be any point inside the triangle. Draw lines AP, BP, and CP. Let the points of intersection with the opposite sides be D, E, and F respectively.

Using the measurements of the lengths of the segments, we find that

(AF)(BD)(CE) = (FB)(DC)(EA).

In order to prove this, construct two lines through B and C that are parallel to AD.

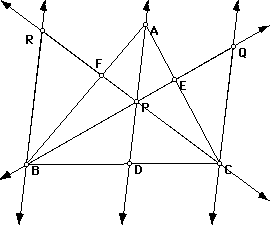
Since triangle PBD is similar to triangle QBC and triangle PCD is similar to triangle RCB
(corresponding angles are congruent), we get

Since triangle RFB is similar to triangle PFA and triangle QEC is similar to triangle PEA (alternate interior angles and vertical angles are congruent), we have


From the similar triangles we can get the following ratios:

Manipulating the first two ratios we get

Thus, using the previous ratios with this new one, we have

Simplifying the right side of the equation, we get

Return to Investigation (Identity of the point of concurrency)