Given a triangle ABC and circle O circumscribed about it, can parabolas, each tangent to all three sides be constructed? If so, is there anything noteworthy about the focal points of the parabolas?

To begin constructing one of the parabolas, extend each of the three sides of the triangle.
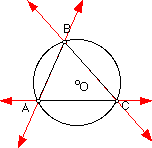
Construct a line perpendicular to side AB through point B and one perpendicular to side AC through point C. Where these two perpendiculars intersect, point D, will be the focal point of the parabola.
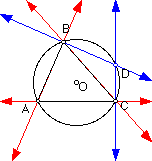
Notice that the focus is on the circumscribed circle. Construct a perpendicular to side BC through the focus point. The point of intersection between the perpendicular and side BC, point E, will be the vertex of the parabola.
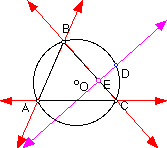
Using point E as the center and segment ED as the radius, construct a circle; and then construct the point of intersection, point F, of the circle with line ED.
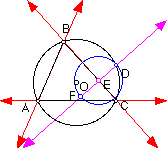
Construct the line perpendicular to line FD through point F; this line is the directrix.
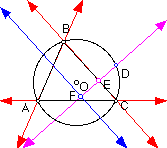
Construct parabola. Click here to see parabola .
Following the same procedure, construct parabolas on the other two sides. Click here to see all three parabolas.
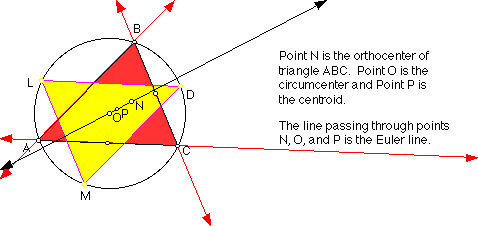
Now construct the triangle whose vertices are the three focal points.

Triangle DLM, formed by the three focal points, appears to be congruent to the original triangle ABC. Click here to check the congruency of the two triangles.
Click here to check the congruency of the the two triangles.
As you have observed, the two triangles are congruent. Now construct the Euler line of triangle ABC, the original triangle. The Euler line is the line passing through the circumcenter, centroid, and the orthocenter.
When the two triangles overlap, the three directrices and the Euler line all intersect in one point.
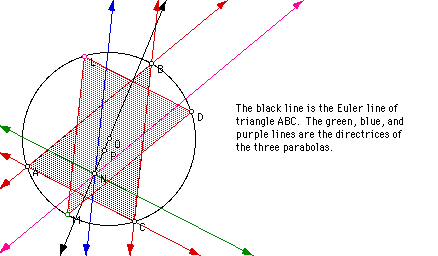
When the two triangles share a common side, the intersection of the three directrices and the Euler line lies on a point on the circle.
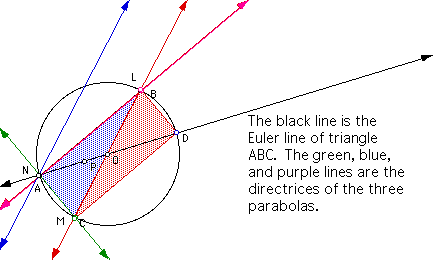
However, when the two triangles are completely separate, the three directrices do not intersect on one point.
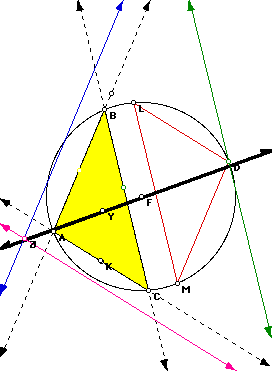
Now, construct the Euler line of triangle DLM.

Notice that the Euler line of triangle ABC is also the Euler line of triangle DLM. Even though the two triangles have only one center in common (the circumcenter), the Euler line is the same line for the two triangles. Click here to see that this is always true.
To summarize, given a triangle with a circumscribed circle, parabolas can be constructed to be tangent to each of the three sides of the triangle. If the three focal points are used as vertices, the triangle formed by them will be congruent to the original triangle. The Euler line of the original triangle is also the Euler line of the triangle formed by the three focal points.