

Perfect triangles are triangles with sides of integer length and having numerically equal integer area and perimeter.
A. Find Pythagorean Triangles (right triangles with integer sides) that are Perfect Triangles. The image to the right shows one example. It is a 6-8-10 right triangle. The area is 24 and the perimeter is 24. Are there other right triangles that are perfect triangles?
B. It is a much more difficult problem to prove there are exactly 5 perfect triangles.You might explore how this could be proven.
C. Given that there are exactly 5 right triangles, find all of them.
Observation.
If we relax the requirement that we have integer values, triangles with numerically equal perimeter and area will have an incircle with radius equal to 2. To see this consider this figure:
The area of the triangle can be seen as the sum of the areas of three triangular pieces with a common vertex at the incenter of the triangle. Thus
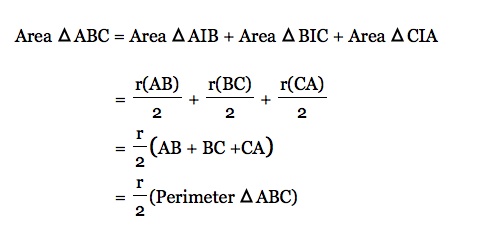
But if the perimeter is numerically equal to the area, then
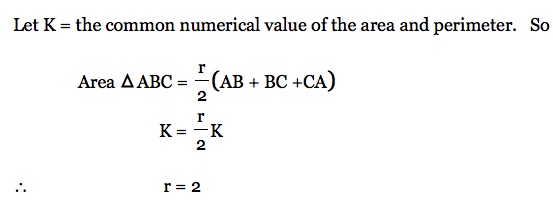
and so, r = 2. Conversely, if radius of the incircle of a triangle is 2, then the area is
which is the perimeter. Although this result is more general (applying to non-integer area and perimeter) it is useful in this problem when the values are restricted to integers.
Another Observaton:
When we have an inscribed circle, each vertex of the triangle is the same distance from the two adjacent points of tangency because the incenter is on the angle bisector and together with the two radii to the adjacent sides of the angle, congruent triangles are formed.
Note that the semi-perimeter s defined for Heron's formula will be
s = x + y + z
This notation and observation may be useful in interpreting the analysis provided in the following suggestions.
Suggestion. Will the shortest side of a perfect triangle be longer than 4 (the diameter of the circle)? Why? Try using some GSP constructions to explore this. When the shortest side is of length 4 and tangent to the circle at its midpoint, the possible other two sides would have to be parallel.
If the shortest side is of length 4 and the tangent point is other than the midpoint, then the circle of radius 2 is not inscribed in a triangle.
A GSP model can easily be constructed to explore these triangles.
Suggestion. How long can the shortest side be?
Suggestion. Can a spreadsheet be set up to search for such triangles?
Reference:
Bonsangue, Martin V.; Gannon, Gerald E.; Buchman, Ed; & Gross, Nathan. (1999). In Search of Perfect Triangles. Mathematics Teacher, 92, 56-61.
The article traces the exploration of this problem by the authors.