
The Department of Mathematics Education

Find two linear functions f(x) and g(x) such that the product
h(x) = f(x).g(x) is tangent to each.

The graphs on the same set of axes are
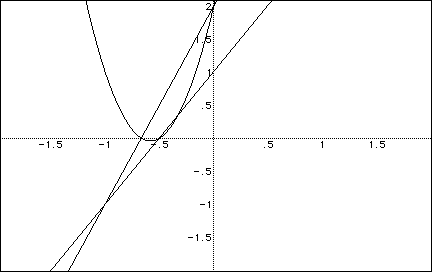
For some novices, seeing the graph of the product h(x) = (3x + 2)(2x+1)
and the graphs of the two straight lines from the factors on the same coordinate
axes provides a new experience. This particular graph has one of the two
lines "close" to being tangent to the product curve but the other
one is not close. How could the picture be changed?
One idea is to spread the two lines so that one has negative slope. Try

The graphs are
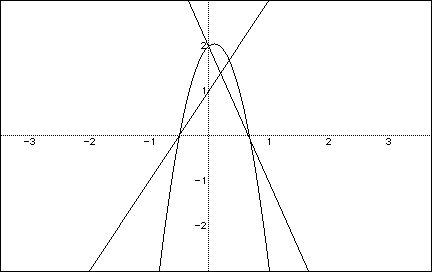
This is better? What can be observed? How can the graph of h(x) be "moved
down?" What if the graphs of f(x) and g(x) had smaller y-intercepts?
Try

The graphs are
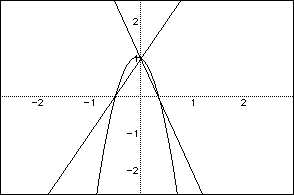
Still not too good but at least the graph of h(x) was "moved down."
Try smaller y-intercepts, such as

and result in graphs of
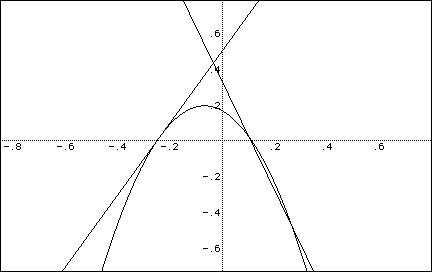
These graphs seem close, but clearly the line with negative slope is
not tangent to the graph of h(x). Looking back over the sequence of graphs
(and perhaps generating some others) the graph of h(x) always has a line
of symmetry parallel to the y-axis. It seems that the pair of tangent lines
will have to have this same symmetry. How? Try making the slopes 3 and -3.
The functions are

and the graphs are

A zoom to the right hand side of the graph with give

showing tangency has not been achieved. A zoom to the left hand side
shows a similar problem.

One could try adjusting the y-intercepts. In fact, if the y-intercepts
were equal, the y-axis would be the line of symmetry. Try

The resulting graphs are

Worse. Try

The graphs are

A zoom to the left shows

and to the right shows
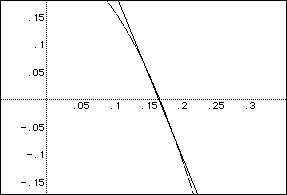
The result seems on target. It remains to confirm f(x) and g(x) each
share exactly one point in common with h(x). Again the tradition is to do
so algebraically, but it might be instructive to look at some graphs of
h(x) - f(x) and h(x) - g(x), such as the following:

Can we immediately generate graphs of other f(x), g(x), and h(x) satisfying
the conditions of the problem? Reviewing the graphs and the strategy, its
seems that the slopes of the lines can vary, the only condition being that
they are m and -m. So, a simpler case might be to let the slopes be 1 and
-1, giving

and

It is also of interest to see both of the solutions on the same graph:

Other solutions could be generated by making some other vertical line
the axis of symmetry. Indeed substituting

gives the graphs

for which the equations simplify to

Scenario Two
Try

First consider the graphs of f(x) and g(x) and try to sketch in h(x).
The graph is
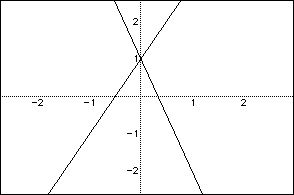
What do these lines tell you about the parabola? What points do you know
the curve will go through? Why? What causes it to open the way it does?
Now let's add the graph of the parabola and compare it with our sketch.

How is it like our sketch? How is it different? Is there anything we
should notice or consider?
It appears that all three graphs seem to intersect at 1 on the y axis. Lets
zoom for a closer look.

Maybe changing one of the functions will help with the explanation.
Consider


The three functions no longer intersect at 1 on the y-axis. However,
the changed function, f(x), does intersect the curve at its y-intercept.
When g(x) = 1 the parabola intersects f(x). Is the opposite true? Lets graph
and see.

It seems that if f(x) = 1 then h(x) = g(x) and if g(x) = 1 then h(x)
= f(x). Lets test this by trying to generate h(x) from a new f(x) and g(x).
Let

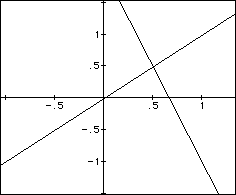
Then add the sketch h(x) and compare with . . .
 .
.In this process we seem to have also noticed that the lines and the parabola
intersect at the points when the lines cross the x-axis. Why would this
be true?
Now the goal is to get one line tangent to the parabola. The function g(x)
is close to being tangent. If we could just get the two points to slid together
then they would become one point -- the point of tangency. (If a line intersects
a parabola in exactly one point, what is true about the line?)
Since f(x) takes on a value of 1 when x = 1, then lets try to change g(x)
so that g(1) = 0. Lets see we could change the slope or change g(x)'s position
up and down.
Lets try them both.
To change slope, g(x) = -2x + 2 and test to see if g(1) = -2(1) + 2 = 0
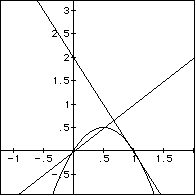

Or change position, g(x) = -3x + 3 and test g(1) = -3(1) + 3 = 0
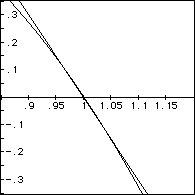
This seems to imply that f(x) and h(x) are tangent at the f(x) and h(x)'s
common root, if the function g(x) takes on the value 1 at this root. Or
in other words if f(a) = 0 and g(a) = 1 then f(x) is tangent to h(x) at
a.
What would we have to do to get both f and g tangent to h? That would mean
that when f(x) = 0, then g(x) = 1; and when g(x) = 0, then f(x) = 1. Lets
first start with an easy function for f and then try to generate a g(x)
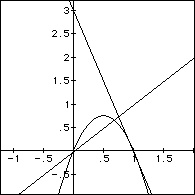
which satisfies what we want. Lets begin with f(x) = x.
Now when f(x) = 0, then g(x) should take a value of 1. In other words
if f(0) = 0, then g(0) = 1. Likewise, when g(x) = 0, f(x) should have a
value of 1. Since f(1) = 1 then g(1) = 0. We need a our linear function
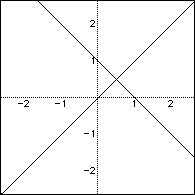
g(x) to go through (0,1) and (1,0). So our g(x) = -x +1. Lets graph it to
check.
That looks right! Now, add the graph of the product and then test it
to see if the curves are tangent.
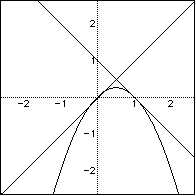
That looks good. (What is the coordinates of the vertex?) Lets zoom in
at the roots.
 and
and  .
.This seems to be a useful direction. Does it work on the previous problem?
When we left off, f(x) = x and g(x) = -3x + 3. Can we use our technique
to find a different f(x) that works for g(x) to produce h(x) = f(x).g(x)
with f(x) and g(x) each tangent to h(x)? We have the following graph.

Since g(1) = 0, then f(1) = 1 and since g(2/3) = 1 then f(2/3) = 0 will
be necessary. So f(x) contains the points (1,1) and (2/3, 0). Try g(x) =
-3x - 2.


Zoom in for a closer look.

What are the coordinates of the upper vertex of the triangle? What are
the coordinates of the vertex of the parabola? Are the lines really tangent
to the parabola? How might this be proved? Where else could the function
f(x) possibly take on the same value as h(x) if h(x) = f(x).g(x)? And how
can we interpret this on the graphs?
Summary
Many issues are hidden in these composite accounts of examination of this
problem. We still have the additional problem of writing a concise argument
of proof of the demonstration -- that the solution will always have the
two lines of slope m and -m crossing on y = 1 and the vertex of the parabola
on y = 1/2.
Each senario presents a somewhat different approach. Which would be most
helpful in finding two quadratic functions f(x) and g(x) such that their
product function h(x) = f(x).g(x) has each tangent? The following graphs
show such functions. How can they be generated?

What are some generalizations of the problem (and the solutions)?