The Golden Ratio
in Architecture
By: Christa Nathe & Kate Hobgood
Proportions and the Golden Ratio
In order to better understand the Golden Ratio, it is
helpful to have an understanding of the mathematical term proportion. The
Columbia Encyclopedia defines the term ‘proportion’ in mathematics as the
equality of two ratios. “Two pairs
of quantities a, b and c, d are in proportion if their ratios ![]() and
and ![]() are equal…” In other words, the two pairs of
quantities are in proportion if the equation
are equal…” In other words, the two pairs of
quantities are in proportion if the equation ![]() holds true. When considering the Golden Ratio, the
quantities refer to lengths of line segments.
holds true. When considering the Golden Ratio, the
quantities refer to lengths of line segments.
In these terms, the Gold Ratio is a division of a line
segment into two segments that such that the ratio of the original segment to
the larger division is equal to the ratio of the larger division to the smaller
division. Let C represent the original segment, A the larger division, and B the smaller division. It is helpful to picture the line segment as follows:

It follows that C = A + B and![]() . Therefore, A
is the geometric mean of B and C, and is commonly referred to as the Golden
Ratio.
. Therefore, A
is the geometric mean of B and C, and is commonly referred to as the Golden
Ratio.
The
Golden Ratio is also referred to as the Golden Rectangle, the Golden Section,
the Divine Proportion, and Phi (![]() ). Phi is
defined as an irrational number that has unique properties in mathematics in
which
). Phi is
defined as an irrational number that has unique properties in mathematics in
which ![]() is the solution
to a quadratic equation. Phi is
the ratio
is the solution
to a quadratic equation. Phi is
the ratio ![]() and the three
properties are as follows:
and the three
properties are as follows:
Š
![]()
Š
![]() (No other number
has this characteristic)
(No other number
has this characteristic)
Š
![]() (Phi is the
solution to a quadratic equation)
(Phi is the
solution to a quadratic equation)
When using the last
property, we can find the value for ![]() by using the
quadratic equation. There are two
solutions to this equation is
by using the
quadratic equation. There are two
solutions to this equation is![]() and 0.618033988749894848. Given these two values, we define upper case Phi or
and 0.618033988749894848. Given these two values, we define upper case Phi or ![]() = 1.618… and lower case phi = 0.618….
= 1.618… and lower case phi = 0.618….

Referring
back to the length of the line segment, C is 1.1618… times the length A, and A
is 1.1618 times the length of B.
Alternatively, B is 0.618… of A, and A is 0.618… of C. The Golden Ratio most commonly
considers ![]() as being the
positive solution, or
as being the
positive solution, or ![]() = 1.618….
= 1.618….
Construction of Golden Rectangle using GSP
The
Golden Ratio and Phi have been used in various geometrical constructions
throughout history. This
distinctive ratio can be found in the human body, nature, solar systems, DNA,
the stock market, the Bible and theology, music, artwork and design, and
architecture. Even though the
Golden Ratio is found in several aspects of culture and science, one can
experience the ratio visibly in structures of ancient and modern architecture.
The
Golden Ratio and Ancient Greek Architecture
The
Greeks were aware of the pleasing aesthetics effects of the golden ratio. Appearing in many architectural
structures, the presence of the golden ratio provided a sense of balance and
equilibrium. The geometrical
figure of the golden ratio is essentially pleasing and easy on the eye.
For instance, consider the Greek’s ancient Parthenon
located in the Akropolis in Athens, Greece. The Parthenon was built in the 5th century B.C.
when the Athenian Empire was influential and models the power and supremacy of
the empire. It was dedicated to
the Greek goddess Athena. The
temple was constructed by three architects during Iktinus, Callicrates, and
Phidias. The symbol for the golden
ratio, the Greek letter phi-![]() , was named after the sculptor Phidias. The golden ratio appears in several
constructions and layouts of the Parthenon.
, was named after the sculptor Phidias. The golden ratio appears in several
constructions and layouts of the Parthenon.
Beginning with the exterior of the building, the
dimensions of the faćade represent the perfect golden ratio. The aligning of the rectangular front
of the temple can be seen in the figure below:

Image from
www.goldennumber.net
The following diagram may provide a more clear
representation of the golden ratio in the faćade of the Parthenon:
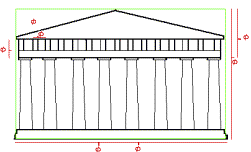
In the above figure, one can see the use of a golden
rectangle that is Phi times as wide as the height of the structure.
Notice the rectangles constructed and the highlighted
portions of the segments. Each
segment length of the rectangle follows the golden ratio, where the ratio of
the lengths of the smaller yellow segment to the larger blue segment is equal
to the ratio of the lengths of the blue segment to the whole white segment.
The golden ratio can also be found throughout the
floor plan of the Parthenon:

The floor plan area is a ![]() rectangle: the length is
rectangle: the length is ![]() times as long as
the width of the ancient temple.
Despite the numerous mathematical occurrence of the golden ratio in the
construction of the Parthenon, there are no historical records of the original
plan of the temple.
times as long as
the width of the ancient temple.
Despite the numerous mathematical occurrence of the golden ratio in the
construction of the Parthenon, there are no historical records of the original
plan of the temple.
Proof of the Golden Ratio in the Parthenon GSP
sketch
The
Golden Ratio and the Great Pyramids


Image from Culturefocus.com
The
most famous monuments of ancient Egypt are the Great Pyramids of Giza. Believed to have been constructed
around 4,600 years ago, these pyramids were built around the golden ratio, long
before the Greeks and the Parthenon.
The largest of the pyramids in Giza contains the use of phi and the
golden ratio. The golden ratio is
represented as the ratio of the length/height of the triangular face to half
the length of the square base.
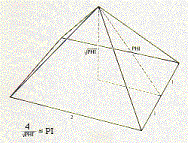
Image from Valdosta Museum website.
Š
The length of the base
of the pyramid is approximately![]() .
.
Š
The height of an
isosceles triangular face is approximately phi![]() .
.
Š
The height of the pyramid is
approximately the square root of phi![]() .
.
Š
The height can then be
found as ![]() .
.
Š
The slope of the pyramid
is very close to the golden pyramid inclination of 51° 50’.
Note that the values used in these ratios are
“approximate”. Due to the age of
the architecture, much of the material has eroded, been extracted, or altered
due to natural means.
The
Golden Ratio and Notre Dame

Image from Wikipedia
Phi
and the use of the golden ratio are found in the design of Notre Dame in Paris,
France. The Gothic Cathedral was
built beginning in the year 1163, and completed in the year 1345. Claimed as the first Christian church
in Paris, Notre Name was built upon the Roman temple of Jupiter. The west faćade of the church was
completed around the year 1200, and it is here where the presence of the use of
the golden ratios is visible. See
the image below with the highlighted segments emphasizing the ratio.

Image from GoldenNumber.net
The
Golden Ratio and the UN Building

Image from
library.thinkquest.org
The
current headquarters for the United Nations was constructed on an 18-acre piece
of land in the East side of Manhattan. Following World War II, the United
Nations was established to help intervene in future global conflicts, such as
aggression, that would in hope avoid another world war. The organization was
established and the headquarters were built under the supervision of lead
architect, Wallace K. Harrison from the United States. Although Harrison is not
typically known to use the golden ratio in his designs, a French architect
Charles E. Jeanneret was on a team of assisting architects. Charles E.
Jeanneret was known to frequently use the golden ratio in his architectural
designs. When constructing the United Nations headquarters, the team of
architects decided to use this ratio in a couple of different ways. When
looking closely at the building we observe that many of the windows in fact
have the golden ratio when comparing their width and height. The more obvious
application of the United Nations headquarters to the golden ratio is found
when looking at the width of the entire building and comparing it to the height
of every ten floors.
The Golden Ratio and the CN Tower
The
unique ratio that the Golden Ratio and Phi represent is undeniably a remarkable
concept of mathematics. The
presence of the Golden Ratio has been found in architecture dating over 4,000
years ago. From the ancient Great
Pyramids to the modern CN Tower, the aesthetically pleasing aspects of ![]() is ever present.
is ever present.
Association to the Fibonacci Sequence
Let f1, f2 , f3
, …, fn denote the values of the Fibonacci sequence. Observe
that each number of the sequence is the sum of the two numbers before it. For
example, 1 + 1 = 2 or using our notation, f1 + f2 = f3.
One can verify this method using the left column of the spreadsheet below. Interestingly enough, the Fibonacci
sequence has a surprising relation to the golden ratio. If one divides f6
by f5, the value is almost phi. The higher the number in the
sequence, the closer the ratio is to phi. Notice from the right column of the
spreadsheet that the
approximation of phi jumps around.
However, as the values of the ratio increase, the approximation begins
to gain consistent decimal place values, yielding a better approximation of
phi.
|
1 |
001/001 |
1 |
|
1 |
002/001 |
2 |
|
2 |
003/002 |
1.5 |
|
3 |
005/003 |
1.666666667 |
|
5 |
008/005 |
1.6 |
|
8 |
13/8 |
1.625 |
|
13 |
21/13 |
1.615384615 |
|
21 |
34/21 |
1.619047619 |
|
34 |
55/34 |
1.617647059 |
|
55 |
89/55 |
1.618181818 |
|
89 |
144/89 |
1.617977528 |
|
144 |
233/144 |
1.618055556 |
|
233 |
377/233 |
1.618025751 |
|
377 |
610/377 |
1.618037135 |
|
610 |
987/610 |
1.618032787 |
|
987 |
1597/987 |
1.618034448 |
|
1597 |
2584/1597 |
1.618033813 |
|
2584 |
4181/2584 |
1.618034056 |
|
4181 |
6765/4181 |
1.618033963 |
|
6765 |
10946/6765 |
1.618033999 |
|
10946 |
|
|