
I. "Completed" Investigation. To start with, here's an investigation into a rather cute relationship that involves the incenter and circumcenter of a triangle.
Construct triangle ABC and extend its internal angle bisectors (dashed in the diagram below) to intersect the circumcircle of triangle ABC (circle Z) at points L, M, and N, as shown. (Of course, the intersection of the angle bisectors is the Incenter of a triangle; Z is the circumcenter.) Find the angles of triangle LMN in terms of the angles A, B, and C.

To measure angles and make some conjectures, download this GSP file and explore!
Results & Proof: By examining the intercepted arcs of circle Z, you may see a relationship. Notice that angle A intercepts arc BC and that arc BM = arc MC (becuase the dashed ray is an angle bisector.) So the measure of angle A is half of arc BC which equals arc BM (which equals arc MC.) Angle B intercepts arc AC and arc AN = arc NC. So the measure of angle B is half of arc AC = arc AN = arc NC. Finally, the measure of angle C is half of arc ALB = arc AL = arc LB.
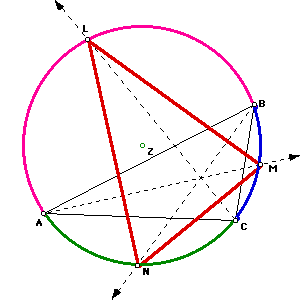
Now, the measure of angle L = half of the sum of arc NC + arc MC, so angle L = half of the sum of angles A and B. Similarly, the measure of angle M = half of the sum of arc AN + arc AL, so angle M = half of the sum of angles B and C. And finally, the measure of angle N = half of the sum of arc LB + arc BM, so angle N = half of the sum of angles C and A. Thus, angles L, M and N are the averages of two of the angles of A, B, and C. Very cute!
II. Investigation In-Progress. Now consider a relationship between the circumcenter and the orthocenter of a circle.
Construct triangle ABC and its orthocenter H. Also construct the circumcircle of triangle ABC. Reflect H over segment AB. What do you notice? Can you prove it?!
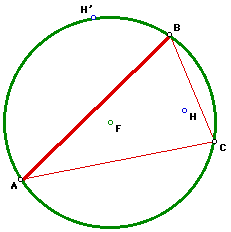
It certainly looks like H' lies on the circumcircle. So, all we need to show is that FH' = FA = FB = FC. Ah, for some reason this task is easier said than done.
Here's a summary of my progress so far. First of all, we know that C, H, and H' are collinear because line CH is perpendicular to segment AB (H is the orthocenter), and because segment HH' is perpendicular to segment AB (by definition of reflection.) So draw in CH', FA, FB, and FH'. I have also drawn BH' in the diagram on the left below and reflected F over segment AB to get F'.
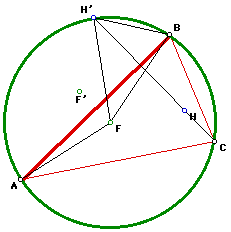

In the diagram on the right, I have drawn in triangle F'BH, which (through properties of reflection) is congruent to triangle FBH'. My goal (right now) is to show that triangle F'BH is isosceles. For some reason I have had no luck, which makes me think either I am not seeing something very obvious, or a much better approach to the problem exists. I will keep looking! In the mean time, if you'd like to experiment with the diagram on the right above, download this GSP file.
III. Investigation In-Progress. Continuing the theme of this write-up, consider this relationship that involves incenters, orthocenters, and "excenters." (Not to mention the center of the Nine-Point circle, which is itself a circumcenter of certain triangles--see Amy's Write-Up 4!)
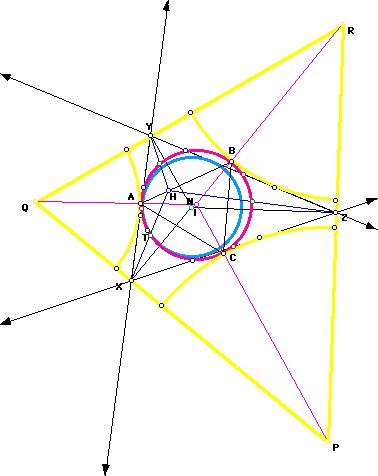
Construct triangle XYZ, its incircle, three excircles, and its Nine-Point circle. Conjecture?
Conjecture: The Nine-Point circle of a triangle appears to be tangent to the three excircles and the incircle of the triangle. That is, in the diagram below, the circle N is the Nine-Point circle of triangle XYZ, and it appears to be tangent to circle I (incircle) and circles Q, R, and P (excircles.) (Note that H is the orthocenter of triangle XYZ.) Of course, this conjecture and diagram beg for a proof...
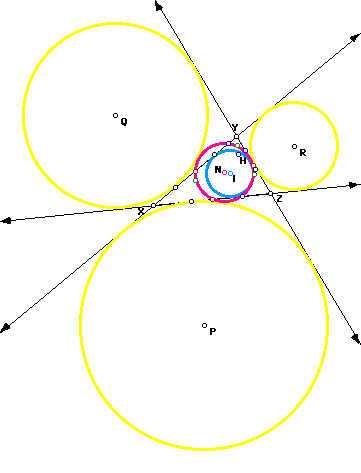
Unfortunately, I don't have one right now. I have made the
diagram below, which I think might be helpful (even if it also
might be rather busy!) Note that I have shown only the arcs of
the excircles that are intercepted by triangle PRQ.
T (black point) represents the point of tangency between the Nine-Point
circle (circle N) and the
incircle (circle I.) Points
A, B, and C are the intersection points between circles P, R,
and Q and the segments NP,
NR, and NQ. I'd like to show
Any ideas? If you'd like, download one or both of these GSP files and explore!