
EMAT6680 Assignment 4
Centers of a Triangle Proof
By Kevin Perry
Centers of a Triangle
In this discussion we will explore different centers of the triangle and prove some properties of one of the centers.† The centers we will discuss are the centroid, the orthocenter, and the circumcenter.
The Centroid
The first center of the triangle is called the centroid, often labeled G, which can also be considered the center of mass of the triangle.† In other words, if you tried to balance the triangle on a single point, the balance point would be the centroid of the circle.† The centroid is intersection of some specific construction lines of the triangle.† To get these lines, we first find the midpoints of each side of the triangle.
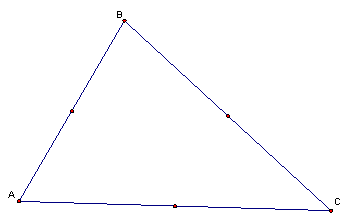
Next, we construct segments from each midpoint to the opposite vertex.
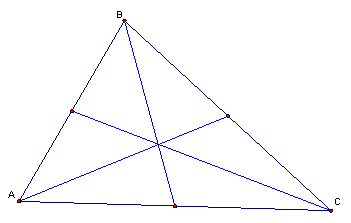
These lines intersect at a point in the middle of the triangle, and this point is called the centroid G.
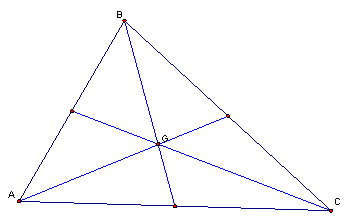 †
†
The Orthocenter
The next center of the triangle is called the orthocenter, labeled H.† It can be found in the following way.
First, construct the altitudes of the triangle from each vertex.† The altitude can be thought of as the perpendicular line to each side that passes through the opposite vertex.
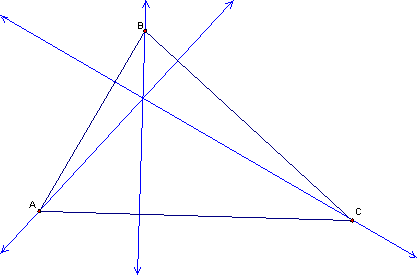

The intersection of these altitude segments is the location of the orthocenter.
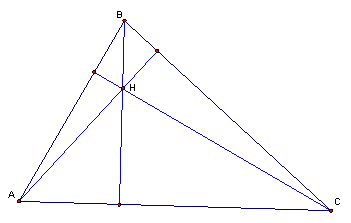 †
†
Note that the orthocenter does not have to lie inside the triangle, but even when it does lie outside the triangle, the centroid is still within the triangle.
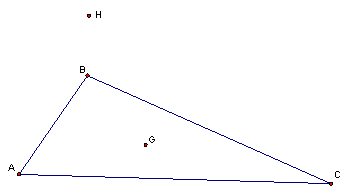
The Circumcenter
The last center of the triangle in this discussion is the circumcenter, labeled C, which is the point that represents the center of a circle that will pass through all of the vertices. In other words, it is the point that is equidistant from all three vertices.† The circumcenter is constructed in the following way.† Again, find the midpoints of the sides of the triangle.
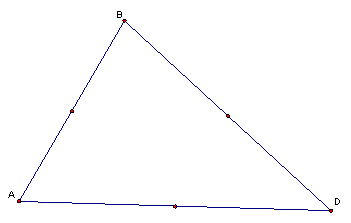
Next, construct the perpendicular line to the side that passes through the midpoint of each side.
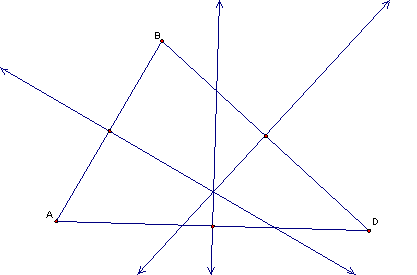
The intersection of these perpendicular lines is the circumcenter of the triangle.
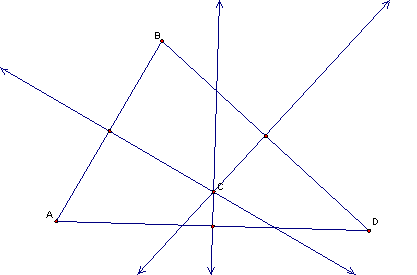 †
†
Again, with the circumcenter, this center point does not always have to lie inside the triangle.
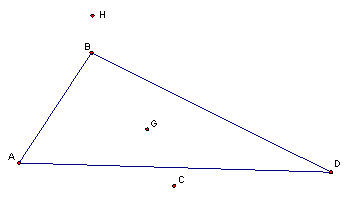
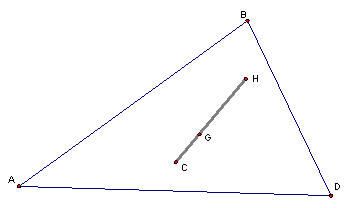
From the above diagram, the relationship between G, H, and C does not seem apparent, but it could be that the three centers are collinear.† If we construct a segment between H and C, we get
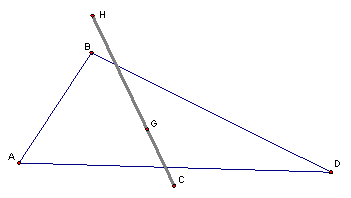
and we can see that all three points lie on the same line.† While this is not a proof, we can change the triangle and see that the points remain collinear.
Check out these
centers yourself on a GSP demonstration.
Proof of the
Circumcenter
Above, we described the circumcenter as the point that is equidistant from all three of the vertices of a triangle.† We will now prove that the circumcenter is the concurrent point of the three perpendicular bisectors of the sides of the triangle.
Prove:† The three perpendicular bisectors of the sides of a triangle are concurrent.
Letís start by first looking at two points.† If we want to find a point or set of points that are equidistant from the two points, we first connect the two points with a segment.
![]()
By definition, the midpoint of a segment is the point along the line segment that is equidistant from the two endpoints.
![]()
If we then construct a perpendicular line to the line segment that passes through the midpoint, we can call this line the perpendicular bisector of the line segment.
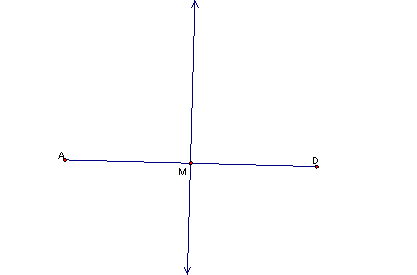
Then, we select a point on the perpendicular bisector and construct line segments from that point to each of the endpoints.

By the definition of a perpendicular line, the angles <AMP and <DMP are both 90 degree angles.† By the definition of the midpoint, the line segments AM and DM are the same length.† And because the line segment MP is common to both triangles AMP and DMP, the two triangles are congruent by the side-angle-side theorem.† Because the two triangles are congruent, the segments AP and DP are also of equal length.
Therefore, for any point P on the perpendicular bisector of the line segment AD, the point is equidistance from the two endpoints A and D.
Now, letís create a triangle ABD.
By the above conclusion, any point on the perpendicular bisector of AD is equidistant from A and D.† By similarity, any point on the perpendicular bisector of AB is equidistant from A and B.† This similarly holds for BD.
If we first take two of the perpendicular bisectors, say the one for AD and the one for AB, we have
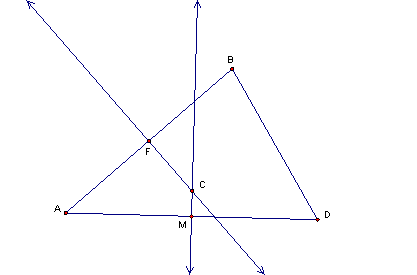
By the conclusion above, we get that the point C is equidistant from points A and D, but we also get that the point C is equidistant from the points A and B.† So if we draw the line segments, we get
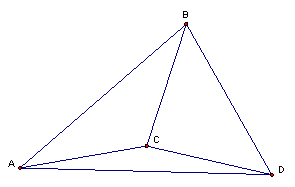
And the results above mean that AC=DC and AC=BC.† Therefore, we can say that BC=DC, or AC=BC=DC.† Because BC=DC, we know from above, that the point C must lie on the perpendicular bisector of BD.† To show this graphically
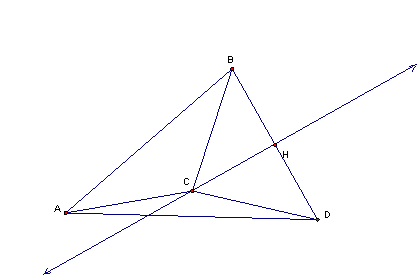
Therefore, the intersection of the three perpendicular
bisectors of the sides of the triangle is the concurrent point C.
Conclusions and
Extensions
In this discussion, we presented three special centers of a triangle.† These collinear centers are the centroid, the orthocenter, and the circumcenter.† We then proved the definition and construction of the circumcenter.
Further study could expand upon the proof of the centroid of the triangle, or discussing the relationship between these three centers, and even to find some other special centers of a triangle.