
Assignment #3
Nicole Mosteller
EMAT 6680
Some Different Ways to Examine
The Roots of a Parabola
by
James W. Wilson and Nicole Mosteller
University of Georgia
It has now become a rather standard exercise, with availble technology, to construct graphs to consider the equation
 and to overlay several graphs of
and to overlay several graphs of
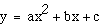 for different values of a, b, or c as the other two are held constant.
From these graphs discussion of the patterns for the roots of
for different values of a, b, or c as the other two are held constant.
From these graphs discussion of the patterns for the roots of
 can be followed.
can be followed.
For example, if we set
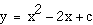 for c = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture is obtained.
for c = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture is obtained.
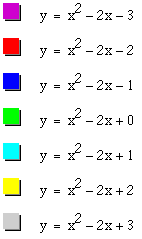
 We can discuss the "movement" of a parabola as c is changed, and with this movement,
we can discuss the changes in the roots for the general equation
We can discuss the "movement" of a parabola as c is changed, and with this movement,
we can discuss the changes in the roots for the general equation
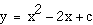 .
For c < 0, our equation will have two real roots - one positive and one negative.
For c = 0, our equation also has two real roots - one positive and one the origin.
For 0 < c < 1, our equation will have two positive real roots.
For c = 1, our equation is tangent to the x-axix at the its vertex, and
our equation has only one root at this point of tangency, (1,0).
And, for c >1, our equation will have no roots.
.
For c < 0, our equation will have two real roots - one positive and one negative.
For c = 0, our equation also has two real roots - one positive and one the origin.
For 0 < c < 1, our equation will have two positive real roots.
For c = 1, our equation is tangent to the x-axix at the its vertex, and
our equation has only one root at this point of tangency, (1,0).
And, for c >1, our equation will have no roots.
This method has proven successful when investigating roots of parabolas, but even with access to technology
this method can be quite time consuming, and we are still plagued with a bit of guess-work.
With the assistance of technology, our investigation into the effect of a, b, and c on the
roots of parabolas can be simplified by investigating just the root equation
 but in the x-a plane, the x-b plane, or the x-c plane.
Below shows an investigation into these various planes.
but in the x-a plane, the x-b plane, or the x-c plane.
Below shows an investigation into these various planes.
In the x-a plane, let's investigate the roots of the parabola given by the quadratic equation
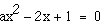 .
In the x-a plane, the a-coordinate shows the different values for the leading coefficient for a quadratic, and
the x-coordinate gives the root at each of the values of a chosen. For example, see Figure 1 below.
.
In the x-a plane, the a-coordinate shows the different values for the leading coefficient for a quadratic, and
the x-coordinate gives the root at each of the values of a chosen. For example, see Figure 1 below.
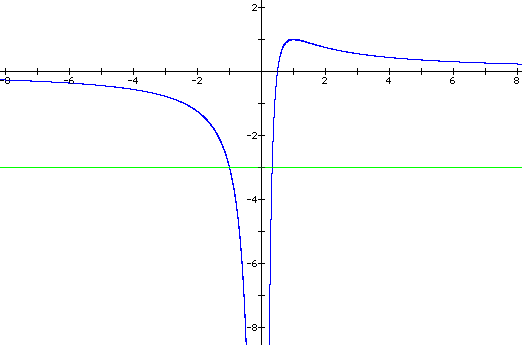 Figure 1:
Figure 1:
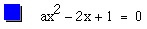
 From this picture, we can see not only the number of roots for each case of a, we can also
find the actual value of the roots by observing the intersection of the roots equation with a equal to some value.
When a = -3, we see two roots - one at -1 and the other at 1/3.
Also from this graph we can make the same generalizations as we did from a multi-graph representation.
For a < 0, there are two real roots - one positive and one negative.
For a = 0, there is only one real root.*
(*Remember when a = 0, our equation becomes linear.)
For 0 < a < 1, there are two real roots - both positive.
For a = 1, there is only one real root where the parabola is tangent to the x-axis.
For a > 1, there are no real roots.
From this picture, we can see not only the number of roots for each case of a, we can also
find the actual value of the roots by observing the intersection of the roots equation with a equal to some value.
When a = -3, we see two roots - one at -1 and the other at 1/3.
Also from this graph we can make the same generalizations as we did from a multi-graph representation.
For a < 0, there are two real roots - one positive and one negative.
For a = 0, there is only one real root.*
(*Remember when a = 0, our equation becomes linear.)
For 0 < a < 1, there are two real roots - both positive.
For a = 1, there is only one real root where the parabola is tangent to the x-axis.
For a > 1, there are no real roots.
In the x-b plane, let's investigate the roots of the parabola given by the quadratic equation
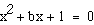 .
In the x-b plane, the b-coordinate shows the different values for the coefficient of the linear term for a quadratic,
and the x-coordinate gives the root at each of the values of b chosen. For example, see Figure 2 below.
.
In the x-b plane, the b-coordinate shows the different values for the coefficient of the linear term for a quadratic,
and the x-coordinate gives the root at each of the values of b chosen. For example, see Figure 2 below.
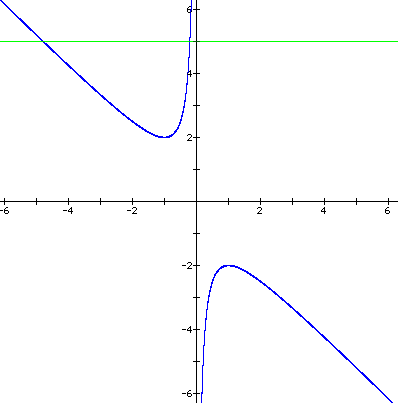 Figure 2:
Figure 2:
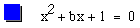
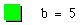 From this graph, again we can see both the number of roots for each case of b
as well as the values of each of the roots.
When b = 5, we see two negative, real roots - both irrational - near -5 and near 0.*
(*The decimal values are -4.79129 and -0.208712.)
Also from this graph we can make the same generalizations as we did from a multi-graph representation.
For b < -2, there are two real roots - both positive.
For b = -2, there is only one real root.
This positvie root is were the parabola is tangent to the x-axis.
For -2 < b < 2, there are no real roots.
For b = 2, there is only one real root where the parabola is tangent to the x axis again.
For b >2, there are two real roots - both negative.
From this graph, again we can see both the number of roots for each case of b
as well as the values of each of the roots.
When b = 5, we see two negative, real roots - both irrational - near -5 and near 0.*
(*The decimal values are -4.79129 and -0.208712.)
Also from this graph we can make the same generalizations as we did from a multi-graph representation.
For b < -2, there are two real roots - both positive.
For b = -2, there is only one real root.
This positvie root is were the parabola is tangent to the x-axis.
For -2 < b < 2, there are no real roots.
For b = 2, there is only one real root where the parabola is tangent to the x axis again.
For b >2, there are two real roots - both negative.
The original multi-graph representation was to observe the roots for the equation
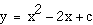 .
In the x-c plane, let's investigate the roots of the parabola given by the quadratic equation
.
In the x-c plane, let's investigate the roots of the parabola given by the quadratic equation
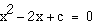 .
We should expect the same results for roots as we anticipated from the original set of graphs.
Remember, in the x-c plane, the c-coordinate shows the different values for the constant for a quadratic,
and the x-coordinate gives the root at each of the values of c chosen.
.
We should expect the same results for roots as we anticipated from the original set of graphs.
Remember, in the x-c plane, the c-coordinate shows the different values for the constant for a quadratic,
and the x-coordinate gives the root at each of the values of c chosen.
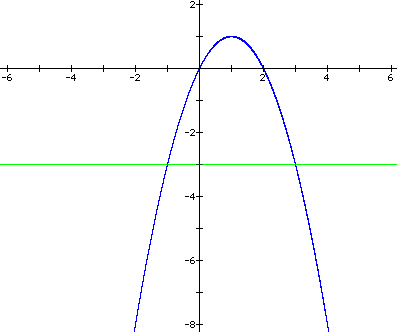 Figure 3:
Figure 3:
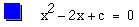
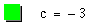 Comparing our results for c = -3, notice that we have two roots -
one root at -1 and the other at 3.
In addition to finding the roots at c = -3, we can now find the roots at any given value of c.
Notice that can make the same generalizations as we did from a multi-graph representation.
For c < 0, our equation will have two real roots - one positive and one negative.
For c = 0, our equation also has two real roots - one positive and one the origin.
For 0 < c < 1, our equation will have two positive real roots.
For c = 1, our equation is tangent to the x-axix at the its vertex, and
our equation has only one root at this point of tangency, (1,0).
And, for c >1, our equation will have no roots.
Comparing our results for c = -3, notice that we have two roots -
one root at -1 and the other at 3.
In addition to finding the roots at c = -3, we can now find the roots at any given value of c.
Notice that can make the same generalizations as we did from a multi-graph representation.
For c < 0, our equation will have two real roots - one positive and one negative.
For c = 0, our equation also has two real roots - one positive and one the origin.
For 0 < c < 1, our equation will have two positive real roots.
For c = 1, our equation is tangent to the x-axix at the its vertex, and
our equation has only one root at this point of tangency, (1,0).
And, for c >1, our equation will have no roots.
Using the multi-graph representation for parabolas certainly give our students an idea
of the effect of the values of a, b, and c in the equation for parabolas
Finding different representations, like the presentations seen in the x-a plane, x-b plane,
and x-c plane serve to enrich our students' mathematics experience
as well as to cement the idea of the roots of parabolas.
If time allows, an extension worth noting is the investigation of the x-a plane, x-b plane, and x-c plane
and the interpretation of the locus of the vertices of the parabolas from these graphs.
Return to Nicole's Page

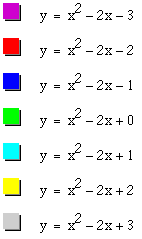
 We can discuss the "movement" of a parabola as c is changed, and with this movement,
we can discuss the changes in the roots for the general equation
We can discuss the "movement" of a parabola as c is changed, and with this movement,
we can discuss the changes in the roots for the general equation
 Figure 1:
Figure 1:
 Figure 2:
Figure 2:
 Figure 3:
Figure 3: