

The investigation of this quadratic equation begins by looking
at differing values of a. As we look at what values we would like
to substitute in for a one immediate possibility that comes to mind
might be zero. However, substituting in the value of zero into a
changes our quadratic equation into a first order linear equation: y = x
+ 2.
Can we predict at this moment whether or not it will be important to furthur
investigations? Let's build our explorations on the same axes as y = x +
2, therefore allowing us to observe any relationships that may exist between
the linear and quadratic function.

One of the first observations we should make is that the
y-intercept of the graph is (0,2). The line also seems to have a slope of
1.
As we begin to investigate the shape and position of our graph for various
values of a, we should begin with values that are elementary in nature so
we can recognize any relationships. My first choice for the value of a
will be 1 and -1.
![]()

![]()

If we place each equation on the same set of axes, we will
begin to see their relationship to each other and the linear function y
= x + 2.

We should observe that the graphs share a common point
at the coordinate (0,2) which is the y-intercept. Will this relationship
hold for any value of a?

A closer look:

It is easy to see that the graph y = x +2 helps to define
the point through which the quadratic equations pass through.

At the value a = 1, we can see that our parabola passes
through the common point of (0,2). We should also observe that the vertex
of the parabola is shifted left of the y-axis and is actually below the
y-coordinate of 2.

In this graph, we substituted the value of 2 in for the
value of a. The parabola continues to share the common point of (0,2), yet
its vertex has moved closer to the y-intercept. The parabola itself seems
to be narrower about its axis of symmetry.

As we continue to increase the value of a, the parabola
continues to become more narrow.


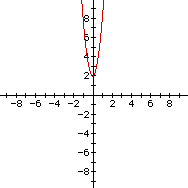


Once again our parabola passes through the point (0,2).
It seems as if in this graph the vertex of the parabola is "sitting"
on the y-intercept giving us the value of the parabola's minimum as (0,2).
Let's investigate more closely what affect a has on its graph by substituting
values that are less than 1.
The following is a graph of our equation with the values of 1/2, 1/3, 1/5, 1/7, and 1/10 substituted in for a.

Using the graphs, lets make some suppositions about the affect a has on the equation. When a was substituted with a value that is greater than 1, our parabola became narrower. When a was substituted with a value less than 1, the parabola became wider. This is known as the 'dilation' of the parabola.
It is also easy to observe that the vertex of the parabola moves when the value of a is changed. In order to show this connection we need to look at the derivative of the parabola when it is set to zero. The reason we need to set the derivative equal to zero is that the derivative will give us the slope of the line tangent to our original equation. At the vertex of our parabola that slope will be equal to zero (a horizontal line).
Let's look at our equation and its derivative:
If we set this to zero and solve for x, our value becomes
As you can see the vertex of the parabola is directly related to the value of a.