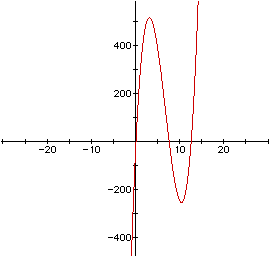
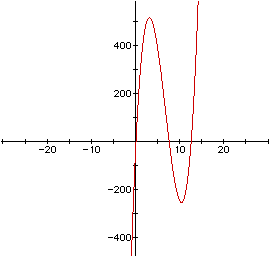
From the graph of this equation, we notice that the maximum occurs somewhere
between 0 and 5 and the maximum seems to be greater than 500 cu. in. We
also notice that there are two x values that produce a volume of 400 cu.
in.
Let's first find the x values that produce a volume of 400 cu. in. We do
this by setting y=400 and solving for x.
Now, let's graph the last equation.
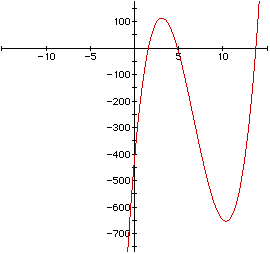
From this graph, we notice that the x's that produce a volume of 400
cu. in. are approximately 1.5 and 4.8. If we zoom in we get the following
graph.
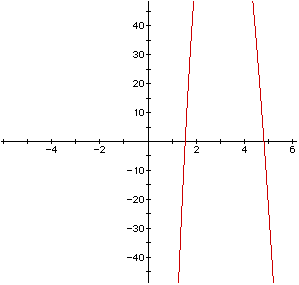
The x-intercepts or the values of x that produce a volume of 400 in.3
are seen more clearly. From this graph, one can tell that when y=400, x=1.5
and x=4.8.
Now we need to find the x values that will produce the maximum volume. To
do this, we need to take the first derivative of our volume function. Our
function is
The derivative is
Now, we need to graph this equation. The x-intercepts or roots will be
the x values that will produce the maximum and minimum volume.
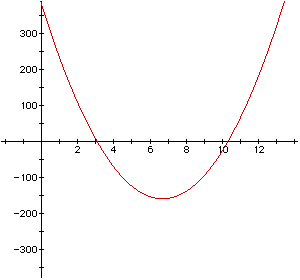
Examining this, we see that the x-intercept that we are interested in
is x=3.04. Once we solve for x in the above equation, we see that x=3.034251.
When we plug this value for x into our volume equation, we get V(x)=513
cu. in.
Now, lets use GSP to investigate the volume. We first need to construct
our box. We get the following construction.

In the above diagram, the yellow portion of the box is the bottom and the blue portions are the sides. The red areas designate the size of the squares that are removed from the piece of cardboard to form the box. The size of the red area also tells you the height of the box. The larger the red area is the deeper the box. The smaller the red area is the flatter the box. By using this GSP diagram, students will be able to explore how the volume changes when you change the height of the box. We can change the height of the box by changing the length of the line segment EF. This diagram does not have the dimensions that our real box has (i.e. It is not 15 by 25), but it does have the 3 to 5 ratio that our original box has. Thus, one is able to visualize what will happen to the volume when we change the size of the square that is to be cut off from each corner. While performing my investigations of volume for different types of boxes, something interesting happened; there are two different types of boxes that will produce a box with zero volume and there is also a point in which the volume would maximize and then would began to decrease again. Thus there are two possibilities for any volume except one the maximum. The following diagrams show these patterns.
In this diagram and the one below, the volume approaches zero, but the x values are very different.
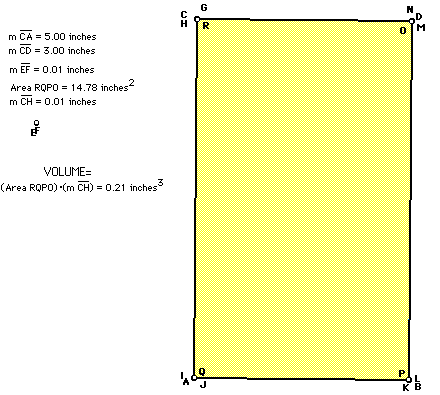
You can see this same pattern for any desired volume, except for the
maximum volume. The maximum for this particular box is shown in the first
diagram presented here. It is 4.10 in.3. One will also notice that since
the maximum volume is greater than 400 in.3 there are two x values that
will produce a volume of 400 in.3.
We are finally asked to solve this problem by using a spreadsheet. I used
the spreadsheet that is available in Claris Works 4.0. We are going to use
the same equations that we used when we solved this problem using Algebra
Expresser. First, we are going to found out what x should be in order to
have a volume of 400 cu. in. First, we need to locate all of our zeros.
We do this by using the following spreadsheet.
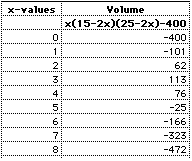
We don't have to test all values of x because we know that x will fall
between 0 and 7.5 since the length of our smallest side is 15 in. From observing
this spreadsheet, one notices that the zeros lie between (1,2) and (4,5).
From our work with Algebra Expresser, we know that the roots lie somewhere
around 1.5 and 4.8. Now let's use our spreadsheet and explore these roots
more closely.
First, lets look at the root that lies around 1.5. From our work in Algebra
Expresser, we know that the zero is somewhere between 1.52 and 1.53. Because
of this previous knowledge, I started with the x-value 1.519 and added .001
until I found the root. We get the following spreadsheet.
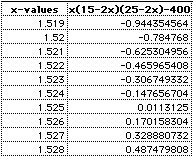
As you can see from the above table, the root is approximately 1.525.
Therefore, when x is approximately 1.525, the volume will be approximately
400 in.3.
Now, we need to find the other x-value that produces a volume of 400 in.3.
From our first spreadsheet and from our work with Algebra Expresser, we
know that the other value lies somewhere between 4.78 and 4.8. I used the
following spreadsheet to find a more exact answer. I started with 4.78 and
added .001 until I reached 4.8.

From this table, you notice that the other x-value that produces a volume
of 400 cu. in. is approximately 4.793.
Now, we need to find the x-value that produces the maximum volume. We will
use the following volume formula to find this x-value. V(x)=x(15-2x)(25-2x)
As in our first spreadsheet, we are only concerned with the x-values that
range from 0 to 7.5 because the maximum length of the smallest side is 7.5.
We get the following spreadsheet when we start with 0 and increment by 1
until we reach 8.
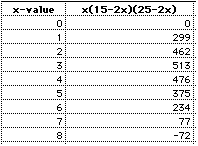
From examining this table, you notice that when x=3 the volume is at
a maximum of 513 cu. in.
From all of our investigations, we found that when the length of the squares
being cut off are 1.525 or 4.793 then the volume is 400 cu. in. and when
x=3 then the volume is at its maximum. We have investigated this problem
using several distinct methods with each method given us a better understanding
of the problem.