HistoryHistory

---

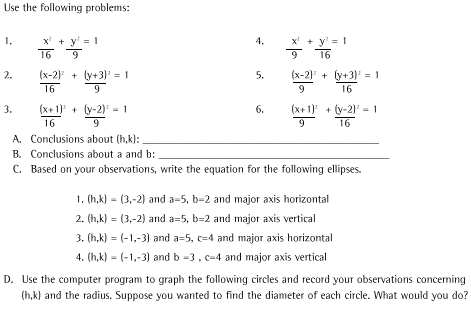
Lesson I: Definition and Basic Properties of Ellipse

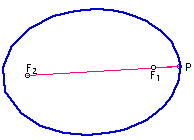
Definition: Ellipse is commonly defined as the locus of points P such that the sum of the distances from P to two fixed points F1, F2 (called foci) are constant. That is, distance[P,F1] + distance[P,F2] = 2 a, where a is a positive constant.

GSP File An interactive java construction	GSP File
Figure 1. Two constructions of an ellipse Movie Clip

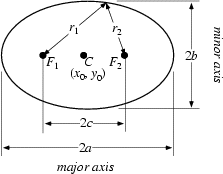
Before students spend several minutes on observing and playing with the ellipse, introduce two important lines (axes) for an ellipse, major and minor axes, and the vertexes of an ellipse (See Figure 2).

Vertexes of the ellipse are defined as the intersections of the ellipse and a line passing through foci. The distance
between the vertexes are called major axis or focal axis. A line passing the center and perpendicular to the major
axis is the minor axis.

Figure 2. Vertexes (V1 and V2), Major and Minor Axes of an Ellipse

Click here to investigate parabolas on an interactive java applet (If you do not have GSP software on your computer, it's an perfect opportunity to take a look at.)

Click here for an interactive java applet game which is about to find the foci of an ellipse.

Click here for an interactive java applet toy to construct ellipses with a tool called "Ellipsograph".

Click here for a java applet to investigate relative properties of two congruent ellipses.

Return to the lesson plans list

Lesson II: Introduction to the algebraic representation of an ellipse

Analytic Equation of an Ellipse

Before introducing a general equation (i.e. the analytic, polar or parametric form) of an ellipse, it is important to understand where this equation comes from. Thus, before giving a formal equation of an ellipse, deal with the following construction of the equation with referring to the notation on the Figure 3:

First of all notice that

(Why? Let the students think about the picture below!)

Let an ellipse lie along the x-Axis and find the equation of the Figure 3 where F1 and F2 are at (-c,0) and (c,0). In Cartesian Coordinates,

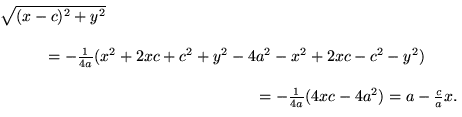
Since

This makes the equation in the simplest form:

If, instead of being centered at (0, 0), the Center of the ellipse is at , equation becomes

In class explorations:

Return to the lesson plans list

Lesson III: Polar and Parametric Equations of an Ellipse

As can be seen from the Cartesian Equation for the ellipse, the curve can also be given by a simple parametric form analogous to that of a circle, but with the x and y coordinates having different scalings,

---

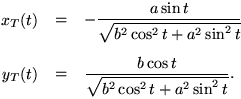
In Polar Coordinates, the Anglemeasured from the center of the ellipse is called the Eccentric Angle. Writing for the distance of a point from the ellipse center, the equation in Polar Coordinates is just given by the usual

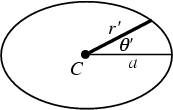
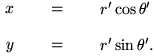
Here, the coordinates and are written with primes to distinguish them from the more common polar coordinates for an ellipse which are centered on a focus. Plugging the polar equations into the Cartesian equation (9) and solving for gives

Return to the lesson plans list

Lesson IV: Circumference and Area of an Ellipse

Circumference: The circumference of an ellipse with major axis radius, a, and minor axis radius, b, is given (approximately) by

Area: The area of an ellipse with major axis radius, a, and minor axis radius, b, is given by

The Area of an arbitrary ellipse given by the Quadratic Equation

Return to the lesson plans list

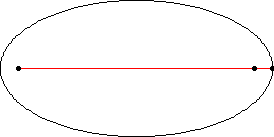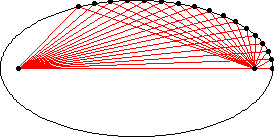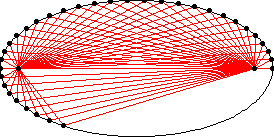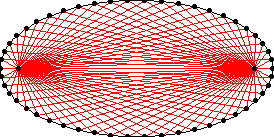
Lesson V: Reflective (Optical) Property of an Ellipse

In an ellipse, lightrays from one focus will reflect to the ther focus (See Figure 4).

Figure 4. Reflective property of an ellipse GSP File Movie Clip

Click here for an interactive java applet to investigate reflection (optical) property of an ellipse.

Students' Investigations

Investigation 1. The locus of points for a fixed point on the coming ray (from F2 to P) is an ellipse (See Figure 5). Why? What kind of evidences do we have? What properties does it have (like where is its foci--hint: one of them is F2)?

Figure 5. GSP File

---

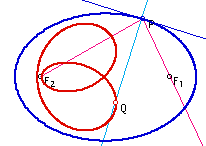
Investigation 2. What is the locus of points for a fixed point Q on the reflected ray (from P to F1) is an ellipse? (See Figure 6 for various locus which depends on the location of Q). Describe the properties of this curve?

Figure 6. GSP File

---

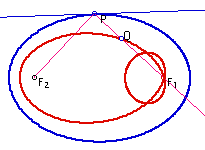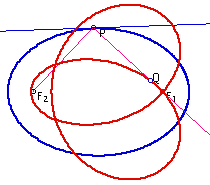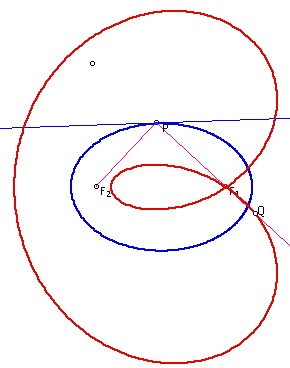Investigation 3. What happens if Q is on the normal line at the point Q (See Figure 7).

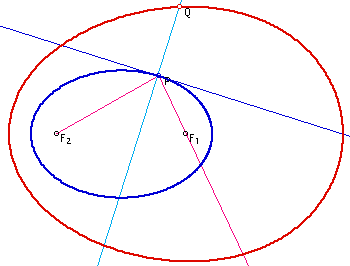Figure 7. GSP File