

![]() Check Problems from last lesson
Check Problems from last lesson
The word trigonometry comes from Greek words that mean "triangle measurement."
 When Egyptians first used a sundial
around 1500 B.C., they were using trigonometry.
When Egyptians first used a sundial
around 1500 B.C., they were using trigonometry.
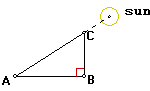 The picture to the
right is a model of how a sundial works:
The picture to the
right is a model of how a sundial works:
As the sun shines on the staff given by ![]() ,
it casts a shadow represented by
,
it casts a shadow represented by ![]() . The
staff is a fixed length, therefore the length of
. The
staff is a fixed length, therefore the length of ![]() varies with the measure of angle
varies with the measure of angle ![]() , which
changes as the sun moves throughout the day. The Egyptians looked
at the ratio
, which
changes as the sun moves throughout the day. The Egyptians looked
at the ratio ![]() to determine the time. This ratio
is called the tangent of
to determine the time. This ratio
is called the tangent of ![]() .
.
A ratio of the lengths of two sides of a right triangle is called a tigonometric ratio. The three most common ratios are sine, cosine, and tangent. They are abbreviated sin,cos, and tan respectively.
Trigonometric Ratios Definition:
refer to the triangle to the right:
Trogonometric ratios are related to the acute angles of a triangle, not the right angle. The value of the trigonometric ratio, depends only on the angle. Consider the following three similar triangles (why are they similar?):

By the definition of the sin above we have ![]() ,
,
![]() ,
,![]() .
.
But since the triangles are similar, the ratio of their sides
are equal, thus ![]() .
.
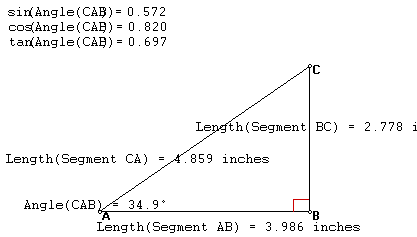 Activity:
Open the GSP Sketch and use Calculate
in the measure menu to calculate the sin,cos, and tangent of CAB
by computing the ratios in the definition above.
Activity:
Open the GSP Sketch and use Calculate
in the measure menu to calculate the sin,cos, and tangent of CAB
by computing the ratios in the definition above.
Compare to the calculated sin,cos, and tangent.
 Trigonometric ratios can be used
to find the measures of acute angles in a right triangle when
you know the measures of the two sides of the triangle. You must
determine which ratio to use depending on which sides are given.
Then, use the inverse trigonometric functions on your calculator
to find the angle.
Trigonometric ratios can be used
to find the measures of acute angles in a right triangle when
you know the measures of the two sides of the triangle. You must
determine which ratio to use depending on which sides are given.
Then, use the inverse trigonometric functions on your calculator
to find the angle.
Example: Find the approximate measure of
Find the approximate measure of
![]() E.
E.
We know the leg adjacent to ![]() E and
the measure of the hypotenuse. We would therefore use the cosine,
since it is defined in terms of the adjacent side and the
E and
the measure of the hypotenuse. We would therefore use the cosine,
since it is defined in terms of the adjacent side and the ![]() hypontenuse:
hypontenuse:
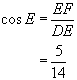
Now on your calculator, make sure it is in degree mode and enter:
5 ![]() 14 = INV COS
14 = INV COS
or on a TI-83 calculator: First hit mode key and select degree by using the arrows to put cursor over degree and pressing enter.
Now press 2nd COS 5 ![]() 14
) Enter
14
) Enter
You should get 69.075168. So, ![]() E
E![]() .
.