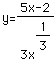. It may bring a question of multiplication
of two different functions. Say, students can think of 
and y=
. They will find out that drawing the graph of is not as easy
as they think. They will approach with its differentiated function 
. The next graphs are 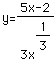and drawn by Algebra
Xpressor. The same type of problem happens again as we could see in Part-6.
Algebra can't draw the half of graph when x values are negative. But, students know that the graph will pass through (0,0) and (1,0).
But, one thing which is interesting is that the graph would not be symmetric.
Because we do not obtain the same y-values even if we put -x instead of
x. The differentiated function talks an important thing to students.
Since for any value of negative x the vlaues of y are always positive in
the differentiated 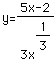function , so all the tangent line
should have positive slopes. More over, students can calculated some number
of negative x-values and their y-values in the function . Therefore students
will complete the graph of . The completed one is the following.
In fact, the left part of the graph was hand-made by using the drawing ability of Micro Soft Word. But, Students will have to have lots of information for the graph.