
Some Different Ways to Examine
ax2 + bx + c = 0
by
James W. Wilson and Kyungsoon Jeon
University of Georgia
It has now become a rather standard exercise with available technology to construct graphs to consider the equation
and to overlay several graphs of
for different values of a, b, or c as the other two are held constant. From these graphs discussion of the patterns for the roots of ax2 + bx + c = 0 can be followed.
For example, if we set y = x2 + bx + 1 for b = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture is obtained.

We can discuss the "movement" of a parabola as b is changed. The parabola always passes through the same point on the y-axis ( the point (0,1) with this equation). For b < -2 the parabola will intersect the x-axis in two points with positive x values (i.e. the original equation will have two real roots, both positive). For b = -2, the parabola is tangent to the x-axis and so the original equation has one real and positive root at the point of tangency. For -1 < b < 2, the parabola does not intersect the x-axis -- the original equation has no real roots. Similarly for b = 2 the parabola is tangent to the x-axis (one real negative root) and for b > 2, the parabola intersects the x-axis twice to show two negative real roots for each b. Consider again, the equation x2 + bx + 1 = 0.
Now graph this relation in the xb plane. We get the following graph.

If we take any particular value of b, say b = 3, and overlay this equation on the graph we add a line parallel to the x-axis. If it intersects the curve in the xb plane the intersection points correspond to the roots of the original equation for that value of b. We have the following graph.

For each value of b we select, we get a horizontal line. It is clear on a single graph that we get two negative real roots of the original equation when b > 2, one negative real root when b = 2, no real roots for -2 < b < 2, One positive real root when b = -2, and two positive real roots when b < -2.
Here, let's look at the roots. One of the most interesting things is that the two roots of an equation x2 + bx +1 = 0 are not different signs. The roots are all positive or all negative. So we have the following question.
Is there another way to estimate the characteristics of roots, their sum or their product, and their signs?
Let's consider y = x2 -8x +12 for an explicit understanding of the relationship between the coefficient of an equation and its roots. It is easy to know the roots of the equation x2 -8x +12 = 0, because it can be factored as ( x - 2 ) ( x - 6 ). We can check it in the graph of y = x2 -8x +12 .
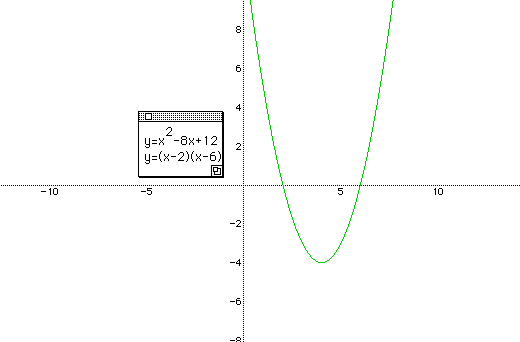
Just think about the sign of roots, the sum and product of the roots. It has two positive root, x = 2 and x = 6. What is the sum of two roots? It is 8. What is the product of two roots? It is 12.
Compare the values with the coefficients of the equation x2 -8x +12 = 0 . What can you find? The sum of two roots is the same with the negative coefficient of x of the equation, and the product of two roots is the same with the constant of the equation. Can you think that we can generalize this rule to every equation?
Let's think of another example, y = 3x2 + 6x -9. What is the roots of 3x2+6x -9 =0.

As the graph shows, the function intersect the x - axis in x = 1 and x = -3. What's the sum of two roots? It is -2. What is the product of two roots? It is - 3. Look at the coefficients of 3x2 + 6x -9 = 0.
It is different so that we can not apply the way from the previous example. If we follow the rule that we generalized in the former example, the sum should be -6, and the product should be -9.
Is there anything different that made these results? Yes, it is.
The function y = 3x2 + 6x -9 has a coefficient 3 in front of x2. If we divide the estimated numbers of the sum and the product by 3, then we get the right answers for the sum and product of two roots.
Let's make a generalization. For a given ax2 + bx + c = 0, the sum of two root of the equation is - b/a, and the product of two roots is c/a.
Here we need to compare the two graphs, y = 3x2 + 6x -9 and y = x2 + 2x -3.

As two graphs show, two function intersect the x - axis in the same place, but their shapes are different. They have different y - intercepts, different slope and so on. But we can know the sum and product of two roots just with two function and their coefficient.
Let's go back to the x2 + bx + 1 = 0 . As we talked about a horizontal line b for each value of b we select. How about the case of -2 < b < 2 ? . We now know that the equation has no real value.

But we can do the same calculation of getting the sum and product of two roots
without loss of generalization. For example, the sum is - 1 and the product is
1 for x2 + x + 1=0. If students know the determinant of the equation, ax2 + bx
+ c = 0, then we get D = b2 -4ac = 12 - 4* 1* 1 = -3 < 0. So it is certain
that the equation do not have real roots. But we can still extend our thinking
to the imaginary roots of an equation. The equation x2 + x + 1=0 has two roots
x=
 ,
and still the sum of two roots is -1 , and the product of two roots is 1.
,
and still the sum of two roots is -1 , and the product of two roots is 1.
For further study of the relationship between the coefficients of a function and the characteristics of roots of an equation, we can think of the next question. For a given equation x2 + 5x +c = c. If c = 1 , the x2 + 5x + 1 = 0 has two negative roots.

The sum of two roots is -5 and the product of two roots is 1. Here is another way of thinking according to the process we did so far. we are certain that the fact that the equation has two negative roots though we do not have the graph of y = x2 + 5x + 1. Because the product of two numbers is positive, 1, and the sum of two numbers is negative, -5. Now we check the graph.
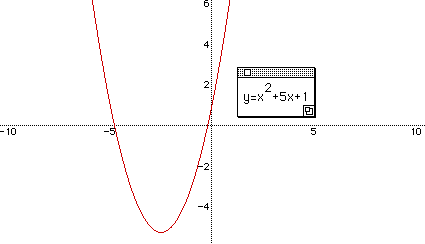
What we did just before is the reverse of usual process of thinking. Generally, we first graph a function and then find the roots or get some information of the function such as the sign of two roots, the sum and the product of two roots. But we can also the estimate the shape of a function with a given function and the relationship of the coefficients of a function.
It is real that algebraic understanding of an equation, a function, and exploring many characteristics of them has been posed in one of the most important curriculum in the secondary school education. I think that the combination of graphical software and algebraic understanding can accelerate the learning of mathematical concepts in a classroom.