
___________________________________________________________________________________________________________________________________________
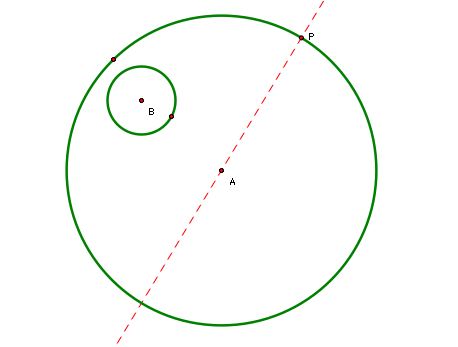
This assignment will explore the set of circles tangent to two given circles. First, let's begin with a construction of two given circles and a point, P, on the larger given circle:


We will investigate two scenarios to demonstrate the tangent circles where 1) the smaller given circle is on the exterior of the desired tangent circle, and 2) the smaller given circle is on the interior of the desired tangent circle. The above images show a diameter of the larger given circle. Next, let's construct an identical circle to the smaller given circle with center at point P:
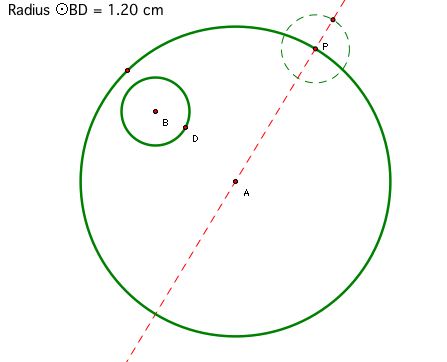
We used the known radius BD and center point, P, to construct these green dashed circles above. Notice the new points on this circle with center P -- one point is on the exterior of the larger given circle and the other is on the interior of the larger given circle.
Next, let's construct segment BL and segment BM with their respective midpoints. Then, construct a perpendicular bisector:
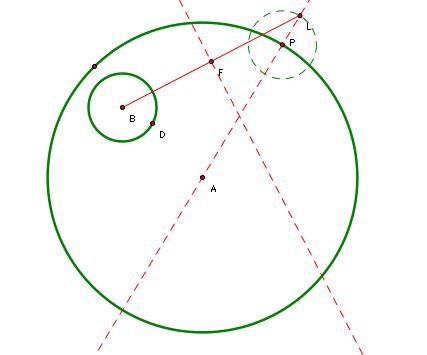

Now, we can observe the respective isosceles triangles with a vertex at the intersection of the larger given circle's diameter and the newly constructed perpendicular bisector -- X and Y, respectively. Also, we can construct the respective tangent circles of the two given circles by defining the radius from center (i.e. X and Y, respectively) to the point P:
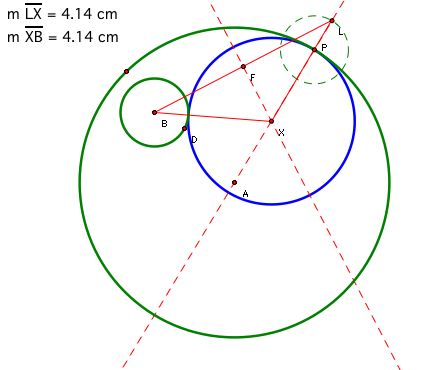
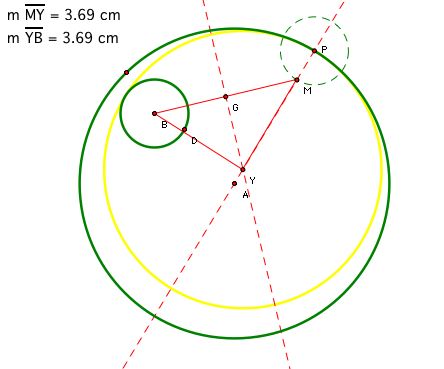
For future reference, one can use these GSP Script Tools to construct the above tangent circles -- Blue Tangent Circle and Yellow Tangent Circle. Also, one can animate these tangent circles to observe how they change as P moves around the larger given circle.
__________________________________________________________________________________________________________________________________________
Now, suppose we trace the perpendicular lines to segment BL and segment BM, respectively, as the point P moves along the larger given circle. To see this animation for Blue Tangent Circle, click here. To see this animation for Yellow Tangent Circle, click here (GSP 4.06 software required to view the links). Otherwise, observe the results below:

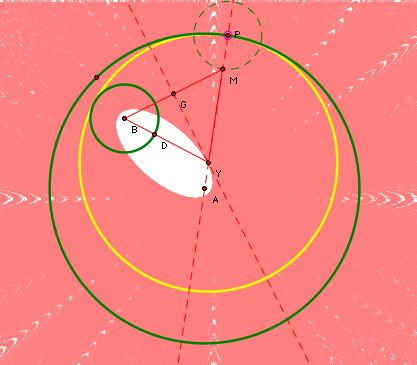
What do you observe? Exactly, an ellipse with foci at A and B! How does each ellipse differ? Well, one observation is that the tangency of the smaller given circle and the tangent circle remains in the interior of the ellipse on the left, but this tangency occurs on the exterior of the ellipse on the right.
__________________________________________________________________________________________________________________________________________
Likewise, interesting observations occur when the given circles overlap and when they are outside of each other (i.e. do not touch and are not in the interior of the other)...
First, let's look at the tangent circle construction when our given circles overlap:
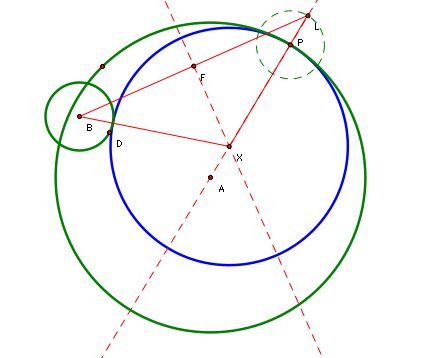
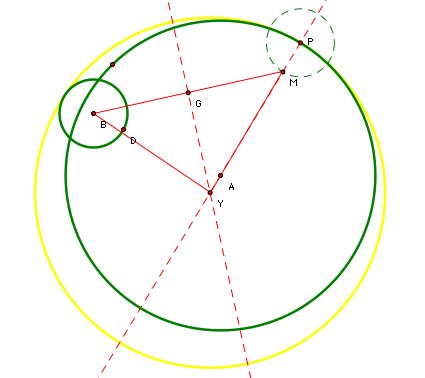
Suppose we trace the center of the tangent circles as the point P moves around the larger given circle. What is the resulting image?
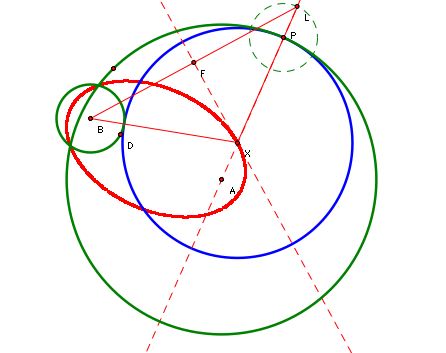

...an ellipse and a hyperbola!
Now, suppose the two given circles do not intersect. What happens when we trace the center of the tangent circles as the point P moves around the larger given circle in this scenario?
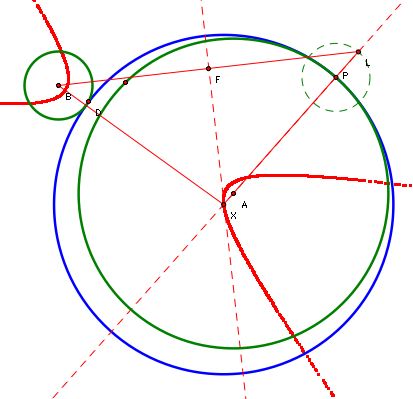
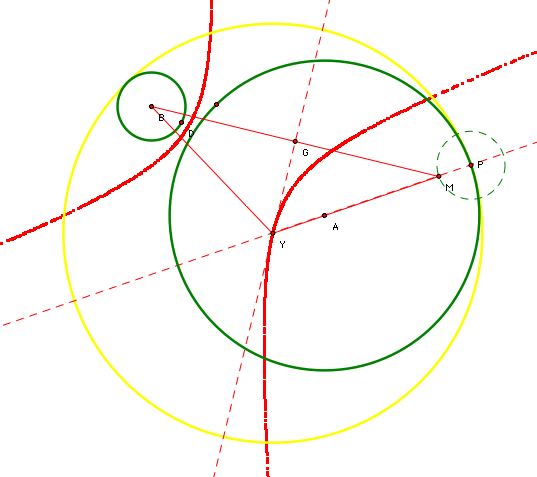
...two hyperbolas!
__________________________________________________________________________________________________________________________________________