

A. Consider any triangle ABC. Select a point P inside the triangle and draw lines AP, BP, and CP extended to their intersections with the opposite sides in points D, E, and F respectively.
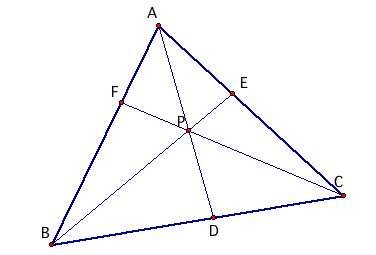
Explore (AF)(BD)(EC) and (FB)(DC)(EA) for various triangles and various locations of P.
B. Conjecture? Prove it! (you may need draw some parallel lines to produce some similar triangles) Also, it probably helps to consider the ratio

Can the result be generalized (using lines rather than segments to construct ABC) so that point P can be outside the triangle? Show a working GSP sketch.
C. Show that when P is inside triangle ABC, the ratio of the areas of triangle ABC and triangle DEF is always greater than or equal to 4. When is it equal to 4?
Parts A & B: To begin, let's consider (AF)(BD)(EC) and (FB)(DC)(EA) for various positions of P inside and outside the triangle ABC.
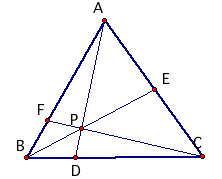
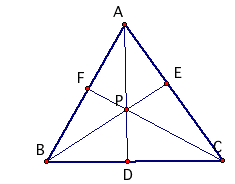

Now, in each of the above instances, (AF)(BD)(EC)=(FB)(DC)(EA)! Let's try to prove this. We will consider two cases, the first when P is inside triangle ABC and second when P is outside triangle ABC.
Case 1: P inside triangle ABC
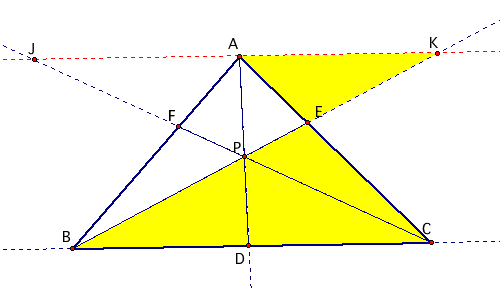
Consider any given triangle ABC with point P on the interior. Then consider the lines extended from the vertices A, B, and C to their respective opposite sides through P to D, E, and F respectively. Now, through triangle similarity, we can show that (AF)(BD)(EC)/(FB)(DC)(EA)=1.
To begin, let's extend the line CF and BE. Next, we will construct line JK through A which is parallel to BC.
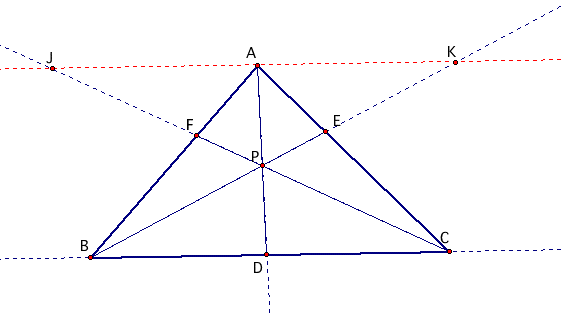
Then, angle AEK = angle BEC since they are opposite angles (or vertical angles) and angle AKE = angle EBC since they are alternate interior angles. Then, angle EAK = angle ECB since they are alternate interior angles (and by transitivity and sums of interior angles). Therefore, triangle AEK is similar to triangle CDB. To visualize the similar triangles, see the image below.
Now similar to above, angle JFA = angle BFC since they are opposite angles and angle FJA = angle FCB since they are alternate interior angles. Then, angle FAJ = angle FBC since they are alternate interior angles (and by transitivity and sums of interior angles). Therefore, triangle AFJ is similar to BFC. To 'see' the similar triangles, please see the image below.

Similar to above, angle JPA = angle CPD since they are opposite angles and angle PJA = angle PCD since they are alternate interior angles. Then, angle PAJ = angle PDC since they are alternate interior angles (and by transitivity and sums of interior angles). Therefore, triangle PAJ is similar to PDC. To 'see' the similar triangles, please see the image below.
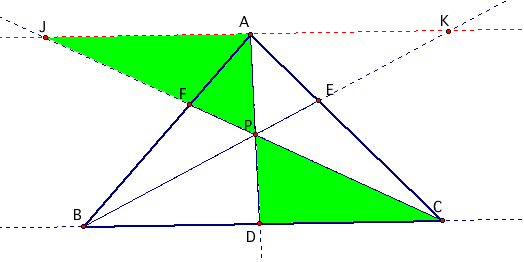
Similar to above, angle APK = angle DPB since they are opposite angles and angle PKA = angle PBD since they are alternate interior angles. Then, angle PDB = angle {AK since they are alternate interior angles (and by transitivity and sums of interior angles). Therefore, triangle PAK is similar to PKB. To 'see' the similar triangles, please see the image below.

Now, using the four pairs of similar triangles we have the following ratios:
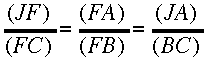
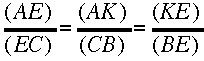

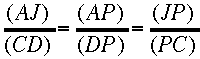
Using these ratios we can say
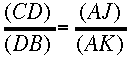
Then, we can say
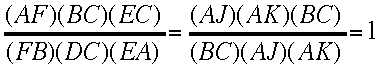
And our proof is complete.
Case 2: P is outside triangle ABC.
Consider any given triangle ABC with point P on the interior. Then consider the lines extended from the vertices A, B, and C to their respective opposite sides through P to D, E, and F respectively. Now, through triangle similarity, we can show that (AF)(BD)(EC)/(FB)(DC)(EA)=1.
To begin, let's extend the line CF and BE. Next, we will construct line JK through A which is parallel to BC.
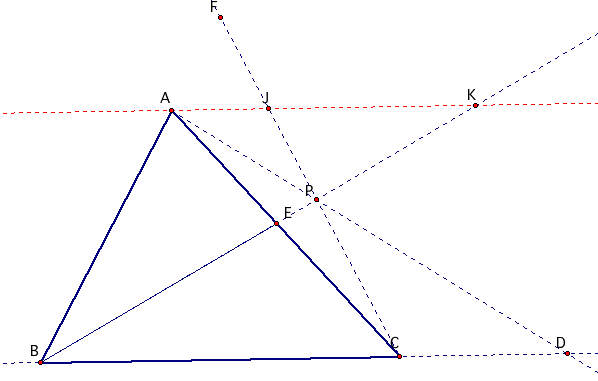
Similar to our actions in Case 1, we have the same four pairs of congruent triangles as when P is inside triangle ABC. Then, following exactly above, it holds that
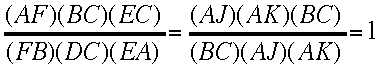
when P is outside triangle ABC.
Now, moving on to part C.
We can see through exploration that the ratio of triangle ABC : triangle DEF is always greater than or equal to 4. To view point P's movement in a GSP please download the GSP file and use the action buttons.

Now, to answer when the ratio is equal to four, recall that in assignment 4 we defined the medial triangle to have 1/4 the area of it's larger 'host' triangle.
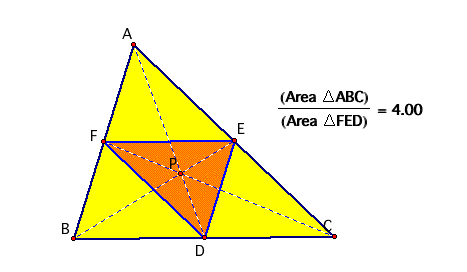
With that, the part C is complete!