

There are multipe ways to state the definition of the limit of function, but if you do not understand the mathematical jargon, what good is the definition anyways? In lieu of beginning with mathematical definition, let's begin with three explorations.
Exploration 1 (Zeno's Paradox): John has decided that he is remarkably hungry and is craving Taco Bell. John decides to walk to Taco Bell, but decides to travel is a most precourioius way, John will begin by traveling only half way from his house to Taco Bell in his first hour of walking. Within the second hour, John will travel half the remaining distrance from his house to Taco Bell, or one quarter of the distance between his house and Taco Bell. During the third hour, John will travel one half the remaining distance, or said another way, one eighth of the distance from his house to Taco Bell.
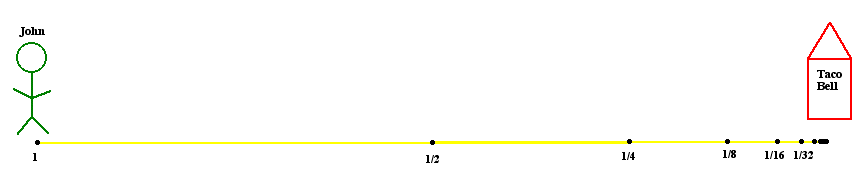
Now our question is, what happens to John in the "long run"? Does John ever reach Taco Bell? If so, when?
Exploration 2 (Fibonacci Numbers): The Fibonacci sequence is the set of numbers 1, 1, 2, 3, 5, 8, 13, 21, 34, ... where the next number in the sequence is the sum of the previous two (i.e. 21+34=55, so 55 would be the next number in the sequence). Look at the ratios of pairs of consecutive numbers, for example
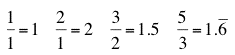
Compute some additional ratios. What happens in the "long run"? Does the sequence of ratios tend to appraoch a specific value, or in other words, does the sequence of ratios approach a limit?
Exploration 3 (e function): Consider the function
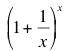
Is zero in the domain of the function? What happens as this function as x gets closer and closer to zero? In other words, is there a limit, or "long run" value that this function approaches as x gets close to zero? Begin by exploring this question with your calculator or mathematics software. Compare the graph and table values when you zoom in very close.
Now that you have completed some explorations of limit, you should have generated your own idea and concept of what a limit is. To take this concept further we will explore one problem in depth, but first, let us formally state the definition of limit.
limit: We say "the limit of f(x), as x approaches a, equals L"when we write
In other words, if we can make the values of f(x) arbitrarily close to L (as close to L as we want to go) by taking values sufficiently close to a, but not equal to a.
There are also left-hand limits and right-hand limits, i.e.
We say "the left-hand limit of f(x) as x approaches a for the limit of f(x) as x approaches a from the left is equal to L" if we can make the values of f(x) as close to L as we like by taking x sufficiently close to a and x less than a. For the right-hand limit we say "the right-hand limit of f(x) as x approaches a for the limit of f(x) as x approaches a from the right is equal to L" if we can make the values of f(x) as close to L as we like by taking x sufficiently close to a and x greater than a (Stewart 1998).
Directly from our above definitions, we know the following statement is true:
Now, we will need to use these above definitions to complete the following problem:
Consider the function f whose graph is shown below.
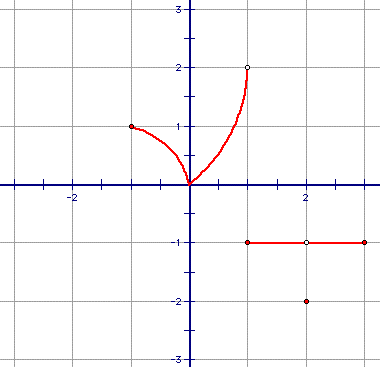
Indicate whether the following statements below are true or false. If a statement is false, correct it so that it is true.
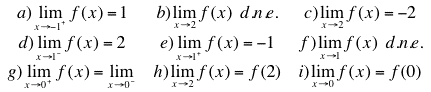
In you would like further practice with limits, complete the following problem.
Compute ![]() and
and ![]() for
the following functions.
for
the following functions.
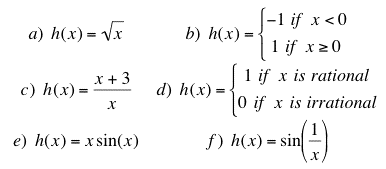
This activity was adapted from a Math Excel course taught at Portland State University, spring term 2004 by Joe Ediger and Erin Horst.
Stewart, J. (1998) Calculus: Concepts and contexts. Pacific Grove, CA: Brooks/Cole Publishing Co.