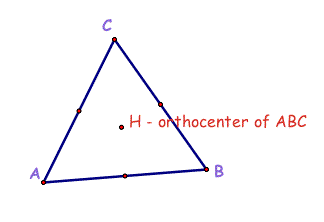
Start by constructing any triangle ABC, including the midpoints. Include the Orthocenter, H.

Using the Orthocenter we can divide triangle ABC into 3 smaller triangles. See below.
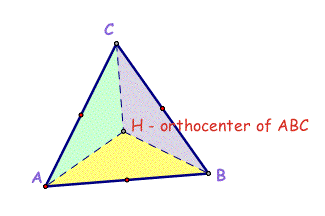
We now have 4 triangles to study and compare. The original being triangle ABC and the other 3 triangles formed using H are: AHC, CHB, and AHB.
The question: How are these 3 triangles formed by using the Orthocenter similar? different?
MORE ORTHOCENTERS:
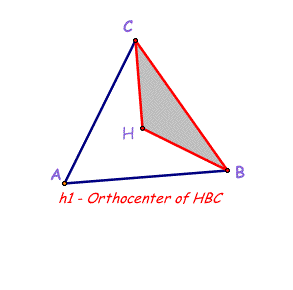
Find the Orthocenter of triangle HBC (shaded gray) - labeled h1 and happens to be located on vertex point A of the original triangle ABC.
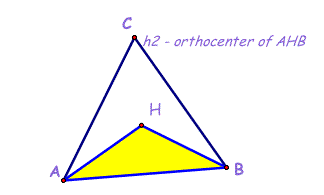
Find the Orthocenter of triangle AHB (shaded yellow) - labeled h2 and happens to be located on the vertex point C of the original triangle ABC.
Find the Orthocenter of triangle HAC (shaded green) - labeled h3 and happens to be located on the vertex point B of the original triangle ABC.
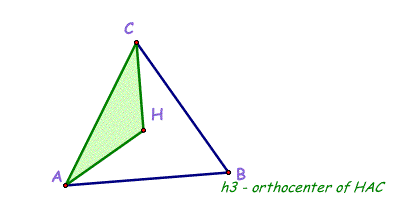
Thus, each of the 3 triangles formed by using H, the Orthocenter, of the original triangle ABC, has their Orthocenter located on a vertex point (A, B or C).
How else can we compare these 3 triangles?
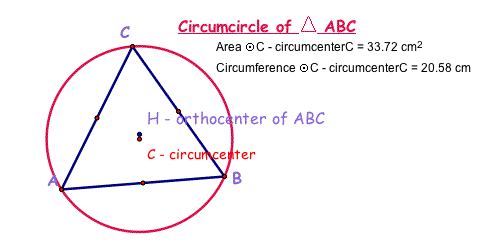
The circumcircle for triangle ABC is shown below, as well as H, the Orthocenter, and the Circumcenter of triangle ABC.
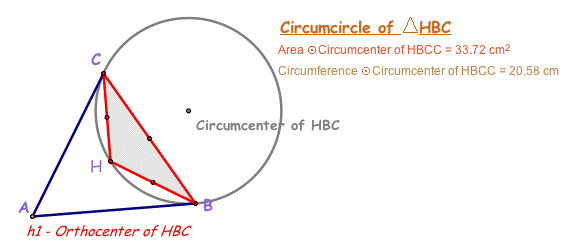
The circumcircle for triangle HBC is shown below along with the Circumcenter of triangle HBC.
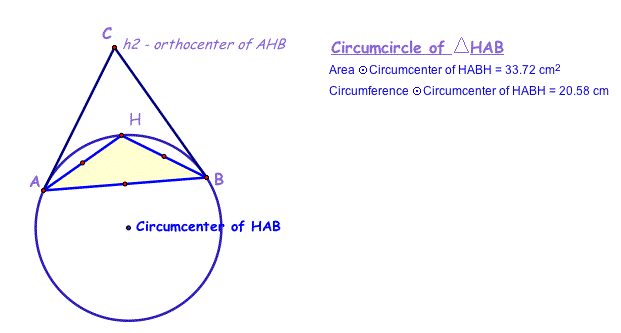
The circumcircle for triangle HAB is shown below along with the Circumcenter of triangle HAB.
The circumcircle for triangle HAC is shown below along with the Circumcenter of triangle HAC.
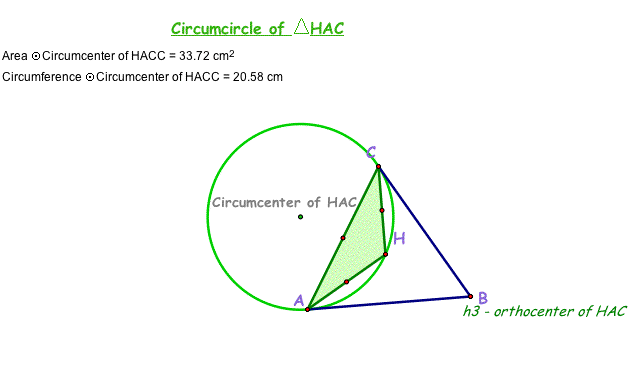
Note that the areas and the circumferences of all four of these circumcircles are equal -
Area = 33.72 square cm. and Circumference = 20.58 cm for all of them.
Thus all four of the triangles are similar.
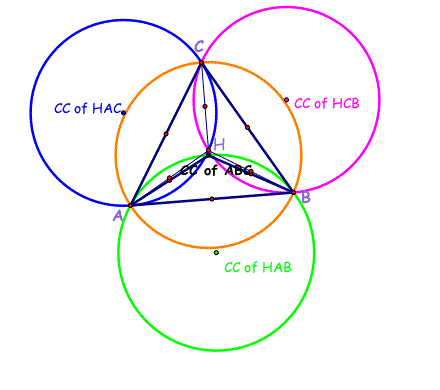
Looking at the BIG PICTURE.
We can look at all 4 of the circumcircles overlaid onto triangle ABC. Note the intersection points of these 4 circumcircles: 3 circles intersect at each of the vertex points of triangle ABC.
For instance, CC of HAC and CC of HCB intersect with CC of ABC at vertex point C.
CC of HCB and CC of HAB intersect CC of ABC at vertex point B.
CC of HAB and CC of HAC intersect CC of ABC at vertex point A.