by Soo Jin Lee
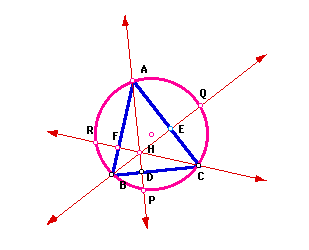
11. Construct any acute triangle ABC and its circumcircle. Construct the three altitudes AD, BE, and CF. Extend each altitude to its intersection with the circumcircle at corresponding points P, Q, and R.
Find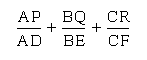 
.
and prove your result.
I constructed picture as below and interesting result have come out.
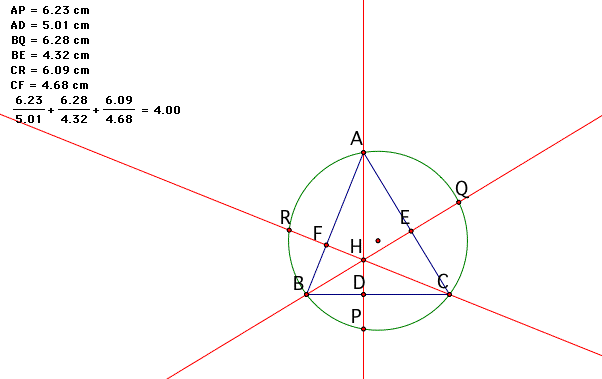
is always "4"
whenever I move my vertexes around.
you can experience this by clicking here.
the proof for this result is..
since DP = HD, HE = EQ and HF = FR
AP/AD + BQ/BE + CR/CF
= (AD + HD)/AD + (BE + HE)/BE + (CF + HF)/CF
= 1 + HD/AD + 1 + HE/BE + 1 + HF/CF
= 3 + (HD/AD + HE/BE + HF/CF)
If I can prove that that (HD/AD + HE/BE + HF/CF) = 1,
= 4 is established.
the area of the triangle ABC
= AD * BC * (1/2) ------------------1)
= AB * CF * (1/2) -------------------2)
= AC * BE * (1/2)--------------------3)
= area of triangle ABH + area of BCH + area of CAH
= (HF * AB * 1/2) + (BC * HD * 1/2) + (AC * EH * 1/2)-----------4)
by 1) = 4)
AD * BC * (1/2) = (HF * AB * 1/2) + (BC * HD * 1/2) + (AC * EH * 1/2)
dividing by both sides by AD * BC * (1/2),
1 = (HF * AB)/(AD * BC) + (BC * HD)/(AD * BC) + (AC* EH )/(AD * BC)----5)
since 1) = 2) = 3),
AD * BC = AB * CF = AC * BE
then we can substitute those into the equation 5) and we will get the below equation,
1 = (HF * AB)/(AB * CF) + (BC * HD)/(AD * BC) + (AC* EH )/(AC * BE )
by eliminating same factor, we will get
HF/CF + HD/AD + EH/BE = 1
so now I can prove
AP/AD + BQ/BE + CR/CF
= 3 + (HD/AD + HE/BE + HF/CF)
= 3 + 1
= 4