By Soo Jin Lee and Jaehong Shin
Day 2
Last time we saw some patterns to draw the answer for
(1/4) + (1/4)^2 + (1/4)^3 + (1/4)^4 +... + (1/4)^n+...------------*
the answer of * is 1/3.
Why??
Well, we already looked at some patterns to find the conclusion.
If you want to look back on your patterns, click here to see the Day 1
By doing continuous job, you will finally find the below picture which is telling you the answer is (1/3).
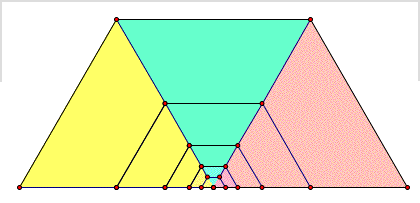
Let's investigate some other examples!
Exploration1
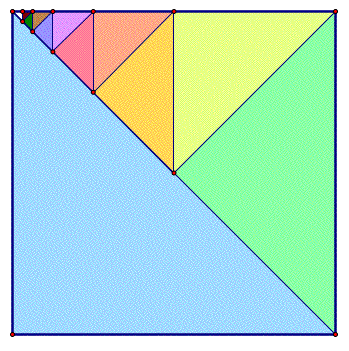
1. Take a piece of square grid paper. outline a 32 * 32 square. Divide the square in half by drawing a diagonal.
Color in half the square. Now divide the uncolored triangle into two congruent triangles and color in one of those triangles.
Again, divide the uncolored triangle in half, and color in one, Think of doing this infinitely many times.
Would you ever have to start coloring outside the original square? If not, it has a finite sum.
2. What do you get for the infinite sum of (1/2)
i.e (1/2) + (1/2)^2 + (1/2)^3 + (1/2)^4 +... + (1/2)^n+...=?
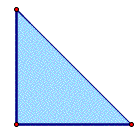-1/2 
---(1/2)^2
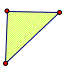--(1/2)^3 .............
Do you recognize the infinite sum of (1/2) is "1"!!!!
Notice the two series that we have observed so far.
(1/4) + (1/4)^2 + (1/4)^3 + ... + (1/4)^n + ... = 1/3
(1/2) + (1/2)^2 + (1/2)^3 + ... + (1/2)^n + ... = 1/1 = 1
That makes me wonder if
(1/3) + (1/3)^2 + (1/3)^3 +... + (1/3)^n + ... = 1/2
It seems like true, but how can we show that?
think about this as your homework.